Advertisements
Advertisements
Question
Check whether 7 + 3x is a factor of 3x3 + 7x.
Solution 1
7 + 3x will be a factor of 3x3 + 7x only if 7 + 3x divides 3x3 + 7x leaving no remainder.
Let p(x) = 3x3 + 7x
7 + 3x = 0
⇒ 3x = -7
`⇒ x = -7/3`
`therefore"Remainder "= 3(-7/3)^3+7(-7/3)`
`= -343/9-49/3`
`= -490/9`
`≠ 0`
∴ 7 + 3x is not a factor of 3x3 + 7x.
Solution 2
Let us divide (3x3 + 7x) by (7 + 3x). If the remainder obtained is 0, then 7 + 3x will be a factor of 3x3 + 7x.
By long division,
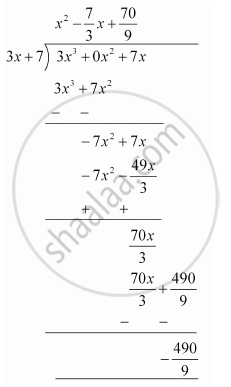
As the remainder is not zero, therefore, 7 + 3x is not a factor of 3x3 + 7x.
APPEARS IN
RELATED QUESTIONS
Find the value of a, if the division of ax3 + 9x2 + 4x – 10 by x + 3 leaves a remainder 5.
What number should be subtracted from x3 + 3x2 – 8x + 14 so that on dividing it by x – 2, the remainder is 10?
Using the Remainder Theorem, factorise the following completely:
3x3 + 2x2 – 23x – 30
Use remainder theorem and find the remainder when the polynomial g(x) = x3 + x2 – 2x + 1 is divided by x – 3.
If p(x) = 4x3 - 3x2 + 2x - 4 find the remainderwhen p(x) is divided by:
x + 2
Using remainder theorem, find the value of a if the division of x3 + 5x2 – ax + 6 by (x – 1) leaves the remainder 2a.
Find ‘a’ if the two polynomials ax3 + 3x2 – 9 and 2x3 + 4x + a, leaves the same remainder when divided by x + 3.
When 2x3 – 9x2 + 10x – p is divided by (x + 1), the remainder is – 24.Find the value of p.
What is the remainder when x2018 + 2018 is divided by x – 1
If x51 + 51 is divided by x + 1, the remainder is ______.
