Advertisements
Advertisements
Question
Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial
t2 – 3, 2t4 + 3t3 – 2t2 – 9t – 12
Solution
t2 - 3, 2t4 + 3t3 - 2t2 - 9t - 12
t2 - 3 = t2 + 0.t - 3
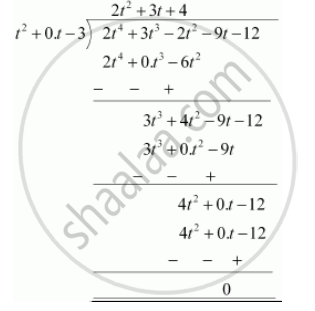
Since the remainder is 0,
Hence, t2 - 3 is a factor of 2t4 + 3t3 - 2t2 - 9t - 12
APPEARS IN
RELATED QUESTIONS
Divide the polynomial p(x) by the polynomial g(x) and find the quotient and remainder in each of the following
p(x) = x3 – 3x2 + 5x – 3, g(x) = x2 – 2
Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial
x3 – 3x + 1, x5 – 4x3 + x2 + 3x + 1
On dividing x3 – 3x2 + x + 2 by a polynomial g(x), the quotient and remainder were x – 2 and –2x + 4, respectively. Find g(x)
Give examples of polynomials p(x), g(x), q(x) and r(x), which satisfy the division algorithm
deg r(x) = 0
If the polynomial x4 – 6x3 + 16x2 – 25x + 10 is divided by another polynomial x2 – 2x + k, the remainder comes out to be x + a, find k and a.
Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in the following f(x) = x3 − 6x2 + 11x − 6, g(x) = x2 + x + 1
Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in the following f(x) = 15x3 − 20x2 + 13x − 12; g(x) = x2 − 2x + 2
Check whether the first polynomial is a factor of the second polynomial by applying the division algorithm g(x) = 2x2 − x + 3, f(x) = 6x5 − x4 + 4x3 − 5x2 − x − 15
The sum of (x + 5) observations is (x3 + 125). Find the mean of the observations
For which values of a and b, are the zeroes of q(x) = x3 + 2x2 + a also the zeroes of the polynomial p(x) = x5 – x4 – 4x3 + 3x2 + 3x + b? Which zeroes of p(x) are not the zeroes of q(x)?
