RD Sharma solutions for Mathematics [English] Class 10 chapter 2 - Polynomials [Latest edition]

Find the zeroes of the following quadratic polynomial and verify the relationship between the zeroes and the coefficients:

x² – 2x – 8

Find the zeroes of the following quadratic polynomial and verify the relationship between the zeroes and the coefficients:

4s² – 4s + 1

Find the zeroes of the following quadratic polynomial and verify the relationship between the zeroes and the coefficients:

t² – 15

Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients

`p(x) = x^2 + 2sqrt2x + 6`

Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients

`q(x)=sqrt3x^2+10x+7sqrt3`

Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients

`f(x)=x^2-(sqrt3+1)x+sqrt3`

Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients

`g(x)=a(x^2+1)-x(a^2+1)`

Find the zeroes of the following quadratic polynomial and verify the relationship between the zeroes and the coefficients.

6x² – 3 – 7x

If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate α - β

If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate `1/alpha-1/beta`

If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate `1/alpha+1/beta-2alphabeta`

If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate α²β + αβ²

If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate α⁴ + β⁴ 

If α and β are the zeroes of the quadratic polynomial f(x) = ax² + bx + c, then evaluate `1/(aalpha+b)+1/(abeta+b)`.

If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate `beta/(aalpha+b)+alpha/(abeta+b)`

If α and β are the zeros of the quadratic polynomial f(x) = ax² + bx + c, then evaluate :

`a(α^2/β+β^2/α)+b(α/β+β/α)`

If α and β are the zeros of the quadratic polynomial f(x) = 6x² + x − 2, find the value of `alpha/beta+beta/alpha`.

If a and are the zeros of the quadratic polynomial f(x) = 𝑥² − 𝑥 − 4, find the value of `1/alpha+1/beta-alphabeta`

If 𝛼 and 𝛽 are the zeros of the quadratic polynomial p(x) = 4x² − 5x −1, find the value of α^2β + αβ².

If a and 3 are the zeros of the quadratic polynomial f(x) = x² + x − 2, find the value of `1/alpha-1/beta`.

If 𝛼 and 𝛽 are the zeros of the quadratic polynomial f(x) = x² − 5x + 4, find the value of `1/alpha+1/beta-2alphabeta`

If 𝛼 and 𝛽 are the zeros of the quadratic polynomial f(t) = t² − 4t + 3, find the value of `alpha^4beta^3+alpha^3beta^4`

If α and β are the zeros of the quadratic polynomial p(y) = 5y² − 7y + 1, find the value of `1/alpha+1/beta`

If α and β are the zeros of the quadratic polynomial p(s) = 3s² − 6s + 4, find the value of `alpha/beta+beta/alpha+2[1/alpha+1/beta]+3alphabeta`

If α and β are the zeros of the quadratic polynomial f(x) = x² − px + q, prove that `alpha^2/beta^2+beta^2/alpha^2=p^4/q^2-(4p^2)/q+2`

If the squared difference of the zeros of the quadratic polynomial f(x) = x² + px + 45 is equal to 144, find the value of p.

If the sum of the zeros of the quadratic polynomial f(t) = kt² + 2t + 3k is equal to their product, find the value of k.

If one zero of the quadratic polynomial f(x) = 4x² − 8kx − 9 is negative of the other, find the value of k.

If α and β are the zeros of the quadratic polynomial f(x) = x² − 1, find a quadratic polynomial whose zeroes are `(2alpha)/beta" and "(2beta)/alpha`

If α and β are the zeros of the quadratic polynomial f(x) = x² − 3x − 2, find a quadratic polynomial whose zeroes are `1/(2alpha+beta)+1/(2beta+alpha)`

If α and β are the zeros of a quadratic polynomial such that α + β = 24 and α − β = 8, find a quadratic polynomial having α and β as its zeros.

If α and β are the zeros of the quadratic polynomial f(x) = x² − p (x + 1) — c, show that (α + 1)(β +1) = 1− c.

If If α and β are the zeros of the quadratic polynomial f(x) = x² – 2x + 3, find a polynomial whose roots are α + 2, β + 2.

If If α and β are the zeros of the quadratic polynomial f(x) = x² – 2x + 3, find a polynomial whose roots are `(alpha-1)/(alpha+1)` , `(beta-1)/(beta+1)`

If α and β are the zeroes of the polynomial f(x) = x² + px + q, form a polynomial whose zeroes are (α + β)² and (α − β)².

Verify that the numbers given along side of the cubic polynomials are their zeroes. Also verify the relationship between the zeroes and the coefficients.

`2x^3 + x^2 – 5x + 2 ; 1/2, 1, – 2`

Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also verify the relationship between the zeroes and the coefficients in each case

x³ – 4x² + 5x – 2; 2, 1, 1

Find a cubic polynomial with the sum, sum of the product of its zeroes taken two at a time, and product of its zeros as 3, −1 and −3 respectively.

If the zeros of the polynomial f(x) = 2x³ − 15x² + 37x − 30 are in A.P., find them.

Find the condition that the zeros of the polynomial f(x) = x³ + 3px² + 3qx + r may be in A.P.

If the zeros of the polynomial f(x) = ax³ + 3bx² + 3cx + d are in A.P., prove that 2b³ − 3abc + a²d = 0.

If the zeros of the polynomial f(x) = x³ − 12x² + 39x + k are in A.P., find the value of k.

Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in the following f(x) = x³ − 6x² + 11x − 6, g(x) = x² + x + 1

Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in the following f(x) = 10x⁴ + 17x³ − 62x² + 30x − 3, g(x) = 2x² + 7x + 1

Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in the following f(x) = 4x³ + 8x² + 8x + 7, g(x) = 2x² − x + 1

Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in the following f(x) = 15x³ − 20x² + 13x − 12; g(x) = x² − 2x + 2

Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial

t² – 3, 2t⁴ + 3t³ – 2t² – 9t – 12

Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial

x³ – 3x + 1, x⁵ – 4x³ + x² + 3x + 1

Check whether the first polynomial is a factor of the second polynomial by applying the division algorithm g(x) = 2x² − x + 3, f(x) = 6x⁵ − x⁴ + 4x³ − 5x² − x − 15

Obtain all zeros of f(x) = x³ + 13x² + 32x + 20, if one of its zeros is −2.

Obtain all zeros of the polynomial f(x) = x⁴ − 3x³ − x² + 9x − 6, if two of its zeros are `-sqrt3` and `sqrt3`

Find all zeros of the polynomial f(x) = 2x⁴ − 2x³ − 7x² + 3x + 6, if its two zeros are `-sqrt(3/2)` and `sqrt(3/2)`

What must be added to the polynomial f(x) = x⁴ + 2x³ − 2x² + x − 1 so that the resulting polynomial is exactly divisible by x² + 2x − 3 ?

What must be subtracted from the polynomial f(x) = x⁴ + 2x³ − 13x² − 12x + 21 so that the resulting polynomial is exactly divisible by x² − 4x + 3 ?

Find all the zeros of the polynomial x⁴ + x³ − 34x² − 4x + 120, if two of its zeros are 2 and −2.

Find all zeros of the polynomial 2x⁴ + 7x³ − 19x² − 14x + 30, if two of its zeros are `sqrt2` and `-sqrt2`.

Find all the zeros of the polynomial 2x³ + x² − 6x − 3, if two of its zeros are `-sqrt3` and `sqrt3`

Find all the zeros of the polynomial x³ + 3x² − 2x − 6, if two of its zeros are `-sqrt2` and `sqrt2`

The sum and product of the zeros of a quadratic polynomial are \[- \frac{1}{2}\] and −3 respectively. What is the quadratic polynomial.

Write the family of quadratic polynomials having \[- \frac{1}{4}\] and 1 as its zeros.

In Fig. 2.17, the graph of a polynomial p(x) is given. Find the zeros of the polynomial.

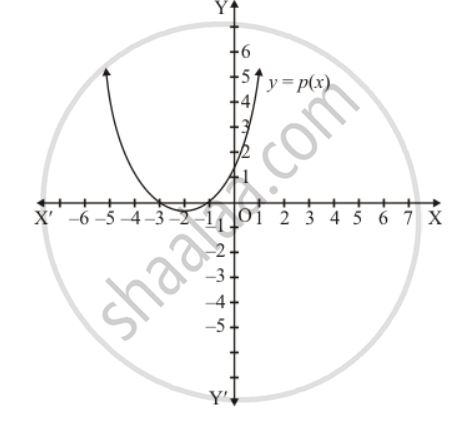
The graph of a polynomial y = f(x), shown in Fig. 2.18. Find the number of real zeros of f(x).

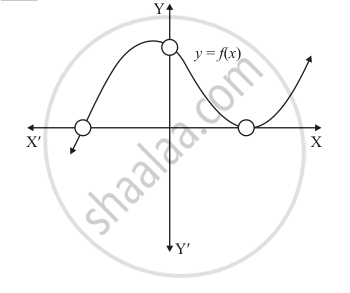
The graph of the polynomial f(x) = ax² + bx + c is as shown below (Fig. 2.19). Write the signs of 'a' and b² − 4ac.

The graph of the polynomial f(x) = ax² + bx + c is as shown in Fig. 2.20. Write the value of b² − 4ac and the number of real zeros of f(x).

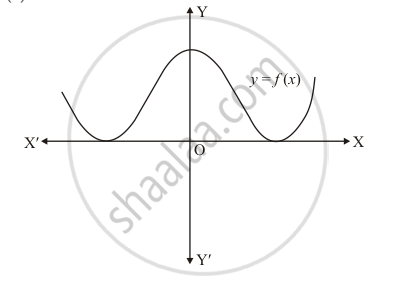
The graph of a polynomial f(x) is as shown in Fig. 2.21. Write the number of real zeros of f(x).

Write a quadratic polynomial, sum of whose zeros is \[2\sqrt{3}\] and their product is 2.

If α, β are the zeros of the polynomial f(x) = x² + x + 1, then \[\frac{1}{\alpha} + \frac{1}{\beta} =\]

If α, β are the zeros of the polynomial p(x) = 4x² + 3x + 7, then \[\frac{1}{\alpha} + \frac{1}{\beta}\]  is equal to

If one zero of the polynomial f(x) = (k² + 4)x² + 13x + 4k is reciprocal of the other, then k=

If the sum of the zeros of the polynomial f(x) = 2x³ − 3kx² + 4x − 5 is 6, then the value ofk is

If α and β are the zeros of the polynomial f(x) = x² + px + q, then a polynomial having \[\frac{1}{\alpha} \text{and}\frac{1}{\beta}\]  is its zero is 

If α, β are the zeros of polynomial f(x) = x² − p (x + 1) − c, then (α + 1) (β + 1) =

If α, β are the zeros of the polynomial f(x) = x² − p(x + 1) − c such that (α +1) (β + 1) = 0, then c =

If f(x) = ax² + bx + c has no real zeros and a + b + c = 0, then 

If the diagram in Fig. 2.22 shows the graph of the polynomial f(x) = ax² + bx + c, then

Figure 2.23 show the graph of the polynomial f(x) = ax² + bx + c for which 

If the product of zeros of the polynomial f(x) ax³ − 6x² + 11x − 6 is 4, then a =

If zeros of the polynomial f(x) = x³ − 3px² + qx − r are in A.P., then

If the product of two zeros of the polynomial f(x) = 2x³ + 6x² − 4x + 9 is 3, then its third zero is

If the polynomial f(x) = ax³ + bx − c is divisible  by the polynomial g(x) = x² + bx + c, then ab =

If Q.No. 14, c =

If one root of the polynomial f(x) = 5x² + 13x + k is reciprocal of the other, then the value of k is

If α, β, γ are the zeros of the polynomial f(x) = ax³ + bx² + cx + d, the\[\frac{1}{\alpha} + \frac{1}{\beta} + \frac{1}{\gamma} =\]

If α, β, γ are the zeros of the polynomial f(x) = ax³ + bx² + cx + d, then α² + β² + γ² =

If α, β, γ are are the zeros of the polynomial f(x) = x³ − px² + qx − r, the\[\frac{1}{\alpha\beta} + \frac{1}{\beta\gamma} + \frac{1}{\gamma\alpha} =\]

If α, β are the zeros of the polynomial f(x) = ax² + bx + c, then\[\frac{1}{\alpha^2} + \frac{1}{\beta^2} =\]

If two of the zeros of the cubic polynomial ax³ + bx² + cx + d are each equal to zero, then the third zero is

If two zeros x³ + x² − 5x − 5 are \[\sqrt{5}\ \text{and} - \sqrt{5}\], then its third zero is

The product of the zeros of x³ + 4x² + x − 6 is

What should be added to the polynomial x² − 5x + 4, so that 3 is the zero of the resulting polynomial?

What should be subtracted to the polynomial x² − 16x + 30, so that 15 is the zero of the resulting polynomial?

A quadratic polynomial, the sum of whose zeroes is 0 and one zero is 3, is

If two zeroes of the polynomial x³ + x² − 9x − 9 are 3 and −3, then its third zero is

If \[\sqrt{5}\ \text{and} - \sqrt{5}\]   are two zeroes of the polynomial x³ + 3x² − 5x − 15, then its third zero is

If x + 2 is a factor of x² + ax + 2b and a + b = 4, then

The polynomial which when divided by −x² + x − 1 gives a quotient x − 2 and remainder 3, is