Advertisements
Advertisements
Question
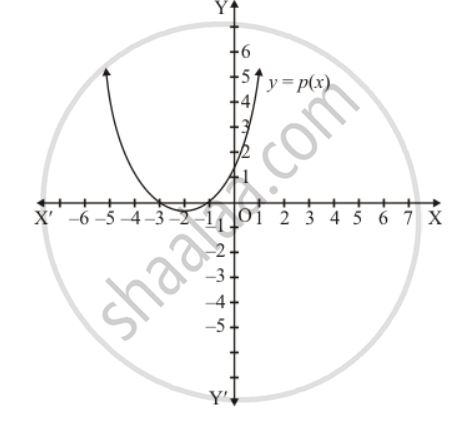
In Fig. 2.17, the graph of a polynomial p(x) is given. Find the zeros of the polynomial.
Solution
Just see the point of intersection of the curve and x-axis and find out the x-coordinate of these points. These x-coordinates will be the zeros of the polynomial Since the intersection points are
`(-3,0)` and `(-1,0)`

Hence, the zeros of the polynomial is -3 and -1
APPEARS IN
RELATED QUESTIONS
Classify the following polynomials as linear, quadratic, cubic and biquadratic polynomials:
`3y`
Classify the following polynomials as polynomials in one-variable, two variables etc:
`t^3_3t^2+4t-5`
The sum and product of the zeros of a quadratic polynomial are \[- \frac{1}{2}\] and −3 respectively. What is the quadratic polynomial.
The graph of the polynomial f(x) = ax2 + bx + c is as shown in Fig. 2.20. Write the value of b2 − 4ac and the number of real zeros of f(x).

If the product of two zeros of the polynomial f(x) = 2x3 + 6x2 − 4x + 9 is 3, then its third zero is
State whether the given algebraic expression is polynomial? Justify.
10
Case Study -1

The figure given alongside shows the path of a diver, when she takes a jump from the diving board. Clearly it is a parabola.
Annie was standing on a diving board, 48 feet above the water level. She took a dive into the pool. Her height (in feet) above the water level at any time‘t’ in seconds is given by the polynomial h(t) such that h(t) = -16t2 + 8t + k.
Rita’s height (in feet) above the water level is given by another polynomial p(t) with zeroes -1 and 2. Then p(t) is given by ______.
`sqrt(2)` is a polynomial of degree ______.
The degree of the sum of two polynomials each of degree 5 is always 5.
Classify the following as a constant, linear, quadratic and cubic polynomials:
1 + x + x2
