Advertisements
Advertisements
Question
If f(x) is a polynomial such that f(a) f(b) < 0, then what is the number of zeros lying between a and b?
Solution
If f (x) is a polynomial such that `f(a) f (b) < 0` then this means the value of the polynomial are of different sign for a to b
Hence, at least one zero will be lying between a and b
APPEARS IN
RELATED QUESTIONS
State division algorithm for polynomials.
If f(x) = x3 + x2 − ax + b is divisible by x2 − x write the value of a and b.
If one zero of the polynomial f(x) = (k2 + 4)x2 + 13x + 4k is reciprocal of the other, then k=
If zeros of the polynomial f(x) = x3 − 3px2 + qx − r are in A.P., then
Divide. Write the quotient and the remainder.
(21x4 − 14x2 + 7x) ÷ 7x3
Divide. Write the quotient and the remainder.
(6x5 − 4x4 + 8x3 + 2x2) ÷ 2x2
An asana is a body posture, originally and still a general term for a sitting meditation pose, and later extended in hatha yoga and modern yoga as exercise, to any type of pose or position, adding reclining, standing, inverted, twisting, and balancing poses. In the figure, one can observe that poses can be related to representation of quadratic polynomial.


The graph of parabola opens downwards, if:
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.

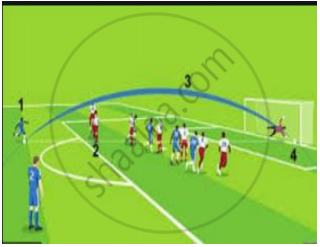
The three zeroes in the below shown graph are:
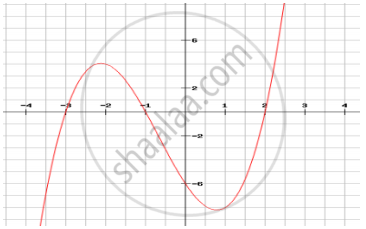
Determine the degree of the following polynomial:
2x – 1
Determine the degree of the following polynomial:
y3(1 – y4)
