Advertisements
Online Mock Tests
Chapters
2: Polynomials
3: Pair of Linear Equations in Two Variables
4: Quadratic Equations
5: Arithmetic Progression
6: Co-Ordinate Geometry
7: Triangles
8: Circles
9: Constructions
10: Trigonometric Ratios
11: Trigonometric Identities
12: Trigonometry
13: Areas Related to Circles
14: Surface Areas and Volumes
15: Statistics
▶ 16: Probability
![RD Sharma solutions for Mathematics [English] Class 10 chapter 16 - Probability RD Sharma solutions for Mathematics [English] Class 10 chapter 16 - Probability - Shaalaa.com](/images/8193647920-mathematics-english-class-10_6:5809898a5fef45e9a2f7e6b414d392fa.jpg)
Advertisements
Solutions for Chapter 16: Probability
Below listed, you can find solutions for Chapter 16 of CBSE RD Sharma for Mathematics [English] Class 10.
RD Sharma solutions for Mathematics [English] Class 10 16 Probability Exercise 16.1 [Pages 20 - 26]
The probability that it will rain tomorrow is 0.85. What is the probability that it will not rain tomorrow?
A die is thrown. Find the probability of getting 2 or 4
A die is thrown. Find the probability of getting a multiple of 2 or 3
A die is thrown. Find the probability of getting:
an even prime number
A die is thrown. Find the probability of getting a number greater than 5
A die is thrown once. Find the probability of getting a number lying between 2 and 6.
Three different coins are tossed together. Find the probability of getting exactly two heads.
Three different coins are tossed together. Find the probability of getting at least two heads.
Three coins are tossed together. Find the probability of getting at least one head and one tail
Three coins are tossed together. Find the probability of getting no tails
A and B throw a pair of dice. If A throws 9, find B’s chance of throwing a higher number.
Two unbiased dice are thrown. Find the probability that the total of the numbers on the dice
is greater than 10.
A card is drawn at random from a well-shuffled deck of playing cards. Find the probability that the card drawn is a black king.
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is either a black card or a king
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is black and a king
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is a jack, queen or a king
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is neither a heart nor a king
A card is drawn at random from a well-shuffled deck of playing cards. Find the probability that the card drawn is a card of spade or an ace.
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is neither an ace nor a king
A card is drawn at random from a well shuffled pack of 52 playing cards. Find the probability of getting neither a red card nor a queen.
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is other than an ace
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is a ten
One card is drawn from a well-shuffled deck of 52 cards. Find the probability of getting a spade.
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is a black card
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is the seven of clubs
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is jack
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is the ace of spades
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is a queen
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is a heart
A card is drawn at random from a pack of 52 cards. Find the probability that card drawn is a red card
In a lottery of 50 tickets numbered 1 to 50, one ticket is drawn. Find the probability that the
drawn ticket bears a prime number.
An urn contains 10 red and 8 white balls. One ball is drawn at random. Find the probability
that the ball drawn is white.
A bag contains 3 red balls, 5 black balls and 4 white balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is Red
A bag contains 3 red balls, 5 black balls and 4 white balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is Black
A bag contains 3 red balls, 5 black balls and 4 white balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is Not red
What is the probability that a number selected from the numbers 1, 2, 3, ..., 15 is a multiple
of 4?
A bag contains 5 white and 7 red balls. One ball is drawn at random. What is the probability that ball drawn is not black?
A bag contains 6 red, 8 black and 4 white balls. A ball is drawn at random. What is the probability that ball drawn is not black?
Tickets numbered from 1 to 20 are mixed up and a ticket is drawn at random. What is the probability that the ticket drawn has a number which is a multiple of 3 or 7?
In a lottery there are 10 prizes and 25 blanks. What is the probability of getting a prize?
A bag contains 5 white and 7 red balls. One ball is drawn at random. What is the
probability that ball drawn is white?
If the probability of winning a game is 0.3, what is the probability of losing it?
A bag contains 5 black, 7 red and 3 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is Red
A bag contains 5 black, 7 red and 3 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is black or white
Cards numbered 1 to 30 are put in a bag. A card is drawn at random from this bag. Find the probability that the number on the drawn card is not a perfect square number
A bag contains 5 black, 7 red and 3 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is not black
A bag contains 4 red, 5 black and 6 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is White
A bag contains 4 red, 5 black and 6 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is Red
A bag contains 4 red, 5 black and 6 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is Not black
A bag contains 4 red, 5 black and 6 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is Red or white
One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a king of red suit
One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a face card
One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a red face card
One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a queen of black suit
One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a jack of hearts
One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a spade
Five cards, the ten, jack, queen, king and ace of diamonds, are well-shuffled with their face downwards. One card is then picked up at random. What is the probability that the card is the queen?
Five cards—ten, jack, queen, king, and an ace of diamonds are shuffled face downwards.One card is picked at random If a king is drawn first and put aside, what is the probability that the second card picked up is the ace?
A bag contains 3 red balls and 5 black balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is red?
A bag contains 3 red balls and 5 black balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is Black
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? that the sum of the two numbers that turn up is 8?
A game of chance consists of spinning an arrow which is equally likely to come to rest pointing to one of the number, 1, 2, 3, ..., 12 as shown in Fig. below. What is the probability that it will point to 10?
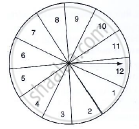
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? of obtaining a total of 6?
A game of chance consists of spinning an arrow which is equally likely to come to rest pointing to one of the number, 1, 2, 3, ..., 12 as shown in Fig. below. What is the probability that it will point to an odd number?

A game of chance consists of spinning an arrow which is equally likely to come to rest
pointing to one of the number, 1, 2, 3, ..., 12 as shown in Fig. below. What is the
probability that it will point to a number which is multiple of 3?
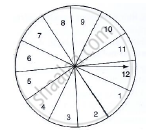
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability of obtaining a total of 10?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? of obtaining the same number on both dice?
A game of chance consists of spinning an arrow which is equally likely to come to rest
pointing to one of the number, 1, 2, 3, ..., 12 as shown in Fig. below. What is the
probability that it will point to an even number?
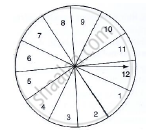
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability of obtaining a total more than 9?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability that the sum of the two numbers appearing on the top of the dice is 13?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability that the sum of the numbers appearing on the top of the dice is less than or equal to 12?
In a class, there are 18 girls and 16 boys. The class teacher wants to choose one pupil form class monitor. What she does, she writes the name of each pupil on a card and puts them into a basket and mixes thoroughly. A child is asked to pick one card from the basket. What is the probability that the name written on the card is the name of a girl?
In a class, there are 18 girls and 16 boys. The class teacher wants to choose one pupil for class monitor. What she does, she writes the name of each pupil on a card and puts them into a basket and mixes thoroughly. A child is asked to pick one card from the basket. What is the probability that the name written on the card is the name of a boy?
Why is tossing a coin considered to be a fair way of deciding which team should choose ends in a game of cricket?
What is the probability that a number selected at random from the number 1,2,2,3,3,3, 4, 4, 4, 4 will be their average?
There are 30 cards, of same size, in a bag on which numbers 1 to 30 are written. One card is taken out of the bag at random. Find the probability that the number on the selected card is not divisible by 3.
A bag contains cards which are numbered from 2 to 90. A card is drawn at random from the bag. Find the probability that it bears a two digit number
A bag contains cards which are numbered from 2 to 90. A card is drawn at random from the bag. Find the probability that it bears a number which is a perfect square
A bag contains 5 red, 8 white and 7 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is red or white
A bag contains 5 red, 8 white and 7 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is not black
A bag contains 5 red, 8 white and 7 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is neither white nor black.
Find the probability that a number selected from the number 1 to 25 is not a prime number when each of the given numbers is equally likely to be selected.
Two customers are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another. What is the robability that both will visit the shop on the same day?
Two customers are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another. What is the probability that both will visit the shop on different days?
Two customers are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another. What is the probability that both will visit the shop on consecutive days?
A bag contains 8 red, 6 white and 4 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is Red or white
A bag contains 8 red, 6 white and 4 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is Not black
A bag contains 8 red, 6 white and 4 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is Neither white nor black
Find the probability that a number selected at random from the numbers 1, 2, 3, ..., 35 is a Prime number
Find the probability that a number selected at random from the numbers 1, 2, 3, ..., 35 is a Multiple of 7
Find the probability that a number selected at random from the numbers 1, 2, 3, ..., 35 is a Multiple of 3 or 5
From a pack of 52 playing cards Jacks, queens, kings and aces of red colour are removed. From the remaining, a card is drawn at random. Find the probability that the card drawn is A black queen
From a pack of 52 playing cards Jacks, queens, kings and aces of red colour are removed. From the remaining, a card is drawn at random. Find the probability that the card drawn is A red card
From a pack of 52 playing cards Jacks, queens, kings and aces of red colour are removed. From the remaining, a card is drawn at random. Find the probability that the card drawn is A black jack
From a pack of 52 playing cards Jacks, queens, kings and aces of red colour are removed. From the remaining, a card is drawn at random. Find the probability that the card drawn is a picture card (Jacks, queens and kings are picture cards)
The faces of a red cube and a yellow cube are numbered from 1 to 6. Both cubes are rolled. What is the probability that the top face of each cube will have the same number?
A bag contains lemon flavoured candies only. Malini takes out one candy without looking into the bag. What is the probability that she takes out an orange flavoured candy?
A bag contains lemon flavoured candies only. Malini takes out one candy without looking into the bag. What is the probability that she takes out a lemon flavoured candy?
The probability of selecting a green marble at random from a jar that contains only green, white and yellow marbles is 1/4 The probability of selecting a white marble at random from the same jar is 1/3 If this jar contains 10 yellow marbles. What is the total number of marbles in the jar?
It is given that m a group of 3 students, the probability of 2 students not having the same birthday is 0.992. What is the probability that the 2 students have the same birthday?
A bag contains 3 red balls and 5 black balls. A ball is draw at random from the bag. What is the probability that the ball drawn is red?
A bag contains 3 red balls and 5 black balls. A ball is draw at random from the bag. What is the probability that the ball drawn is not red?
A box contains 5 red marbles, 8 white marbles, and 4 green marbles, One marble is taken out of the box at random. What is the probability that the marble taken out will be red?
A box contains 5 red marbels, 8 white marbles and 4 green marbles, One marble is taken out of the box at ramdom. What is the probability that the marble taken out will be white?
A box contains 5 red marbels, 8 white marbles and 4 green marbles, One marble is taken out of the box at ramdom. What is the probability that the marble taken out will be not green?
A lot consists of 144 ball pens of which 20 are defective and the others are good. Nuri will buy a pen if it is good, but will not buy if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that she will buy it?
A lot consists of 144 ball pens, of which 20 are defective and the others are good. Nuri will buy a pen if it is good, but will not buy if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that she will not buy it?
12 defective pens are accidently mixed with 132 good ones. It is not possible to just look at pen and tell whether or not it is defective. one pen is taken out at random from this lot. Determine the probability that the pen taken out is good one.
Five cards − the ten, jack, queen, king and ace of diamonds, are well-shuffled with their face downwards. One card is then picked up at random.
What is the probability that the card is the queen?
Five cards − the ten, jack, queen, king and ace of diamonds, are well-shuffled with their face downwards. One card is then picked up at random.
If the queen is drawn and put a side, what is the probability that the second card picked up is (a) an ace? (b) a queen?
Harpreet tosses two different coins simultaneously (say, one is of Re 1 and other of Rs 2). What is the probability that he gets at least one head?
Cards marked with numbers 13, 14, 15, ...., 60 are placed in a box and mixed thoroughly.One card is drawn at random from the box. Find the probability that number on the card drawn is divisible by 5
Cards marked with numbers 13, 14, 15, ...., 60 are placed in a box and mixed thoroughly.One card is drawn at random from the box. Find the probability that number on the card drawn is a number is a perfect square
A bag contains tickets numbered 11, 12, 13,..., 30. A ticket is taken out from the bag at random. Find the probability that the number on the drawn ticket is a multiple of 7
A bag contains tickets numbered 11, 12, 13,..., 30. A ticket is taken out from the bag at random. Find the probability that the number on the drawn ticket is greater than 15 and a multiple of 5.
Fill in blank:
Probability of a sure event is...........
Fill in blank:
Probability of an impossible event is...........
Fill in blank:
The probability of an event (other than sure and impossible event) lies between……
Fill in blank:
Every elementary event associated to a random experiment has........... probability.
Fill in blank:
Probability of an event A + Probability of event ‘not A’ ...........
Fill in blank:
Sum of the probabilities of each outcome m an experiment is ..........
Examine the following statement and comment:
If two coins are tossed at the same time, there are 3 possible outcomes—two heads,
two tails, or one of each. Therefore, for each outcome, the probability of occurrence
is 1/3
Examine the following statement and comment:
If a die is thrown once, there are two possible outcomes—an odd number or an
even number. Therefore, the probability of obtaining an odd number is 1 /2 and the
probability of obtaining an even number is 1/2
.
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears a two-digit number.
A box contains loo red cards, 200 yellow cards and 50 blue cards. If a card is drawn at random from the box, then find the probability that it will be a blue card
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears a perfect square number.
A box contains loo red cards, 200 yellow cards and 50 blue cards. If a card is drawn at random from the box, then find the probability that it will be not a yellow card
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears a number divisible by 5.
A box contains loo red cards, 200 yellow cards and 50 blue cards. If a card is drawn at random from the box, then find the probability that it will be neither yellow nor a blue card.
A box contains cards numbered 3, 5, 7, 9, ..., 35, 37. A card is drawn at random form the box. Find the probability that the number on the drawn card is a prime number.
A group consists of 12 persons, of which 3 are extremely patient, other 6 are extremely honest and rest are extremely kind. A person form the group is selected at random. Assuming that each person is equally likely to be selected, find the probability of selecting a person who is extremely patient
A group consists of 12 persons, of which 3 are extremely patient, other 6 are extremely honest and rest are extremely kind. A person form the group is selected at random. Assuming that each person is equally likely to be selected, find the probability of selecting a person who is extremely kind or honest. Which of the above you prefer more.
Cards numbered 1 to 30 are put in a bag. A card is drawn at random from this bag. Find the probability that the number on the drawn card is not divisible by 3
Cards numbered 1 to 30 are put in a bag. A card is drawn at random from this bag. Find the probability that the number on the drawn card is a prime number greater than 7
A piggy bank contains hundred 50 paise coins, fifity ₹1 coins, twenty ₹2 coins and ten ₹5 coins. If it is equally likely that one of the coins will fall out when the bank is turned upside down, find the probability that the coin which fell will be a 50 paise coin
A piggy bank contains hundred 50 paise coins, fifity ₹1 coins, twenty ₹2 coins and ten ₹5 coins. If it is equally likely that one of the coins will fall out when the bank is turned upside down, find the probability that the coin which fell will be of value more than ₹1
A piggy bank contains hundred 50 paise coins, fifity ₹1 coins, twenty ₹2 coins and ten ₹5 coins. If it is equally likely that one of the coins will fall out when the bank is turned upside down, find the probability that the coin which fell will be of value less than ₹5
A piggy bank contains hundred 50 paise coins, fifity ₹1 coins, twenty ₹2 coins and ten ₹5 coins. If it is equally likely that one of the coins will fall out when the bank is turned upside down, find the probability that the coin which fell will be a ₹1 or ₹2 coin
A bag contains cards numbered from 1 to 49. A card is drawn from the bag at random, after mixing the card thoroughly. Find the probability that the number on the drawn card is an odd number
A bag contains cards numbered from 1 to 49. A card is drawn from the bag at random, after mixing the card thoroughly. Find the probability that the number on the drawn card is a multiple of 5
A bag contains cards numbered from 1 to 49. A card is drawn from the bag at random, after mixing the card thoroughly. Find the probability that the number on the drawn card is a perfect square
A bag contains cards numbered from 1 to 49. A card is drawn from the bag at random, after mixing the card thoroughly. Find the probability that the number on the drawn card is an even prime number
A box contains 20 cards numbered from 1 to 20. A card is drawn at random from the box. Find the probability that the number on the drawn card is divisible by 2 or 3
A box contains 20 cards numbered from 1 to 20. A card is drawn at random from the box. Find the probability that the number on the drawn card is a prime number
In a simultaneous throw of a pair of dice, find the probability of getting 8 as the sum
In a simultaneous throw of a pair of dice, find the probability of getting a doublet
In a simultaneous throw of a pair of dice, find the probability of getting a doublet of prime numbers
In a simultaneous throw of a pair of dice, find the probability of getting a doublet of odd numbers
In a simultaneous throw of a pair of dice, find the probability of getting a sum greater than 9
In a simultaneous throw of a pair of dice, find the probability of getting an even number on first
In a simultaneous throw of a pair of dice, find the probability of getting an even number on one and a multiple of 3 on the other
In a simultaneous throw of a pair of dice, find the probability of getting neither 9 nor 1 1 as the sum of the numbers on the faces
In a simultaneous throw of a pair of dice, find the probability of getting a sum less than 6
Two different dice are thrown together. Find the probability that the numbers obtained have a sum less than 7
In a simultaneous throw of a pair of dice, find the probability of getting a sum more than 7
In a simultaneous throw of a pair of dice, find the probability of getting 1 at least once
In a simultaneous throw of a pair of dice, find the probability of getting a number other than 5 on any dice.
What is the probability that an ordinary year has 53 Sundays?
What is the probability that a leap year has 53 Sundays and 53 Mondays?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? that the sum of the two numbers that turn up is 8?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? of obtaining a total of 6?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability of obtaining a total of 10?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? of obtaining the same number on both dice?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability of obtaining a total more than 9?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability that the sum of the two numbers appearing on the top of the dice is 13?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability that the sum of the numbers appearing on the top of the dice is less than or equal to 12?
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability? that the product of numbers appearing on the top of the dice is less than 9.
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability?
that the difference of the numbers appearing on the top of two dice is 2.
A black die and a white die are thrown at the same time. Write all the possible outcomes. What is the probability?
that the numbers obtained have a product less then 16.
A bag contains cards which are numbered from 2 to 90. A card is drawn at random from the bag. Find the probability that it bears a two digit number
A bag contains cards which are numbered from 2 to 90. A card is drawn at random from the bag. Find the probability that it bears a number which is a perfect square
The faces of a red cube and a yellow cube are numbered from 1 to 6. Both cubes are rolled. What is the probability that the top face of each cube will have the same number?
The probability of selecting a green marble at random from a jar that contains only green, white and yellow marbles is 1/4 The probability of selecting a white marble at random from the same jar is 1/3 If this jar contains 10 yellow marbles. What is the total number of marbles in the jar?
- A lot of 20 bulbs contain 4 defective ones. One bulb is drawn at random from the lot. What is the probability that this bulb is defective?
- Suppose the bulb drawn in (1) is not defective and is not replaced. Now one bulb is drawn at random from the rest. What is the probability that this bulb is not defective?
A number is selected at random from first 50 natural numbers. Find the probability that it is a multiple of 3 and 4.
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears a two-digit number.
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears a perfect square number.
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears a number divisible by 5.
Two dice, one blue and one grey, are thrown at the same time.
(i) Write down all the possible outcomes and complete the following table:
| Event : ‘Sum on 2 dice’ |
2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| Probability | `1/36` | `5/36` | `1/36` |
A bag contains 6 red balls and some blue balls. If the probability of drawing a blue ball the bag is twice that of a red ball, find the number of blue balls in the bag.
The king, queen and jack of clubs are removed from a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the
probability of getting a card of heart
The king, queen and jack of clubs are removed from a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the
probability of getting a card of queen
The king, queen and jack of clubs are removed from a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the
probability of getting a card of clubs.
The king, queen and jack of clubs are removed form a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the probability of getting a card of a face card
The king, queen and jack of clubs are removed form a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the probability of getting a card of a queen of diamond.
Two dice are thrown simultaneously. What is the probability that 5 will not come up on either of them?
Two dice are thrown simultaneously. What is the probability that 5 will come up on at least one?
Two dice are thrown simultaneously. What is the probability that 5 wifi come up at both dice?
A number is selected at random from first 50 natural numbers. Find the probability that it is a multiple of 3 and 4.
A dice is rolled twice. Find the probability that 5 will not come up either time
A dice is rolled twice. Find the probability that 5 will come up exactly one time
All the black face cards are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is drawn at random. Find the probability of getting face card
All the black face cards are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is drawn at random. Find the probability of getting red card
All the black face cards are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is drawn at random. Find the probability of getting black card
All the black face cards are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is drawn at random. Find the probability of getting king
Cards numbered from 11 to 60 are kept in a box. If a card is drawn at random from the box, find the probability that the number on the drawn card is an odd number
Cards numbered from 11 to 60 are kept in a box. If a card is drawn at random from the box, find the probability that the number on the drawn card is a perfect square number
Cards numbered from 11 to 60 are kept in a box. If a card is drawn at random from the box, find the probability that the number on the drawn card is divisible by 5
Cards numbered from 11 to 60 are kept in a box. If a card is drawn at random from the box, find the probability that the number on the drawn card isa prime number less than 20
All kings and queens are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is randomly drawn from it. Find the probability that this card is a red face card
All kings and queens are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is randomly drawn from it. Find the probability that this card is a black card.
All jacks, queens and kings are removed from a pack of 52 cards. The remaining cards are well-shuffled and then a card is randomly drawn from it. Find the probability that this card is a black face card
All jacks, queens and kings are removed from a pack of 52 cards. The remaining cards are well-shuffled and then a card is randomly drawn from it. Find the probability that this card is a red card.
Red queens and black jacks are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the card drawn is a king
Red queens and black jacks are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the card drawn is of red colour
Red queens and black jacks are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the card drawn is a face card
Red queens and black jacks are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the card drawn is a queen
All red face cards are removed from a pack of playing cards. The remaining cards are well shuffled and then a card is drawn at random from them. Find the probability that the drawn card is a red card
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of square
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of green colour
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of green colour
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of green square.
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of blue circle and
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of green
All red face cards are removed from a pack of playing cards. The remaining cards are well shuffled and then a card is drawn at random from them. Find the probability that the drawn card is a red card
All red face cards are removed from a pack of playing cards. The remaining cards are well shuffled and then a card is drawn at random from them. Find the probability that the drawn card is a face card and
All red face cards are removed from a pack of playing cards. The remaining cards are well shuffled and then a card is drawn at random from them. Find the probability that the drawn card is a card of clubs.
Two customers are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another. What is the robability that both will visit the shop on the same day?
Two customers are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another. What is the probability that both will visit the shop on different days?
Two customers are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another. What is the probability that both will visit the shop on consecutive days?
RD Sharma solutions for Mathematics [English] Class 10 16 Probability Exercise 16.2 [Page 33]
Suppose you drop a tie at random on the rectangular region shown in the given figure. What is the probability that it will land inside the circle with diameter 1 m?

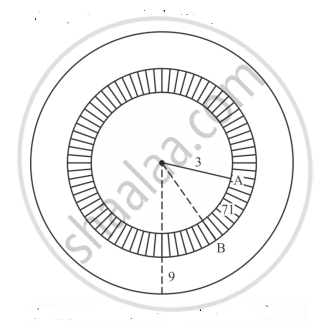
In the accompanying diagram a fair spinner is placed at the center O of the circle.
Diameter AOB and radius OC divide the circle into three regions labelled X, Y and Z. If
∠BOC = 45°. What is the probability that the spinner will land in the region X?(See fig)

A target shown in Fig. below consists of three concentric circles of radii, 3, 7 and 9 cm
respectively. A dart is thrown and lands on the target. What is the probability that the dart will land on the shaded region?
In below Fig., points A, B, C and D are the centers of four circles that each have a radius of length one unit. If a point is selected at random from the interior of square ABCD. What is the probability that the point will be chosen from the shaded region?

In the Fig. below, JKLM is a square with sides of length 6 units. Points A and B are the
mid- points of sides KL and LM respectively. If a point is selected at random from the
interior of the square. What is the probability that the point will be chosen from the interior of ΔJAB?
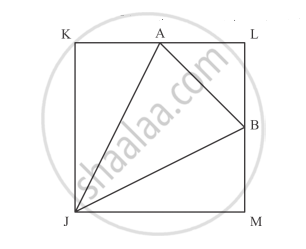
In the given figure, a square dart board is shown. The length of a side of the larger square is 1.5 times the length of a side of the smaller square. If a dart is thrown and lands on the larger square. What is the probability that it will land in the interior of the smaller square?
RD Sharma solutions for Mathematics [English] Class 10 16 Probability Exercise 16.3 [Pages 34 - 35]
Cards each marked with one of the numbers 4, 5, 6, ..., 20 are placed in a box and mixed thoroughly. One card is drawn at random from the box. What is the probability of getting an even number?
One card is drawn from a well shuffled deck of 52 playing cards. What is the probability of getting a non-face card?
A bag contains 5 red, 8 green and 7 white balls, One ball is drawn at random from the bag. What is the probability of getting a white ball or a green ball?
A die is thrown once. What is the probability of getting a prime number?
A die thrown once. What is the probability of getting a number lying between 2 and 6?
A die is thrown once. What is the probability of getting an odd number?
If \[\bar{E}\] denote the complement or negation of an even E, what is the value of P(E) + P(\[\bar{E}\]) ?
One card is drawn at random from a well shuffled deck of 52 cards. What is the probability of getting an ace?
Two coins are tossed simultaneously. What is the probability of getting at least one head?
Tickets numbered 1 to 20 are mixed up and then a ticket is drawn at random. What is the probability that the ticket drawn bears a number which is a multiple of 3?
From a well shuffled pack of cards, a card is drawn at random. Find the probability of getting a black queen.
A die is thrown once. Find the probability of getting a number less than 3.
Two coins are tossed simultaneously. Find the probability of getting exactly one head.
A die is thrown once. What is the probability of getting a number greater than 4?
What is the probability that a number selected at random from the numbers 3, 4, 5, ....9 is a multiple of 4?
What is the probability that a number selected at random from the numbers 3, 4, 5, ....9 is a multiple of 4?
A letter of English alphabet is chosen at random. Determine the probability that the chosen letter is a consonant.
A bag contains 3 red and 5 black balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is not red.
A number is chosen at random from the number –3, –2, –1, 0, 1, 2, 3. What will be the probability that square of this number is less then or equal to 1?
RD Sharma solutions for Mathematics [English] Class 10 16 Probability Exercise 16.4 [Pages 35 - 39]
If a digit is chosen at random from the digit 1, 2, 3, 4, 5, 6, 7, 8, 9, then the probability that it is odd, is
\[\frac{4}{9}\]
\[\frac{5}{9}\]
\[\frac{1}{9}\]
\[\frac{2}{3}\]
In Q. No. 1, The probability that the digit is even is
\[\frac{4}{9}\]
\[\frac{5}{9}\]
\[\frac{1}{9}\]
\[\frac{2}{3}\]
In the probability that the digit is a multiple of 3 is
\[\frac{1}{3}\]
\[\frac{2}{3}\]
\[\frac{1}{9}\]
\[\frac{2}{9}\]
If three coins are tossed simultaneously, then the probability of getting at least two heads, is
\[\frac{1}{4}\]
\[\frac{3}{8}\]
\[\frac{1}{2}\]
\[\frac{1}{4}\]
In a single throw of a die, the probability of getting a multiple of 3 is
\[\frac{1}{2}\]
\[\frac{1}{3}\]
\[\frac{1}{6}\]
\[\frac{2}{3}\]
The probability of guessing the correct answer to a certain test questions is\[\frac{x}{12}\] If the probability of not guessing the correct answer to this question is\[\frac{ 2}{3}\] then x =
2
3
4
6
A bag contains three green marbles, four blue marbles, and two orange marbles, If a marble is picked at random, then the probability that it is not an orange marble is
\[\frac{1}{4}\]
\[\frac{1}{3}\]
\[\frac{4}{9}\]
\[\frac{7}{9}\]
A number is selected at random from the numbers 3, 5, 5, 7, 7, 7, 9, 9, 9, 9 The probability that the selected number is their average is
\[\frac{1}{10}\]
\[\frac{3}{10}\]
\[\frac{7}{10}\]
\[\frac{9}{10}\]
The probability of throwing a number greater than 2 with a fair dice is
\[\frac{3}{5}\]
\[\frac{2}{5}\]
\[\frac{2}{3}\]
\[\frac{1}{3}\]
A card is accidently dropped from a pack of 52 playing cards. The probability that it is an ace is
\[\frac{1}{4}\]
\[\frac{1}{13}\]
\[\frac{1}{52}\]
\[\frac{12}{13}\]
A number is selected from numbers 1 to 25. The probability that it is prime is
\[\frac{2}{3}\]
\[\frac{1}{6}\]
\[\frac{1}{3}\]
\[\frac{5}{6}\]
Which of the following cannot be the probability of an event?
\[\frac{2}{3}\]
\[- 1 . 5\]
\[15 %\]
\[0 . 7\]
If P(E) = 0.05, then P(not E) =
−0.05
0.5
0.9
0.95
Which of the following cannot be the probability of occurence of an event?
0.2
0.4
0.8
1.6
The probability of a certain event is
0
1
1/2
no existent
The probability of an impossible event is
0
1
1/2
non-existent
Aarushi sold 100 lottery tickets in which 5 tickets carry prizes. If Priya purchased a ticket, what is the probability of Priya winning a prize?
\[\frac{19}{20}\]
\[\frac{1}{25}\]
\[\frac{1}{20}\]
\[\frac{17}{20}\]
A number is selected from first 50 natural numbers. What is the probability that it is a multiple of 3 or 5?
\[\frac{13}{25}\]
\[\frac{21}{50}\]
\[\frac{12}{25}\]
\[\frac{23}{50}\]
A month is selected at random in a year. The probability that it is March or October, is
\[\frac{1}{12}\]
\[\frac{1}{6}\]
\[\frac{3}{4}\]
None of these
From the letters of the word ''MOBILE", a letter is selected. The probability that the letter is a vowel, is
\[\frac{1}{3}\]
\[\frac{3}{7}\]
\[\frac{1}{6}\]
\[\frac{1}{2}\]
A die is thrown once. The probability of getting a prime number is
\[\frac{2}{3}\]
\[\frac{1}{3}\]
\[\frac{1}{2}\]
\[\frac{1}{6}\]
The probability of getting an even number, when a die is thrown once is
\[\frac{1}{2}\]
\[\frac{1}{3}\]
\[\frac{1}{6}\]
\[\frac{5}{6}\]
A box contains 90 discs, numbered from 1 to 90. If one disc is drawn at random from the box, the probability that it bears a prime number less than 23, is ______.
`7/90`
`9/90`
`1/9`
`10/90`
`4/15`
`4/45`
`8/89`
\[\frac{9}{89}\]
The probability that a number selected at random from the numbers 1, 2, 3, ..., 15 is a multiple of 4, is
\[\frac{4}{15}\]
\[\frac{2}{15}\]
\[\frac{1}{5}\]
\[\frac{1}{3}\]
Two different coins are tossed simultaneously. The probability of getting at least one head is
\[\frac{1}{4}\]
\[\frac{1}{8}\]
\[\frac{3}{4}\]
\[\frac{7}{8}\]
If two different dice are rolled together, the probability of getting an even number on both dice is
\[\frac{1}{36}\]
\[\frac{1}{2}\]
\[\frac{1}{6}\]
\[\frac{1}{4}\]
A number is selected at random from the numbers 1 to 30. The probability that it is a prime number is
\[\frac{2}{3}\]
\[\frac{1}{6}\]
\[\frac{1}{3}\]
\[\frac{11}{30}\]
A card is drawn at random from a pack of 52 cards. The probability that the drawn card is not an ace is
\[\frac{1}{13}\]
\[\frac{9}{13}\]
\[\frac{4}{13}\]
\[\frac{12}{13}\]
A number x is chosen at random from the numbers −3, −2, −1, 0, 1, 2, 3 the probability that | x | < 2 is
\[\frac{5}{7}\]
\[\frac{2}{7}\]
\[\frac{3}{7}\]
\[\frac{1}{7}\]
If a number x is chosen from the numbers 1, 2, 3, and a number y is selected from the numbers 1, 4, 9. Then, P(xy < 9)
\[\frac{7}{9}\]
\[\frac{5}{9}\]
\[\frac{2}{3}\]
\[\frac{1}{9}\]
The probability that a non-leap year has 53 sundays, is
\[\frac{2}{7}\]
\[\frac{5}{7}\]
\[\frac{6}{7}\]
\[\frac{1}{7}\]
In a single throw of a pair of dice, the probability of getting the sum a perfect square is
\[\frac{1}{18}\]
\[\frac{7}{36}\]
\[\frac{1}{6}\]
\[\frac{2}{9}\]
What is the probability that a non-leap year has 53 Sundays?
\[\frac{6}{7}\]
\[\frac{1}{7}\]
\[\frac{5}{7}\]
None of these
Two numbers 'a' and 'b' are selected successively without replacement in that order from the integers 1 to 10. The probability that\[\frac{a}{b}\] is an integer, is
\[\frac{17}{45}\]
\[\frac{1}{5}\]
\[\frac{17}{90}\]
\[\frac{8}{45}\]
Two dice are rolled simultaneously. The probability that they show different faces is
\[\frac{2}{3}\]
\[\frac{1}{6}\]
\[\frac{1}{3}\]
\[\frac{5}{6}\]
What is the probability that a leap year has 52 Mondays?
\[\frac{2}{7}\]
\[\frac{4}{7}\]
\[\frac{5}{7}\]
\[\frac{6}{7}\]
If a two digit number is chosen at random, then the probability that the number chosen is a multiple of 3, is
\[\frac{3}{10}\]
\[\frac{29}{100}\]
\[\frac{1}{3}\]
\[\frac{7}{25}\]
Two dice are thrown together. The probability of getting the same number on both dice is
\[\frac{1}{2}\]
\[\frac{1}{3}\]
\[\frac{1}{6}\]
\[\frac{1}{12}\]
In a family of 3 children, the probability of having at least one boy is
\[\frac{7}{8}\]
\[\frac{1}{8}\]
\[\frac{5}{8}\]
\[\frac{3}{4}\]
A bag contains cards numbered from 1 to 25. A card is drawn at random from the bag. The probability that the number on this card is divisible by both 2 and 3 is
\[\frac{1}{5}\]
\[\frac{3}{25}\]
\[\frac{4}{25}\]
\[\frac{2}{25}\]
Solutions for 16: Probability
![RD Sharma solutions for Mathematics [English] Class 10 chapter 16 - Probability RD Sharma solutions for Mathematics [English] Class 10 chapter 16 - Probability - Shaalaa.com](/images/8193647920-mathematics-english-class-10_6:5809898a5fef45e9a2f7e6b414d392fa.jpg)
RD Sharma solutions for Mathematics [English] Class 10 chapter 16 - Probability
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 10 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. RD Sharma solutions for Mathematics Mathematics [English] Class 10 CBSE 16 (Probability) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. RD Sharma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 10 chapter 16 Probability are Sample Space, Concept Or Properties of Probability, Simple Problems on Single Events, Probability - A Theoretical Approach, Basic Ideas of Probability, Basic Ideas of Probability, Probability - A Theoretical Approach, Basic Ideas of Probability, Random Experiments, Equally Likely Outcomes, Type of Event - Elementry, Type of Event - Complementry, Outcome, Sample Space, Event and Its Types, Probability of an Event, Type of Event - Exclusive, Type of Event - Exhaustive, Concept Or Properties of Probability, Addition Theorem.
Using RD Sharma Mathematics [English] Class 10 solutions Probability exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in RD Sharma Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 10 students prefer RD Sharma Textbook Solutions to score more in exams.
Get the free view of Chapter 16, Probability Mathematics [English] Class 10 additional questions for Mathematics Mathematics [English] Class 10 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
