Advertisements
Advertisements
Question
Construct a triangle ABC, given that the radius of the circumcircle of triangle ABC is 3.5 cm, ∠ BCA = 45° and ∠ BAC = 60°.
Solution
Steps of construction: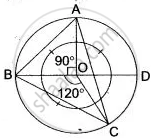
(i) Draw a circle with radius = 3.5 cm
(ii) Again make ∠ BOC = 120°
(iii) Draw diameter BOD and construct ∠ BOA = 90°
(iv) Join AB, AC, and BC. Then ∠ ABC is the required triangle.
APPEARS IN
RELATED QUESTIONS
Using a ruler and a compass, construct a triangle ABC in which AB = 7 cm, ∠CAB = 60° and AC = 5 cm. Construct the locus of :
- points equidistant from AB and AC.
- points equidistant from BA and BC.
Hence construct a circle touching the three sides of the triangle internally.
Use ruler and compasses only for the following questions. All constructions lines and arcs must be clearly shown
Construct a ABC in which BC = 6.5 cm, ABC = 60°, AB = 5 cm.
In the figure given below 'O' is the centre of the circle. If QR = OP and ∠ORP = 20°. Find the value of 'x' giving reasons

Construct a ΔABC with BC = 6.5 cm, AB = 5.5 cm, AC = 5 cm. Construct the incircle of the triangle. Measure and record the radius of the incircle.
Construct a triangle ABC in which base BC = 6 cm, AB = 5.5 cm and ∠ABC = 120°.
Construct a circle circumscribing the triangle ABC.
Draw a cyclic quadrilateral ABCD so that D is equidistant from B and C.
Construct Δ ABC in which AB = 5 cm, BC = 4. 5 cm and ∠ ABC = 60" .. Construct a cirde to circumscribe. Δ ABC.
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
Does the perpendicular bisector of BC pass through O?
In triangle ABC, ∠ABC = 90°, AB = 6 cm, BC = 7.2 cm and BD is perpendicular to side AC. Draw circumcircle of triangle BDC and then state the length of the radius of this circumcircle drawn.
Use ruler and compasses only for this question:
(i) Construct A ABC, where AB = 3.5 cm, BC = 6 cm and ∠ ABC = 60°.
(ii) Construct the locus of points inside the triangle which are equidistant from BA and BC.
(iii) Construct the locus of points inside the triangle which are equidistant from B and C.
(iv) Mark the point P which is equidistant from AB, BC, and also equidistant from B and C. Measure and record the length of PB.
(i) Construct a triangle ABC, in which AB = 5.0 cm, BC = 3.5 cm and ∠ ABC = `67 1/2°`
( Use a pair of compasses and ruler only.)
(ii) Construct a circle to touch AB at B and it pass though C.
