Advertisements
Advertisements
Question
Construct a triangle ABC with given conditions.
AB = 7 cm, AC = 6.5 cm and ∠A = 120°
Solution
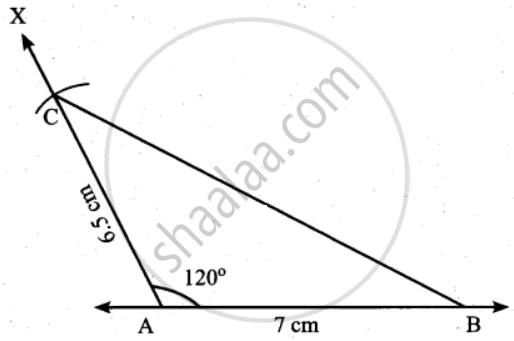

Construction:
Step 1: Drawn a line. Marked A and B on the line such that AB = 7 cm.
Step 2: At A, drawn a ray AX making an angle of 120° with AB.
Step 3: With A as centre, drawn an arc of radius 6.5 cm to cut the ray AX.
Marked the point of intersection as C.
Step 4: Joined BC.
ABC is the required triangle.
APPEARS IN
RELATED QUESTIONS
Consider the given pairs of triangles and say whether each pair is that of congruent triangles. If the triangles are congruent, say ‘how’; if they are not congruent say ‘why’ and also say if a small modification would make them congruent: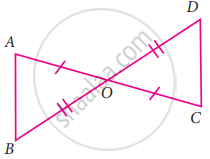
For the given pair of triangles state the criterion that can be used to determine the congruency?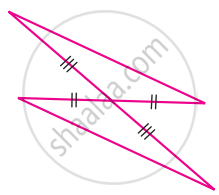
Construct a triangle PQR with given conditions.
∠P = 60°, ∠R = 35° and PR = 7.8 cm
In the given figure, ΔPQR ≅ Δ ______.

In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.

In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.

In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.
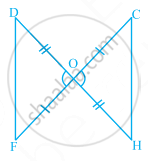
In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.

State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
∆PQR: PQ = 3.5 cm, QR = 4.0 cm, ∠Q = 60°
∆STU: ST = 3.5 cm, TU = 4 cm, ∠T = 60°
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
∆ABC: AB = 4.8 cm, ∠A = 90°, AC = 6.8 cm
∆XYZ: YZ = 6.8 cm, ∠X = 90°, ZX = 4.8 cm
