Advertisements
Advertisements
Question
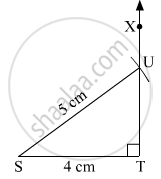
Construct a triangle of the measures given below.
In the right-angled ∆STU, hypotenuse SU = 5 cm and l(ST) = 4 cm.
Solution
Steps of construction:
- Draw the Base:
- Since ST = 4 cm ST = 4 cm using a ruler.
- Construct the Right Angle at T:
- Place the protractor at point T on the line ST.
- Mark a point where the angle measures 90∘
- Draw a perpendicular line from T.
- Mark the Hypotenuse SU:
- Set your compass to measure 5 cm (the length of the hypotenuse).
- Place the compass point at S and draw an arc intersecting the perpendicular line from T.
- Label the intersection as point U.
- Complete the Triangle:
- Connect U to S and U to T.
You now have a right-angled triangle ∆STU with SU = 5 cm and ST = 4 cm.
RELATED QUESTIONS
Draw an equilateral triangle with side 6.5 cm.
Draw triangle with the measures given below.
In ∆ NTS, m ∠T = 40°, l(NT) = l(TS) = 5 cm
Draw triangle with the measures given below.
In ∆FUN, l(FU) = 5 cm, l(UN) = 4.6 cm, m∠U = 110°
Construct a triangle of the measures given below.
In ∆SAT, l(AT) = 6.4 cm, m ∠A = 45°, m ∠T = 105°
Construct a triangle of the measures given below.
In ∆MNP, l(NP) = 5.2 cm, m∠N = 70°, m ∠P = 40°
Construct a triangle of the measures given below.
In ∆EFG, l(EG) = 6 cm, m∠F = 65°, m∠G = 45°
Students should take examples of their own and practise the construction of triangles.
Construct a right-angled triangle in which: QP = QR and hypotenuse PR = 7 cm
Construct an isosceles triangle in which: AB = AC, BC = 6 cm and ∠B = 75°
Construct a triangle using the given data: PQ - PR = 1.5 cm, QR = 6.0 and ∠Q = 45°
