Advertisements
Advertisements
Question
Construct a triangle XYZ with the given conditions.
XY = 6.4 cm, ZY = 7.7 cm and XZ = 5 cm
Solution

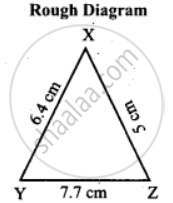
Construction:
Step 1: Draw a line. Marked Y and Z on the line such that YZ = 7.7 cm.
Step 2: With Y as centre drawn an arc of radius 6.4 cm above the line YZ.
Step 3: With Z as centre, drawn an arc or radius 5 cm to intersect arc drawn in steps.
Marked the point of intersection as X.
Step 4: Joined YX and ZX.
Now XYZ is the required triangle.
APPEARS IN
RELATED QUESTIONS
In triangles ABC and PQR, AB = 3.5 cm, BC = 7.1 cm, AC = 5 cm, PQ = 7.1 cm, QR = 5 cm and PR = 3.5 cm. Examine whether the two triangles are congruent or not. If yes, write the congruence relation in symbolic form.
Consider the given pairs of triangles and say whether each pair is that of congruent triangles. If the triangles are congruent, say ‘how’; if they are not congruent say ‘why’ and also say if a small modification would make them congruent: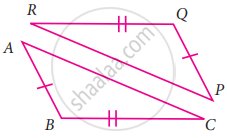
Construct a triangle XYZ with the given conditions.
An equilateral triangle of side 7.5 cm
In the given figure FG = FI and H is midpoint of GI, prove that ∆FGH ≅ ∆FHI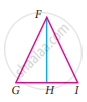
If D is the mid-point of the side BC in ∆ABC where AB = AC, then ∠ADC is ______.
In the following pairs of triangles of figure, the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
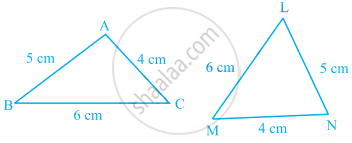
In the following pairs of triangles of figure, the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.

ABC is an isosceles triangle with AB = AC and D is the mid-point of base BC (see figure).
- State three pairs of equal parts in the triangles ABD and ACD.
- Is ∆ABD ≅ ∆ACD. If so why?

In the given figure, it is given that LM = ON and NL = MO
- State the three pairs of equal parts in the triangles NOM and MLN.
- Is ∆NOM ≅ ∆MLN. Give reason?

Triangles DEF and LMN are both isosceles with DE = DF and LM = LN, respectively. If DE = LM and EF = MN, then, are the two triangles congruent? Which condition do you use? If ∠E = 40°, what is the measure of ∠N?
