Question

Construct the following and give justification:

A triangle if its perimeter is 10.4 cm and two angles are 45° and 120°.

Answer 1

Step 1: Draw a line XY = 10.4 i.e., the perimeter.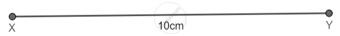

Step 2: Construct an angle equal to ∠B = 45° and another angle equal to ∠C = 120°

Step 3: Bisect these angles and name the intersecting point as A.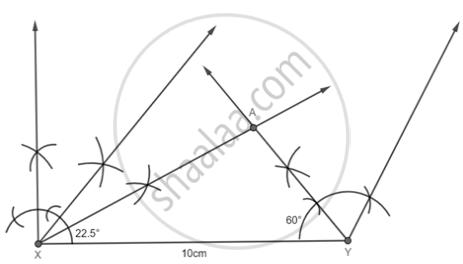

Step 4: Construct perpendicular bisectors of AX and AY and name the PQ and RS respectively.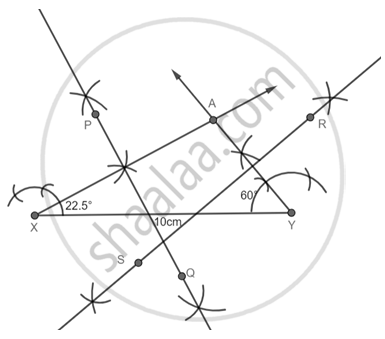

Step 5: Name the intersecting point of PQ and XY as B and RS the intersecting point of RS and XY as C.

Join AB and AC.

ABC is the required triangle.

Justification:

As B is on line PQ which is the perpendicular bisector of AX,

AB + BC + CA = XB + BC + CY = XY

Then, ∠BAX = ∠AXB  ...(As in triangle AXB, AB is equal to XB)

As ∠ABC is the external angle of triangle AXB

Then, ∠ABC = ∠BAX + ∠AXB  ...(Exterior angle sum property)

∠ABC = ∠AXB + ∠AXB

∠ABC = 2∠AXB = 45° or ∠B.

Similarly, ∠ACB = 2∠CAY = 120° or ∠C.

Thus the construction is justified.