Advertisements
Advertisements
Question
Construct ∆XYZ such that l(XY) = 3.7 cm, l(YZ) = 7.7 cm, l(XZ) = 6.3 cm.
Solution
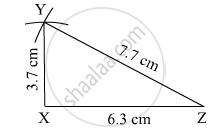
Steps of construction:
- Draw seg XZ of length 6.3 cm.
- Draw an arc of 3.7 cm from the vertex X.
- Draw another arc of 7.7 cm from the vertex Z, cutting the previously drawn arc at Y.
Therefore, △XYZ is the required triangle.
RELATED QUESTIONS
In ∆ PQR, l(PQ) = 6 cm, l(QR) = 3.8 cm, l(PR) = 4.5 cm
Draw an isosceles triangle with base 5 cm and the other sides 3.5 cm each.
Choose the lengths of the sides yourself and draw one equilateral, one isosceles and one scalene triangle.
Construct a triangle of the measures given below.
In ∆EFG, l(EG) = 6 cm, m∠F = 65°, m∠G = 45°
Construct a triangle using the given data: PQ = 4.8cm, QR = 6.3cm and PR = 5.5cm
Construct a right-angled triangle in which: Side AB = 4.5 cm and hypotenuse AC = 7 cm
Construct an isosceles triangle in which: AB = AC, BC = 6 cm and ∠B = 75°
Construct a triangle using the following data: PQ + PR = 10.6 cm, QR = 4.8 cm and ∠R = 45°
Construct a triangle using the given data: XY - XZ = 1.5 cm, YZ = 3.4 cm and ∠X = 45°
Construct a triangle PQR with PQ = 5.4 cm, QR = 4.6 cm and ∠Q = 60°. Draw the perpendicular PS at QR, measure the lengths of SP and SQ,
