Advertisements
Advertisements
Question
Draw the graph for the linear equation given below:
x - 3 = `(2)/(5)(y + 1)`
Solution
The equation will become:
5x - 2y = 17
First prepare a table as follows:
| x | - 1 | 0 | 1 |
| y | - 11 | `-(17)/(2)` | - 6 |
Thus the graph can be drawn as follows:
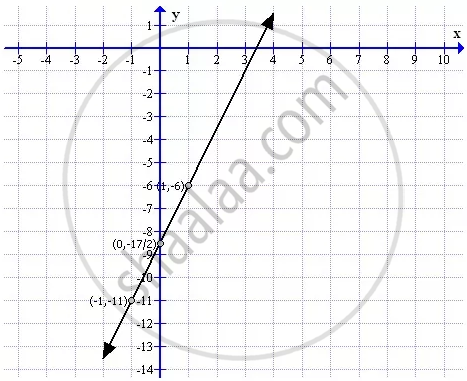
APPEARS IN
RELATED QUESTIONS
Draw the graph for the linear equation given below:
3y + 5 = 0
Draw the graph for the linear equation given below:
y = x
Draw the graph for the linear equation given below:
y = 2x + 3
Draw the graph for the each linear equation given below:
y = `(3x)/(2) + (2)/(3)`
For the pair of linear equations given below, draw graphs and then state, whether the lines drawn are parallel or perpendicular to each other.
2x - 3y = 6
`x/(2) + y/(3) = 1`
Draw the graph of the equation 3x - 4y = 12.
Use the graph drawn to find:
(i) y1, the value of y, when x = 4.
(ii) y2, the value of y, when x = 0.
Draw a graph of each of the following equations: 5x + 2y = 16
Draw a graph of each of the following equations: 2(x - 5) = `(3)/(4)(y - 1)`
Draw a graph for each of the following equations and find the coordinates of the points where the line drawn meets the x-axis and y-axis: 2x + 3y = 12
Draw the graph of the lines represented by the equations x + y = 4 and 2x - y = 2 on the same graph. Find the coordinates of the point where they intersect
