Advertisements
Advertisements
Question
Draw a graph of each of the following equations: 2(x - 5) = `(3)/(4)(y - 1)`
Solution
2(x - 5) = `(3)/(4)(y - 1)`
⇒ 8(x - 5) = 3(y - 1)
⇒ 8x - 40 = 3y - 3
⇒ 3y = 8x - 40 + 3
⇒ 3y = 8x - 37
y = `(8x - 37)/(3)`
When x = 2, = `(8(2) - 37)/(3)` = -7
When x = 5, y = `(8(5) - 37)/(3)` = 1
When x = -1, y = `(8(-1) - 37)/(3)` = -15
| x | 2 | 5 | -1 |
| y | -7 | 1 | -15 |
Plotting the points (2, -7), (5, 1) and (-1, -15), we get a line AB as shown in the figure.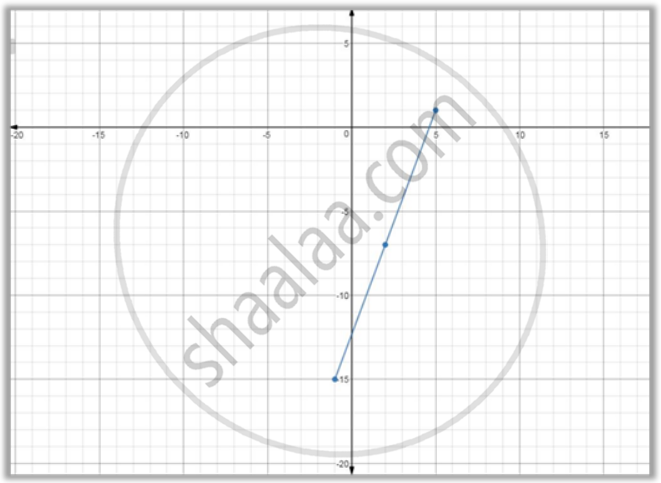
APPEARS IN
RELATED QUESTIONS
The following distribution gives the daily income of 50 workers of a factory.
| Daily income (in ₹) | 200-220 | 220-240 | 240-260 | 260-280 | 280-300 |
| Number of workers | 12 | 14 | 8 | 6 | 10 |
Convert the distribution above to a 'less than type' cumulative frequency distribution and draw its ogive.
Draw the graph for the linear equation given below:
y + 6 = 0
Draw the graph for the linear equation given below:
y = - x
Draw the graph for the linear equation given below:
y = x
Draw the graph for the linear equation given below:
2x - 3y = 4
For the linear equation, given above, draw the graph and then use the graph drawn (in the following case) to find the area of a triangle enclosed by the graph and the co-ordinates axes:
3x - (5 - y) = 7
Draw the graph of the equation 3x - 4y = 12.
Use the graph drawn to find:
(i) y1, the value of y, when x = 4.
(ii) y2, the value of y, when x = 0.
Find if the following points are collinear or not by using a graph:
(i) (-2, -1), (0, 3) and (1, 5)
(ii) (1, 3), (-2, -4) and (3, 5)
(iii) (2, -1), (2, 5) and (2, 7)
(iv) (4, -1), (-5, -1) and (3, -1)
Draw a graph of each of the following equations: x + 6y = 15
Draw a graph of each of the following equations: x = -3y
