Advertisements
Advertisements
Question
Each side of a rectangle is doubled. Find the ratio between :
(i) perimeters of the original rectangle and the resulting rectangle.
(ii) areas of the original rectangle and the resulting rectangle.
Solution
Let length of the rectangle = x
and breadth of the rectangle = y
(i)
Perimeter P = 2(x + y)
Again, new length = 2x
New breadth = 2y
∴ New perimeter P' = 2(2x + 2y)
= 4(x + y) = 2.2(x + y) = 2P
= `"P"/("P"') = 1/2` i.e. P : P' = 1 : 2
(ii)
Area A = xy
New Area A' = (2x)(2y) = 4xy = 4A
∴ `"A"/("A"') = 1/4` i.e. A : A' = 1 : 4
APPEARS IN
RELATED QUESTIONS
A rectangular piece of paper has area = 24 cm² and length = 5 cm. Find its perimeter.
Each rectangular tile is 40 cm long and 30 cm wide. How many tiles will be required to cover the floor of a room with length = 4.8 m and breadth = 2.4 m.
How much wire will be needed to make a rectangle 7 cm long and 4 cm wide?
A game requires its players to run around a square playground. Each side of the playground is 20 m long. One player took 5 rounds around the playground. How many metres did he run altogether?
The sides of a triangle are 24.7cm, 20.4 cm, and 10.5 cm respectively. What is the perimeter of the triangle?
Find the perimeter and the area of the rectangle whose length is 6 m and breadth is 4 m
The length of a rectangle is three times its breadth. If its perimeter is 64 cm, find the sides of the rectangle
How many different rectangles can be made with a 48 cm long string? Find the possible pairs of length and breadth of the rectangles.
Find the perimeter of the following figure
What is the perimeter of the following figures? What do you infer from the answers?
 (a) |
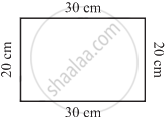 (c) |
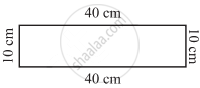 (b) |
 (d) |
