Advertisements
Advertisements
Question
Evaluate the following, using suitable identity
1032
Solution
1032 = (100 + 3)2
Taking a = 100 and b = 3
(a + b)2 = a2 + 2ab + b2 becomes
(100 + 3)2 = 1002 + 2(100)(3) + 32
= 10000 + 600 + 9
1032 = 10609
APPEARS IN
RELATED QUESTIONS
Use a suitable identity to get the following products.
(2y + 5) (2y + 5)
Simplify (4m + 5n)2 + (5m + 4n)2
Simplify (ab + bc)2 − 2ab2c
Using identities, evaluate 1.05 × 9.5
Use an expansion formula to find the value.
(102)2
Expand: (2x + 3y)2
(x2 + y2)(y2 + x2) = (x2 + y2)2
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
x2 + 2x + 1
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
a2x2 + 2abx + b2
Take suitable number of cards given in the adjoining diagram [G(x × x) representing x2, R(x × 1) representing x and Y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles: x2 + 4x + 4. Factorise 2x2 + 6x + 4 by using the figure.
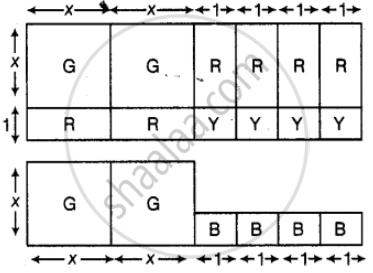
Calculate the area of figure.
