Advertisements
Advertisements
Question
Explain the formation of stationary waves.
Solution
Consider two harmonic progressive waves (formed by strings) that have the same amplitude and same velocity but move in opposite directions. Then the displacement of the first wave (incident wave) is
y1 = A sin (kx – ωt) (waves move toward right) …(1)
and the displacement of the second wave (reflected wave) is
y2 = A sin (kx + ωt) (waves move toward left) …(2)
both will interfere with each other by the principle of superposition, the net displacement is
y = y1 + y2 …… (3)
Substituting equation (1) and equation (2) in equation (3), we get
y = A sin (kx – ωt) + A sin (kx + ωt) …(4)
Using trigonometric identity, we rewrite equation (4) as
y(x, t) = 2A cos (ωt) sin (kx) …(5)
This represents a stationary wave or standing wave, which means that this wave does not move either forward or backward, whereas progressive or travelling waves will move forward or backward. Further, the displacement of the particle in equation (5) can be written in more compact form,
y(x, t) = A’ cos (ωt)
where, A’ = 2A sin (foe), implying that the particular element of the string executes simple harmonic motion with amplitude equals to A’. The maximum of this amplitude occurs at positions for which
sin (kx) = 1 `=> "kx" = pi/2, (3pi)/2, (5pi)/2,... = "m"pi`
where m takes half-integer or half-integral values. The position of maximum amplitude is known as antinode. Expressing wave number in terms of wavelength, we can represent the anti-nodal positions as
`"x"_"m" = ((2"m" + 1)/2) lambda/2`, where, m = 0, 1, 2...
For m = 0 we have maximum at `x_0 = lambda/2`
For m = 1 we have maximum at `x_1 = (3lambda)/4`
For m = 2 we have maximum at `x_2 = (5lambda)/4` and so on.
The distance between two successive antinodes can be computed by
`"x"_"m" - "x"_("m - 1") = ((2"m" + 1)/2) lambda/2 - (((2"m" + 1) + 1)/2) lambda/2 = lambda/2`
Similarly, the minimum of the amplitude A’ also occurs at some points in the space, and these points can be determined by setting
sin (kx) = 0 ⇒ kx = 0, π, 2π, 3π, … = nπ
where n takes integer or integral values. Note that the elements at these points do not vibrate (not move), and the points are called nodes. The nth nodal positions is given by,
`"x"_"n" = "n" lambda/2` where, n = 0, 1, 2,...
For n = 0 we have minimum at `x_0 = 0`
For n = 1 we have minimum at `"x"_1 = lambda/2`
For n = 2 we have maximum at `"x"_2 = lambda` and so on.
The distance between any two successive nodes can be calculated as
`"x"_"n" - "x"_("n - 1") = "n" lambda/2 - ("n - 1") lambda/2 = lambda/2`
APPEARS IN
RELATED QUESTIONS
For a particular tube, among six harmonic frequencies below 1000 Hz, only four harmonic frequencies are given: 300 Hz, 600 Hz, 750 Hz and 900 Hz. What are the two other frequencies missing from this list?
Let y = `1/(1 + x^2)` at t = 0 be the amplitude of the wave propagating in the positive x-direction. At t = 2s, the amplitude of the wave propagating becomes y = `1/(1 + (x - 2)^2)`. Assume that the shape of the wave does not
change during propagation. The velocity of the wave is:
What is a stationary wave?
Write the characteristics of stationary waves.
Discuss the law of transverse vibrations in stretched strings.
Explain the concepts of fundamental frequency, harmonics and overtones in detail.
What is a sonometer? Give its construction and working. Explain how to determine the frequency of tuning fork using a sonometer.
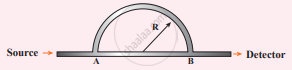
A sound wave is transmitted into a tube as shown in the figure. The sound wave splits into two waves at point A which recombine at point B. Let R be the radius of the semi-circle which is varied until the first minimum. Calculate the radius of the semi-circle if the wavelength of the sound is 50.0 m.
Police in a siren car moving with a velocity 20 ms– chases a thief who is moving in a car with a velocity v0 ms-1. The police car sounds at frequency 300 Hz, and both of them move towards a stationary siren of frequency 400 Hz. Calculate the speed in which thief is moving. (Assume the thief does not observe any beat)
