Advertisements
Advertisements
Question
Figure (a) shows a spring of force constant k clamped rigidly at one end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure (b) shows the same spring with both ends free and attached to a mass mat either end. Each end of the spring in Fig. (b) is stretched by the same force F.
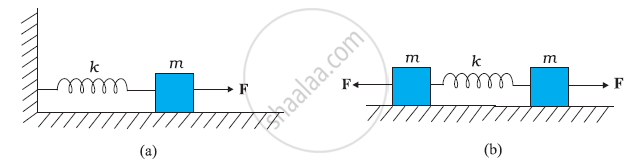
(a) What is the maximum extension of the spring in the two cases?
(b) If the mass in Fig. (a) and the two masses in Fig. (b) are released, what is the period of oscillation in each case?
Solution 1
a) For the one block system:
When a force F, is applied to the free end of the spring, an extension l, is produced. For the maximum extension, it can be written as:
F = kl
Where, k is the spring constant
Hence, the maximum extension produced in the spring, `l = F/k`
For the two block system:
The displacement (x) produced in this case is:
`x = l/2`
Net force, `F = +2 kx = 2k l/2`
`:. l = F/k`
b) For the one block system:
For mass (m) of the block, force is written as:
`F = ma = m (d^2x)/dt^2`
Where, x is the displacement of the block in time t
`:. m (d^2x)/(dt^2) = -kx`
It is negative because the direction of elastic force is opposite to the direction of displacement.
`(d^2x)/(dt^2) = - (k/m) x = - omega^2x`
Where, `omega^2 = k/m`
Where
ω is angular frequency of the oscillation
:. Time period of the oscillation, `T = (2pi)/omega`
`= (2pi)/sqrt(k/m) = 2pi sqrt(m/k)`
For the two block system:
`F = m (d^2x)/(dt^2)`
`m (d^2x)/(dt^2) = - 2kx`
where
Angular frequency, `omga = sqrt((2k)/m)`
:. Time period, `T = (2pi)/omega =( 2pi)sqrt(m/(2k))`
Solution 2
a) Let y be the maximum extension produced in the spring in figure a
Then F = ky (in magnitude)
`:. y = F/k`
if figure (b) the force on one mass acts as the force of reaction due to the force on the other mass. Therefore, each mass behaves as if it is fixed with respect to the other.
Therefore `F= ky => y = F/k`
b) In figure (a) F= -ky
`=> ma = -ky => a = k/m y`
`:.omega^2 = k/m` i.e `omega = sqrt(k/m)`
Therefore, `period T = (2pi)/omega = 2pi sqrt(m/k)`
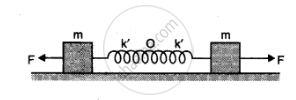
In figure (b) we may consider that the centre of the system is O and there are two springs each of length `l/2` attached to the two masses, each m, so that k' is the spring factor of each of the springs
Then, `K' = 2k`
`:. T = 2pi sqrt(m/k')`
`= 2pi sqrt(m/2k)`
APPEARS IN
RELATED QUESTIONS
The motion of a particle executing simple harmonic motion is described by the displacement function,
x (t) = A cos (ωt + φ).
If the initial (t = 0) position of the particle is 1 cm and its initial velocity is ω cm/s, what are its amplitude and initial phase angle? The angular frequency of the particle is π s–1. If instead of the cosine function, we choose the sine function to describe the SHM: x = B sin (ωt + α), what are the amplitude and initial phase of the particle with the above initial conditions.
Figures correspond to two circular motions. The radius of the circle, the period of revolution, the initial position, and the sense of revolution (i.e. clockwise or anti-clockwise) are indicated on each figure

Obtain the corresponding simple harmonic motions of the x-projection of the radius vector of the revolving particle P, in each case.
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
sin3 ωt
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
3 cos `(π/4 – 2ω"t")`
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
cos ωt + cos 3ωt + cos 5ωt
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
exp (–ω2t2)
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
1 + ωt + ω2t2
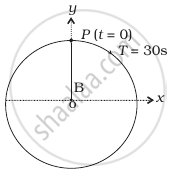
Figure shows the circular motion of a particle. The radius of the circle, the period, sense of revolution and the initial position are indicated on the figure. The simple harmonic motion of the x-projection of the radius vector of the rotating particle P is ______.
The rotation of earth about its axis is ______.
- periodic motion.
- simple harmonic motion.
- periodic but not simple harmonic motion.
- non-periodic motion.
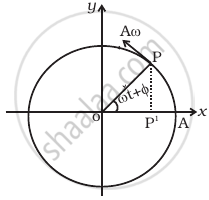
In figure, what will be the sign of the velocity of the point P′, which is the projection of the velocity of the reference particle P . P is moving in a circle of radius R in anticlockwise direction.