Advertisements
Advertisements
Question
Find the area enclosed by each of the following figures [Fig. 20.49 (i)-(iii)] as the sum of the areas of a rectangle and a trapezium: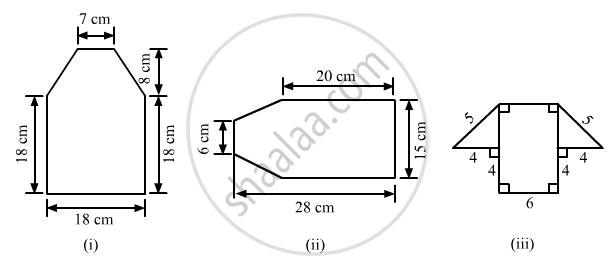
Solution
 \[(i)\]
\[(i)\]
The given figure can be divided into a rectangle and a trapezium as shown below:
From the above firgure:
Area of the complete figure = (Area of square ABCF)+(Area of trapezium CDEF)
\[=(AB\times BC)+[\frac{1}{2}\times(FC+ED)\times(\text{ Distance between FC and ED })]\]
\[=(18\times18)+[\frac{1}{2}\times(18+7)\times(8)]\]
\[=324+100\]
\[ {=424 cm}^2\]
\[(ii)\]
The given figure can be divided in the following manner:\]
From the above figure:
AB = AC-BC=28-20=8 cm
So that area of the complete figure = (area of rectangle BCDE)+(area of trapezium ABEF)
\[=(BC\times CD)+[\frac{1}{2}\times(BE+AF)\times(AB)]\]
\[=(20\times15)+[\frac{1}{2}\times(15+6)\times(8)]\]
\[=300+84\]
\[ {=384 cm}^2\]
The given figure can be divided in the following manner:
From the above figure:
EF = AB = 6 cm
Now, using the Pythagoras theorem in the right angle triangle CDE:
\[ 5^2 {= 4}^2 {+CE}^2 \]
\[ {CE}^2 = 25-16=9\]
\[CE =\sqrt{9}= 3 cm\]
\[\text{ And, }GD=GH+HC+CD=4+6+4=14 cm\]
\[ \therefore\text{ Area of the complete figure }= (\text{ Area of rectangle ABCH })+(\text{ Area of trapezium GDEF })\]
\[=(AB\times BC)+[\frac{1}{2}\times(GD+EF)\times(CE)]\]
\[=(6\times4)+[\frac{1}{2}\times(14+6)\times(3)]\]
\[=24+30\]
\[ {=54 cm}^2\]
APPEARS IN
RELATED QUESTIONS
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 150 cm and 30 dm, altitude = 9 dm.
Top surface of a table is trapezium in shape. Find its area if its parallel sides are 1 m and 1.2 m and perpendicular distance between them is 0.8 m.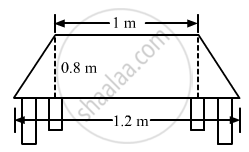
The area of a trapezium is 91 cm2 and its height is 7 cm. If one of the parallel sides is longer than the other by 8 cm, find the two parallel sides.
The area of a trapezium is 384 cm2. Its parallel sides are in the ratio 3 : 5 and the perpendicular distance between them is 12 cm. Find the length of each one of the parallel sides.
There is a pentagonal shaped park as shown in Fig. 20.50. Jyoti and Kavita divided it in two different ways.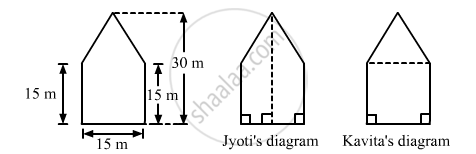
Find the area of this park using both ways. Can you suggest some another way of finding its areas?
The following figure shows the cross-section ABCD of a swimming pool which is a trapezium in shape.
If the width DC, of the swimming pool, is 6.4 m, depth (AD) at the shallow end is 80 cm and depth (BC) at the deepest end is 2.4 m, find its area of the cross-section.
In a trapezium if the sum of the parallel sides is 10 cm and the area is 140 sq.cm, then the height is
A ground is in the form of isosceles trapezium with parallel sides measuring 42 m and 36 m long. The distance between the parallel sides is 30 m. Find the cost of levelling it at the rate of ₹ 135 per sq.m
The perimeter of a trapezium is 52 cm and its each non-parallel side is equal to 10 cm with its height 8 cm. Its area is ______.
Find the area of the following fields. All dimensions are in metres.

