Advertisements
Advertisements
Question
Find the equation of a line passing through the intersection of `"x"/10 + "y"/5` = 14 and `"x"/8 + "y"/6` = 15 and perpendicular to the line x - 2y = 5
Solution
`"x"/10 + "y"/5` = 14 ⇒ x + 2y = 140......(1)
`"x"/8 + "y"/6` = 15 ⇒ 3x + 4y = 360........(2)
(1) can be rewritten as 2x + 4y = 280......(3)
(2) can be rewritten as 3x _ 4y = 360........(4)
subtracting (3) from (4), we get
x = 80
y = 30
Point of intersection of (1) and (2) is (80,30)
slope of x - 2y = 5 is`1/2`
Equation of required line is `("y" - "y"_1)/("x" - "x"_1)` = m
`("y" - 30)/("x" -80)` = -2
⇒ -2x + 160 = y - 30
⇒ 2x + y = 190
APPEARS IN
RELATED QUESTIONS
Find the equation of the line passing through (−5, 7) and parallel to y-axis
Find the equation of the line passing through (5, –3) and parallel to x – 3y = 4.
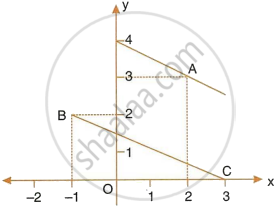
In the following diagram, write down:
- the co-ordinates of the points A, B and C.
- the equation of the line through A and parallel to BC.
A(1, −5), B(2, 2) and C(−2, 4) are the vertices of triangle ABC. Find the equation of the line through C and parallel to AB.
A(8, −6), B(−4, 2) and C(0, −10) are vertices of a triangle ABC. If P is the mid-point of AB and Q is the mid-point of AC, use co-ordinate geometry to show that PQ is parallel to BC. Give a special name to quadrilateral PBCQ.
ABCD is a parallelogram where A(x, y), B(5, 8), C(4, 7) and D(2, −4). Find:
- co-ordinates of A.
- equation of diagonal BD.
Find the slope of a line perpendicular to the foloowing line x - `(3"y")/2 + 1 = 0`
Find the slope of a line perpendicular to the foloowing line 3x - 5y = 9
Find the slope of a line perpendicular to the foloowing line 4x + y = 7
Find the equation of a line perpendicular to the join of A(3,5) and B(-1,7) if it passes through the midpoint of AB.
