Question

A biconvex lens with its two faces of equal radius of curvature R is made of a transparent medium of refractive index μ₁. It is kept in contact with a medium of refractive index μ₂ as shown in the figure.

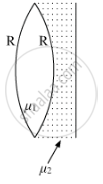
(a) Find the equivalent focal length of the combination.
(b) Obtain the condition when this combination acts as a diverging lens.
(c) Draw the ray diagram for the case μ₁ > (μ₂ + 1) / 2, when the object is kept far away from the lens. Point out the nature of the image formed by the system.

Answer 1

From the lens maker formula, we have

`1/f=(μ−1)(1/R_1−1/R_2)`

where  f=Focal length the lens

 μ=Refractive index of material

R₁=Radius of curvature of first face

R₂=Radius of curvature of second face

Let f₁ anf f₂ be the focal lengths of the two mediums. Then,

`1/f_1=(μ_1−1)[1/R−(−1/R)]`

`⇒1/f=(μ_1−1)(2/R)1/f_2`

`=(μ_2−1)[(−1/R)−1/∞]`

`⇒1/f_2=(μ_2−1)(−1/R)`

(a) If f_eq is the equivalent focal length of the combination, then

`1/f_eq=1/f_1+1/f_2`

`⇒1/f_eq=(2(μ_1−1))/R−(μ_2−1)/R`

`⇒1/f_eq=(2μ_1−μ_2−1)/R`

`⇒f_eq=R/(2μ_1−μ_2−1)`

(b) For the combination to behave as a diverging lens, feq < 0

`⇒R/(2μ_1−μ_2−1)<0`

`⇒2μ_1−μ_2−1<0`

`⇒μ_1<(μ_2+1)/2`

which is the required condition

(c) For μ1>(μ2+1)/2, the combination will behave as the converging lens. So, an object placed far away from the lens will form image at the focus of the lens.

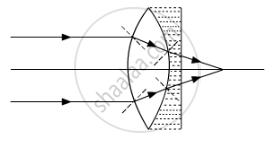
The image so formed will be real and diminished in nature.