Advertisements
Advertisements
Question
Find the midpoint of the line segment joining the following pair of point :
(3a-2b, Sa+7b) and (a+4b, a-3b)
Solution

Coordinates of C are ,
C (x , y) = C `(("a + 4b + 3a - 2b")/2 , ("a - 3b + 5a + 7b")/2)`
= C (2a + b , 3a + 2b)
APPEARS IN
RELATED QUESTIONS
ABCD is a parallelogram where A(x, y), B(5, 8), C(4, 7) and D(2, -4). Find
1) Coordinates of A
2) An equation of diagonal BD
Find the mid-point of the line segment joining the points:
(–6, 7) and (3, 5)
Complete the table below the graph with the help of the following graph.

| Sr. No. | First point | Second point | Co-ordinates of first point (x1 , y1) | Co-ordinates of second point (x2 , y2) | `(y_2 - y_2)/(x_2 - x_2)` |
| 1 | C | E | (1, 0) | (3,4) | `4/2=2` |
| 2 | A | B | (-1,-4) | (0,-2) | `2/1 = 2` |
| 3 | B | D | (0,-2) | (2,2) | `4/2=2` |
(4, 2) and (-1, 5) are the adjacent vertices ofa parallelogram. (-3, 2) are the coordinates of the points of intersection of its diagonals. Find the coordinates of the other two vertices.
Find the mid-point of the line segment joining the points
`(1/2, (-3)/7)` and `(3/2, (-11)/7)`
The centre of a circle is (−4, 2). If one end of the diameter of the circle is (−3, 7) then find the other end
In what ratio does the point Q(1, 6) divide the line segment joining the points P(2, 7) and R(−2, 3)
Find coordinates of midpoint of the segment joining points (0, 2) and (12, 14)
From the figure given alongside, find the length of the median AD of triangle ABC. Complete the activity.
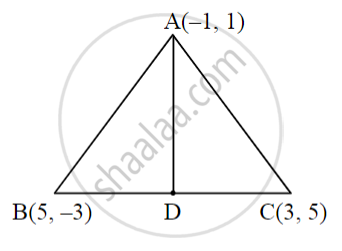
Solution:
Here A(–1, 1), B(5, – 3), C(3, 5) and suppose D(x, y) are coordinates of point D.
Using midpoint formula,
x = `(5 + 3)/2`
∴ x = `square`
y = `(-3 + 5)/2`
∴ y = `square`
Using distance formula,
∴ AD = `sqrt((4 - square)^2 + (1 - 1)^2`
∴ AD = `sqrt((square)^2 + (0)^2`
∴ AD = `sqrt(square)`
∴ The length of median AD = `square`
ABC is a triangle whose vertices are A(1, –1), B(0, 4) and C(– 6, 4). D is the midpoint of BC. Find the coordinates of D.
