Advertisements
Advertisements
Question
Find the support reactions at A and B for the beam loaded as shown in the given figure.
Solution 1
Given : Various forces on beam
To find : Support reactions at A and B
Solution: Draw PQ ⊥to RS
Effective force of uniform load =20 x 6 = 120 kN
2 + `6/2` = 5 m
This load acts at 5m from A
Effective force of uniformly varying load =`1/2` x (80-20) x 6
=180 kN
2+`6/3` x 2 =6m
This load acts at 6m from A
The beam is in equilibrium
Applying the conditions of equilibrium
ΣMA = 0
-120 x 5 -180 x 6 +RBcos30 x 10 -80 x 13 =0
10RBcos 30 = 120 x 5+180 x 6 + 80 x13
RB = 314.0785 N
Reaction at B will be at 60o in second quadrant
ΣFx = 0
RAcosα - RBsin30 = 0
RAcosα - 314.0785 x 0.5 = 0
RAcosα = 157.0393 N ……..(1)
ΣFy = 0
RAsinα - 120 - 180 + RBcos30 - 80 = 0
RAsinα = 12 + 180 -314.0785 x0.866 + 80
RAsinα = 108.008N ……..(2)
Squaring and adding (1) and (2)
RA2(sin2α + cos2α) = 36325.3333
RA = 190.5921 N
Dividing (2) by (1)
`(R_Asinalpha)/(R_Acosalpha)=(108.008)/(157.0393)`
α=tan-1(0.6877)
=34.5173o
Reaction at point A = 190.5921 N at 34.5173o in first quadrant
Reaction at B = 314.0785 N at 60o in second quadrant
Solution 2
As Shown in FBD,
Taking two components of RA & taking support reaction at pt. B which inclined at angle 60∘ with horizontal. Also converting the trapezoidal load into equivalent point loads we get FBD
Now , applying conditions of equilibrium
`sumF_x = H_A - R_B cos 60 = 0` ------(1)
`sumF_Y = V_A - 120 -180-80 +R_B sin 60 = 0` -----(2)
120(5) + 180(6) - RB sin 60(10) + 80(13) = 0
RB = 314.08 KN at θ = 60°
Putting in eqs. (1) and (2), we get
HA = 157.04KN,VA = 108KN
So, RA = 190.59 KN at θ = 34.52°
Support reaction at A is 190.59 KN at θ = 34.52° & at N is RB = 314.08 KN at θ = 60°

APPEARS IN
RELATED QUESTIONS
State Lami’s theorem.
State the necessary condition for application of Lami’s theorem.
Three blocks A,B and C of masses 3 kg,2 kg and 7 kg respectively are connected as shown.Determine the acceleration of A,B and C.Also find the tension in the string.
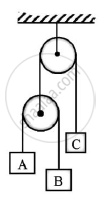
Given : mA=3kg
mB=2 kg
mC=7kg
To find: Acceleration of blocks A,B and C
Block A of weight 2000N is kept on the inclined plane at 35° .It is connected to weight B by an inextensible string passing over smooth pulley.
Determine the weight of pan B so that B just moves down.Assume μ=0.2.
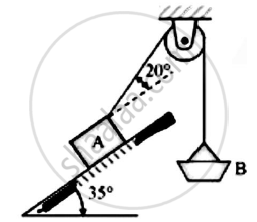
Given : Weight of block A=2000N
Angle of inclined plane = 35°
μ=0.2
To find : Weight of pan B
A rod AD of length 40 cm is suspended from point D as shown in figure. If it has a weight of 25 N and also supports a load of 40N,find the tension in the cable using the method of virtual work.Take AC=30 cm.
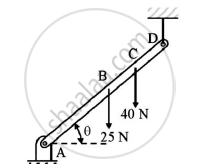
Given : Length of rod AD=40cm=0.4m
AC=0.3m
W=25N
Load on rod AD=40N
To find : Tension in the cable
