Advertisements
Advertisements
Question
Find the lateral surface area, total surface area and the volume of the following cylinders: Radius = 4.2cm, Height = 12cm
Solution
Given that:
Radius (r) = 4.2cm
Height (h) = 12cm
We know that:
Lateral surface Area (L.S.A) of cylinder = 2 x π x r x h
Total surface Area (T.S.A) of cylinder = (2 x π x r x h) + (2 x π x r2)
Volume of cylinder = π x r2 x h
L.S.A of cylinder
= 2 x π x r x h
= `2 xx (22)/(7) xx 4.2 xx 12`
= 316.8cm2
T.S.A of cylinder
= (2 x π x t x h) + (2 x π x r2)
= `(2 xx (22)/(7) xx 4.2 xx 12) + (2 xx (22)/(7) xx 4.2^2)`
= 316.8 + 110.88
= 427.68cm2
Volume of cylinder
= π x r2 x h
= `(22)/(7) xx 4.2^2 xx 12`
= 665.28cm3.
APPEARS IN
RELATED QUESTIONS
Curved surface area of a right circular cylinder is 4.4 `m^2`. If the radius of the base of the cylinder is 0.7 m, find its height.
A well with 10 m inside diameter is dug 8.4 m deep. Earth taken out of it is spread all around it to a width of 7.5 m to form an embankment. Find the height of the embankment.
Vertical cross-section of a right circular cylinder is always a
Two steel sheets each of length a1 and breadth a2 are used to prepare the surfaces of two right circular cylinders ⇀ one having volume v1 and height a2 and other having volume v2 and height a1. Then,
Find the area of base and radius of a cylinder if its curved surface area is 660 sqcm and height is 21 cm.
A cylindrical container with internal diameter of its base 20 cm, contains water upto a height of 14 cm. Find the area of the wet surface of the cylinder.
The radius and height of a cylinder are in the ratio 5:7 and its curved surface area is 5500 sq.cm. Find its radius and height.
In the above question, curved surface area of A is ______ curved surface area of B.
In the example given below, the radius of the base of a cylinder and its height is given. Then find the curved surface area and total surface area.
r = 70 cm, h = 1.4 cm
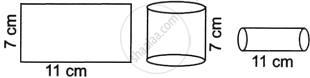
A rectangular sheet of paper of size 11 cm × 7 cm is first rotated about the side 11 cm and then about the side 7 cm to form a cylinder, as shown in the diagram. The ratio of their curved surface areas is ______.