Topics
Compound Interest
- Compound Interest as a Repeated Simple Interest Computation with a Growing Principal
- Use of Compound Interest in Computing Amount Over a Period of 2 Or 3-years
- Use of Formula
- Finding CI from the Relation CI = A – P
GST (Goods and Services Tax)
- Sales Tax, Value Added Tax, and Good and Services Tax
- Computation of Tax
- Concept of Discount
- List Price
- Basic Concepts of Profit and Loss
- Basic/Cost Price Including Inverse Cases.
- Selling Price
- Dealer
- Goods and Service Tax (Gst)
- Gst Tax Calculation
- Input Tax Credit (Itc)
Commercial Mathematics
Algebra
Banking
Geometry
Shares and Dividends
Linear Inequations
- Linear Inequations in One Variable
- Solving Algebraically and Writing the Solution in Set Notation Form
- Representation of Solution on the Number Line
Mensuration
- Circumference of a Circle
- Surface Area of a Right Circular Cone
- Surface Area of a Sphere
- Circle - Direct Application Problems Including Inner and Outer Area
- Surface Area of a Right Circular Cone
- Surface Area of a Sphere
- Volume of a Cylinder
- Volume of a Combination of Solids
- Surface Area of Cylinder
Symmetry
Quadratic Equations
- Quadratic Equations
- Solutions of Quadratic Equations by Factorization
- Nature of Roots of a Quadratic Equation
- Equations Reducible to Quadratic Equations
Trigonometry
- Trigonometric Ratios of Complementary Angles
- Trigonometric Identities
- Heights and Distances - Solving 2-D Problems Involving Angles of Elevation and Depression Using Trigonometric Tables
- Trigonometry
Statistics
- Median of Grouped Data
- Graphical Representation of Data as Histograms
- Ogives (Cumulative Frequency Graphs)
- Concepts of Statistics
- Graphical Representation of Data as Histograms
- Graphical Representation of Ogives
- Finding the Mode from the Histogram
- Finding the Mode from the Upper Quartile
- Finding the Mode from the Lower Quartile
- Finding the Median, upper quartile, lower quartile from the Ogive
- Calculation of Lower, Upper, Inter, Semi-Inter Quartile Range
- Concept of Median
- Mean of Grouped Data
- Mean of Ungrouped Data
- Median of Ungrouped Data
- Mode of Ungrouped Data
- Mode of Grouped Data
- Mean of Continuous Distribution
Solving (Simple) Problems (Based on Quadratic Equations)
- Problems Based on Numbers
- Problems Based on Time and Work
- Problems Based on Geometrical Figures
- Problems Based on Distance, Speed and Time
- Problems on C.P. and S.P.
- Miscellaneous Problems
Ratio and Proportion
- Concept of Ratio
- Concept of Proportion
- Componendo and Dividendo Properties
- Alternendo and Invertendo Properties
- Direct Applications
Probability
Factorization
- Factor Theorem
- Remainder Theorem
- Factorising a Polynomial Completely After Obtaining One Factor by Factor Theorem
Matrices
Arithmetic Progression
- Arithmetic Progression - Finding Their General Term
- Sum of First ‘n’ Terms of an Arithmetic Progressions
- Simple Applications of Arithmetic Progression
- Arithmetic mean
- Properties of an Arithmetic Progression
Geometric Progression
- Geometric Progression - Finding Their General Term.
- Geometric Progression - Finding Sum of Their First ‘N’ Terms
- Simple Applications - Geometric Progression
Reflection
- Reflection Examples
- Reflection Concept
- Reflection of a Point in a Line
- Reflection of a Point in the Origin.
- Invariant Points.
Co-ordinate Geometry Distance and Section Formula
- Co-ordinates Expressed as (x,y)
- Distance Formula
- Section Formula
- The Mid-point of a Line Segment (Mid-point Formula)
- Points of Trisection
- Centroid of a Triangle
Co-ordinate Geometry Equation of a Line
- Slope of a Line
- Concept of Slope
- Equation of a Line
- Various Forms of Straight Lines
- General Equation of a Line
- Slope – Intercept Form
- Two - Point Form
- Geometric Understanding of ‘m’ as Slope Or Gradient Or tanθ Where θ Is the Angle the Line Makes with the Positive Direction of the x Axis
- Geometric Understanding of c as the y-intercept Or the Ordinate of the Point Where the Line Intercepts the y Axis Or the Point on the Line Where x=0
- Conditions for Two Lines to Be Parallel Or Perpendicular
- Simple Applications of All Co-ordinate Geometry.
- Collinearity of Three Points
Similarity
- Similarity of Triangles
- Axioms of Similarity of Triangles
- Areas of Similar Triangles Are Proportional to the Squares on Corresponding Sides
- Conditions for Similarity of Two Triangles: (Sas, Aa Or Aaa and Sss)
- Basic Proportionality Theorem with Applications
- Relation Between the Areas of Two Triangles
- Similarity as a Size Transformation
- Direct Applications Based on the Above Including Applications to Maps and Models
Loci
Circles
- Concept of Circle
- Areas of Sector and Segment of a Circle
- Tangent Properties - If a Line Touches a Circle and from the Point of Contact, a Chord is Drawn, the Angles Between the Tangent and the Chord Are Respectively Equal to the Angles in the Corresponding Alternate Segments
- Tangent Properties - If a Chord and a Tangent Intersect Externally, Then the Product of the Lengths of Segments of the Chord is Equal to the Square of the Length of the Tangent from the Point of Contact to the Point of Intersection
- Tangent to a Circle
- Number of Tangents from a Point on a Circle
- Chord Properties - a Straight Line Drawn from the Center of a Circle to Bisect a Chord Which is Not a Diameter is at Right Angles to the Chord
- Chord Properties - the Perpendicular to a Chord from the Center Bisects the Chord (Without Proof)
- Theorem: Equal chords of a circle are equidistant from the centre.
- Theorem : The Chords of a Circle Which Are Equidistant from the Centre Are Equal.
- Chord Properties - There is One and Only One Circle that Passes Through Three Given Points Not in a Straight Line
- Arc and Chord Properties - the Angle that an Arc of a Circle Subtends at the Center is Double that Which It Subtends at Any Point on the Remaining Part of the Circle
- Theorem: Angles in the Same Segment of a Circle Are Equal.
- Arc and Chord Properties - Angle in a Semi-circle is a Right Angle
- Arc and Chord Properties - If Two Arcs Subtend Equal Angles at the Center, They Are Equal, and Its Converse
- Arc and Chord Properties - If Two Chords Are Equal, They Cut off Equal Arcs, and Its Converse (Without Proof)
- Arc and Chord Properties - If Two Chords Intersect Internally Or Externally Then the Product of the Lengths of the Segments Are Equal
- Cyclic Properties
- Tangent Properties - If Two Circles Touch, the Point of Contact Lies on the Straight Line Joining Their Centers
Constructions
- Circumscribing and Inscribing a Circle on a Regular Hexagon
- Circumscribing and Inscribing a Circle on a Triangle
- Construction of Tangents to a Circle
- Circumference of a Circle
- Circumscribing and Inscribing Circle on a Quadrilateral
- Right Circular Cylinder
- Hollow Cylinder
Definition
- Right Circular Cylinder: Right Circular Cylinder is a solid geometrical figure with straight parallel sides and a circular or oval cross-section.
Formula
- Curved Surface Area of a Cylinder = 2πrh
- Total Surface Area of a Cylinder = 2πr(r + h)
Notes
Right Circular Cylinder:
Right Circular Cylinder is a solid geometrical figure with straight parallel sides and a circular or oval cross-section.
Curved surface area of the cylinder:
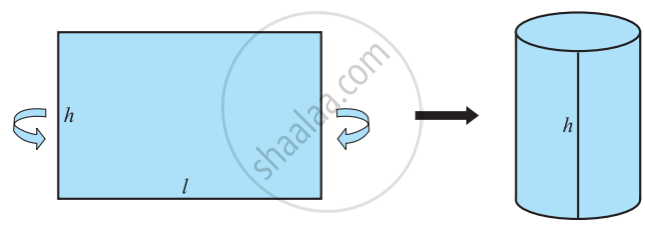
The area of the sheet gives us the curved surface area of the cylinder. Note that the length of the sheet is equal to the circumference of the circular base which is equal to 2πr.
So,
∴ Curved surface area of the cylinder = area of the rectangular sheet = length × breadth
= perimeter of the base of the cylinder × h
= 2πr × h
Therefore, Curved Surface Area of a Cylinder = 2πrh, where r is the radius of the base of the cylinder and h is the height of the cylinder.
Total Surface Area of a Cylinder:
If the top and the bottom of the cylinder are also to be covered, then we need two circles (in fact, circular regions) to do that, each of radius r, and thus having an area of πr 2 each, giving us the total surface area as 2πrh + 2πr 2 = 2πr(r + h).
So, Total Surface Area of a Cylinder = 2πr(r + h), where h is the height of the cylinder and r its radius.
Example
In a building, there are 24 cylindrical pillars. For each pillar, the radius is 28 m, and the height is 4 m. Find the total cost of painting the curved surface area of the pillars at the rate of ₹ 8 per m2.
Radius of cylindrical pillar, r = 28 cm = 0.28 m, height = h = 4 m
curved surface area of a cylinder = 2πrh
curved surface area of a pillar = `2 xx 22/7 xx 0.28 xx 4` = 7.04 m2
curved surface area of 24 such pillar = 7.04 × 24 = 168.96 m2
cost of painting an area of 1 m2 = Rs. 8
Therefore, cost of painting 1689.6 m2 = 168.96 × 8 = Rs. 1351.68.
Example
Find the height of a cylinder whose radius is 7 cm and the total surface area is 968 cm2.
Let height of the cylinder = h, radius = r = 7cm
Total surface area = 2πr(h + r)
i.e., 2 × `22/7` × 7 × (7 + h) = 968
h = 15 cm
Hence, the height of the cylinder is 15 cm.
If you would like to contribute notes or other learning material, please submit them using the button below.
Shaalaa.com | Total Surface Area & Lateral Surface Area of a Cylinder
to track your progress
Series: Surface Area of Cylinder
0%
