Advertisements
Advertisements
Question
Find the slope of the line which is parallel to `x/2 - y/3 -1 = 0 `
Solution
`x/2 - y/3 -1 = 0 `
`y/3 = x/2 -1 `
`y = (3x)/2 - 3`
Slope of this line = `3/2`
Slope of the line which is parallel to the given line = Slope of the given line = `3/2`
APPEARS IN
RELATED QUESTIONS
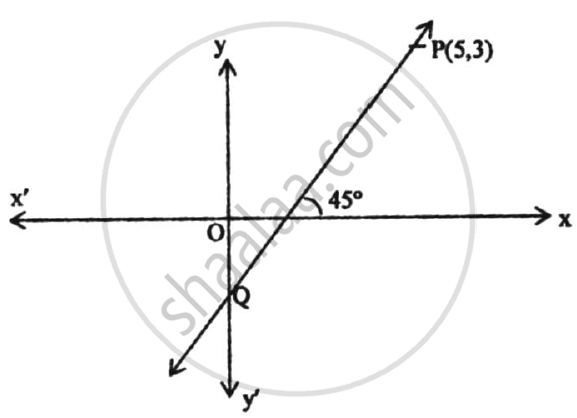
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
A(5, 4), B(−3, −2) and C(1, −8) are the vertices of a triangle ABC. Find:
- the slope of the altitude of AB,
- the slope of the median AD and
- the slope of the line parallel to AC.
Find the value of k for which the lines kx – 5y + 4 = 0 and 5x – 2y + 5 = 0 are perpendicular to each other.
Determine whether the given point is collinear.
\[P\left( 1, 2 \right), Q\left( 2, \frac{8}{5} \right), R\left( 3, \frac{6}{5} \right)\]
Find the slope of a line passing through the given pair of points (2,5) and (-1,8)
Find the slope of a line parallel to the given line x +3y = 7
Find the slope of a line passing through the points (x, 9) and (12, 6) is `(-1)/3 = ("y"_2 - "y"_1)/("x"_2 - "x"_1)`
Find m if the slope of the line passing through the point (-7,5) and (2,m) is `1/3`
If the lines 7y = ax + 4 and 2y = 3 − x, are parallel to each other, then the value of ‘a’ is:
