Advertisements
Advertisements
Question
Find the volume of the solid generated by the complete revolution of the ellipse `"x"^2/36 + "y"^2/25 = 1` about Y-axis.
Solution
The equation of the ellipse is
`"x"^2/36 + "y"^2/25 = 1`
i.e. `"x"^2/36 = 1 - "y"^2/25 `
`therefore "x"^2 = 36/25 (25 - "y"^2)`
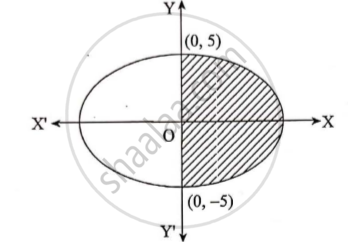
Let V be the required volume of the. solid obtained by revolving the ellipse about Y-axis.
`therefore "V" = pi int_-5^5 "x"^2 "dy"`
`= pi int_-5^5 36/25 (25 - "y"^2) "dy"`
`= 36/25 xx pi xx 2 int _0^5 (25 - "y"^2) "dy"`
....`[because int_(-"a")^"a" "f(x)" "dx" = 2 int_0^"a" "f(x)" "dx"]`
`= (72 pi)/25 [25"y" - "y"^3/3]_0^5`
`= (72 pi)/25 [25(5) - 5^3/3 - 0]`
`= (72 pi)/25 [125 - 125/3] = (72 pi)/25 [250/3]`
= 240 π cubic units.
APPEARS IN
RELATED QUESTIONS
Find the elasticity of demand if the marginal revenue is ₹ 50 and price is ₹ 75.
Evaluate : `int _0^1 ("x" . ("sin"^-1 "x")^2)/sqrt (1 - "x"^2)` dx
Integrate the following functions with respect to x :
`(x^3 + 4x^2 - 3x + 2)/x^2`
Integrate the following functions with respect to x :
`(sin^2x)/(1 + cosx)`
Integrate the following with respect to x :
`x^2/(1 + x^6)`
Integrate the following with respect to x :
`tan x sqrt(sec x)`
Integrate the following with respect to x :
sin5x cos3x
Integrate the following with respect to x:
25xe–5x
Integrate the following with respect to x:
x log x
Integrate the following with respect to x:
27x2e3x
Integrate the following with respect to x:
x3 sin x
Integrate the following with respect to x:
`"e"^(2x) sinx`
Integrate the following with respect to x:\
`logx/(1 + log)^2`
Find the integrals of the following:
`1/sqrt(xx^2 + 4x + 2)`
Integrate the following with respect to x:
`(2x - 3)/(x^2 + 4x - 12)`
Choose the correct alternative:
The gradient (slope) of a curve at any point (x, y) is `(x^2 - 4)/x^2`. If the curve passes through the point (2, 7), then the equation of the curve is
Choose the correct alternative:
`int ("e"^x (1 + x))/(cos^2(x"e"^x)) "d"x` is
Choose the correct alternative:
`int 1/(x sqrt(log x)^2 - 5) "d"x` is
