Advertisements
Advertisements
Question
Find the total surface area of a right circular cone with radius 6 cm and height 8 cm.
Solution
WKT, Total surface area = `pirl+pir^2`
Now l= `sqrth(^2+r^2)` [by Pythagoras theorem]
Here, given
Radius=6cm and height =8cm
⇒ length=`sqrt(6^2+8^2)`
= 10cm
∴ Total surface area= `pirl+pir^2`
=`(22/7xx6xx10)+ (22/7xx6xx6)`
= `(1320/7)+792/7 =301.71cm^2`
APPEARS IN
RELATED QUESTIONS
Find the curved surface area of a cone with base radius 5.25 cm and slant height 10cm.
Find the ratio of the curved surface areas of two cones if their diameters of the bases are equal and slant heights are in the ratio 4 : 3.
A heap of wheat is in the form of a cone of diameter 9 m and height 3.5 m. Find its volume. How much canvas cloth is required to just cover the heap? (Use 𝜋 = 3.14).
The curved surface area of a cone is 12320 cm2. If the radius of its base is 56 cm, find its height.
The area of the base of a conical solid is 38.5 cm2 and its volume is 154 cm3. Find the curved surface area of the solid.
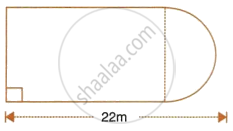
In the following diagram a rectangular platform with a semi-circular end on one side is 22 metres long from one end to the other end. If the length of the half circumference is 11 metres, find the cost of constructing the platform, 1.5 metres high at the rate of Rs. 4 per cubic metres.
The heights of two cones are in the ratio 1:3 and their base radii are in the ratio 3:1. Find the ratio of their volumes.
A buoy is made in the form of a hemisphere surmounted by a right circular cone whose circular base coincides with the plane surface of the hemisphere. The radius of the base of the cone is 3.5 m and its volume is two-third the volume of hemisphere. Calculate the height of the cone and the surface area of the buoy, correct to two decimal places.
A hollow metallic cylindrical tube has an internal radius of 3.5 cm and height 21 cm. The thickness of the metal tube is 0.5 cm. The tube is melted and cast into a right circular cone of height 7 cm. Find the radius of the cone, correct to one decimal place.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate the: height of the tent.
