Advertisements
Advertisements
Question
Find the value of a line parallel to the following line:
`(3"y")/4 + (5"y")/2 = 7`
Solution
`(3"y")/4 + (5"y")/2 = 7`
10y = -3x + 28
y = `-3/10"x" + 14/5`
m1 = `-3/10`
Slope of required line (m2) = m1 = `-3/10`
APPEARS IN
RELATED QUESTIONS
If the straight lines 3x – 5y = 7 and 4x + ay + 9 = 0 are perpendicular to one another, find the value of a.
A(2, 5), B(–1, 2) and C(5, 8) are the vertices of a triangle ABC, `M' is a point on AB such that AM: MB = 1: 2. Find the coordinates of 'M'. Hence find the equation of the line passing through the points C and M
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(2, –1.5)
The point P divides the join of (2, 1) and (−3, 6) in the ratio 2 : 3. Does P lies on the line x − 5y + 15 = 0?
Find the equation of the line passing through : (−1, −4) and (3, 0)
The line through P (5, 3) intersects y-axis at Q.
Find the co-ordinates of Q.
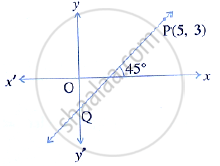
Find if the following points lie on the given line or not:
(5,3) on the line 3x - 5y + 5 = 0
Find the inclination of a line whose gradient is 1.4281
Find the equation of a line passing through (3,7) and making an angle of 60° with the negative direction of the x-axis.
Find the equations of a line passing through the point (2, 3) and having the x – interecpt of 4 units.
