Advertisements
Advertisements
Question
Five charges, q each are placed at the corners of a regular pentagon of side ‘a’ (Figure).
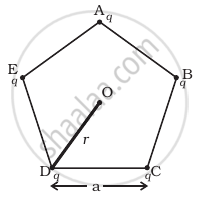
(a) (i) What will be the electric field at O, the centre of the pentagon?
(ii) What will be the electric field at O if the charge from one of the corners (say A) is removed?
(iii) What will be the electric field at O if the charge q at A is replaced by –q?
(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its corners?
Solution
(a) (i) The point O, the centre of the pentagon is equidistant from all the charges at the end point of pentagon. Thus, due to symmetry, the electric field due to all the charges is cancelled out. As a result, electric field at O is zero.
(ii) We can write that the vector sum of electric field due to charge A and electric field due to the other four charges at the centre of cube should be zero or, `vecE_A + vecE_("four charges") = 0`
Hence `vecE_("four charges") = - vecE_A` or ⇒ `|vecE_("four charges")| = |vecE_A|`
When charge q is removed from A, net electric field at the centre due to remaining charges `|vecE_("four charge")| = |vecE_A| = 1/(4 piε_0) q/r^2` along OA.
(iii) If charge q at A is replaced by –q, then electric field due to this negative charge
`vecE_(-q) = 1/(4 piε_0) q/r^2` along OA.
HEnce net electric field at the centre
`vecE_("net") = vecE_(-q) + vecE_("four charges") = 1/(4 pi ε_0) q/r^2 + 1/(4 pi ε_0) q/r^2`
`vecE_("net") = 1/(4 pi ε_0) (2q)/r^2` along OA.
(b) If pentagon is replaced by n-sided regular polygon with charge q at each of its comers. Here again, charges are symmetrical about the centre. The net electric field at O would continue to be zero, it doesn’t depend on the number of sides or the number of charges.
APPEARS IN
RELATED QUESTIONS
The electric field at the origin is along the positive x-axis. A small circle is drawn with the centre at the origin, cutting the axes at points A, B, C and D with coordinates (a, 0), (0, a), (−a, 0), (0, −a), respectively. Out of the points on the periphery of the circle, the potential is minimum at
If a body is charged by rubbing it, its weight
Consider the situation in the figure. The work done in taking a point charge from P to Ais WA, from P to B is WB and from P to C is WC.
Electric potential decreases uniformly from 120 V to 80 V, as one moves on the x-axis from x = −1 cm to x = +1 cm. The electric field at the origin
(a) must be equal to 20 Vcm−1
(b) may be equal to 20 Vcm−1
(c) may be greater than 20 Vcm−1
(d) may be less than 20 Vcm−1
The electric field in a region is directed outward and is proportional to the distance rfrom the origin. Taking the electric potential at the origin to be zero,
Consider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
An electric field of 20 NC−1 exists along the x-axis in space. Calculate the potential difference VB − VA where the points A and B are
(a) A = (0, 0); B = (4 m, 2m)
(b) A = (4 m, 2 m); B = (6 m, 5 m)
(c) A = (0, 0); B = (6 m, 5 m)
Do you find any relation between the answers of parts (a), (b) and (c)?
Find the magnitude of the electric field at the point P in the configuration shown in the figure for d >> a.
In general, metallic ropes are suspended on the carriers taking inflammable materials. The reason is ______.
The electric field intensity produced by the radiations coming from 100 W bulb at 3 m distance is E. The electric field intensity produced by the radiations coming from 50 W bulb at the same distance is:
