Advertisements
Advertisements
प्रश्न
Five charges, q each are placed at the corners of a regular pentagon of side ‘a’ (Figure).
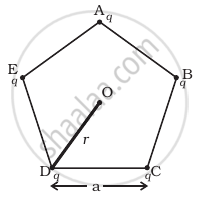
(a) (i) What will be the electric field at O, the centre of the pentagon?
(ii) What will be the electric field at O if the charge from one of the corners (say A) is removed?
(iii) What will be the electric field at O if the charge q at A is replaced by –q?
(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its corners?
Advertisements
उत्तर
(a) (i) The point O, the centre of the pentagon is equidistant from all the charges at the end point of pentagon. Thus, due to symmetry, the electric field due to all the charges is cancelled out. As a result, electric field at O is zero.
(ii) We can write that the vector sum of electric field due to charge A and electric field due to the other four charges at the centre of cube should be zero or, `vecE_A + vecE_("four charges") = 0`
Hence `vecE_("four charges") = - vecE_A` or ⇒ `|vecE_("four charges")| = |vecE_A|`
When charge q is removed from A, net electric field at the centre due to remaining charges `|vecE_("four charge")| = |vecE_A| = 1/(4 piε_0) q/r^2` along OA.
(iii) If charge q at A is replaced by –q, then electric field due to this negative charge
`vecE_(-q) = 1/(4 piε_0) q/r^2` along OA.
HEnce net electric field at the centre
`vecE_("net") = vecE_(-q) + vecE_("four charges") = 1/(4 pi ε_0) q/r^2 + 1/(4 pi ε_0) q/r^2`
`vecE_("net") = 1/(4 pi ε_0) (2q)/r^2` along OA.
(b) If pentagon is replaced by n-sided regular polygon with charge q at each of its comers. Here again, charges are symmetrical about the centre. The net electric field at O would continue to be zero, it doesn’t depend on the number of sides or the number of charges.
APPEARS IN
संबंधित प्रश्न
The figure shows tracks of three charged particles in a uniform electrostatic field. Give the signs of the three charges. Which particle has the highest charge to mass ratio?
Consider a system of n charges q1, q2, ... qn with position vectors `vecr_1,vecr_2,vecr_3,...... vecr_n`relative to some origin 'O'. Deduce the expression for the net electric field`vec E` at a point P with position vector `vecr_p,`due to this system of charges.
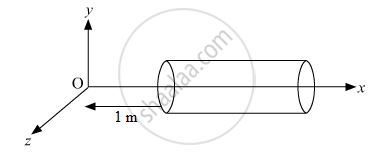
A hollow cylindrical box of length 1 m and area of cross-section 25 cm2 is placed in a three dimensional coordinate system as shown in the figure. The electric field in the region is given by `vecE = 50xhati` where E is NC−1 and x is in metres. Find
(i) Net flux through the cylinder.
(ii) Charge enclosed by the cylinder.
A hollow cylindrical box of length 0.5 m and area of cross-section 25 cm2 is placed in a three dimensional coordinate system as shown in the figure. The electric field in the region is given by `vecE = 20 xhati` where E is NC−1 and x is in metres. Find
(i) Net flux through the cylinder.
(ii) Charge enclosed by the cylinder.
Why does a phonograph record attract dust particles just after it is cleaned?
Consider the situation in the figure. The work done in taking a point charge from P to Ais WA, from P to B is WB and from P to C is WC.
A point charge q is rotated along a circle in an electric field generated by another point charge Q. The work done by the electric field on the rotating charge in one complete revolution is
The electric field and the electric potential at a point are E and V, respectively.
Which of the following quantities does not depend on the choice of zero potential or zero potential energy?
A particle of mass m and charge q is thrown at a speed u against a uniform electric field E. How much distance will it travel before coming to momentary rest ?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. What will be the speed of the particle after travelling this distance?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. How much is the work done by the electric force on the particle during this period?
12 J of work has to be done against an existing electric field to take a charge of 0.01 C from A to B. How much is the potential difference VB − VA?
Consider the situation of the previous problem. A charge of −2.0 × 10−4 C is moved from point A to point B. Find the change in electrical potential energy UB − UA for the cases (a), (b) and (c).
An electric field \[\vec{E} = ( \vec{i} 20 + \vec{j} 30) {NC}^{- 1}\] exists in space. If the potential at the origin is taken to be zero, find the potential at (2 m, 2 m).
Assume that each atom in a copper wire contributes one free electron. Estimate the number of free electrons in a copper wire of mass 6.4 g (take the atomic weight of copper to be 64 g mol−1).
