Advertisements
Advertisements
प्रश्न
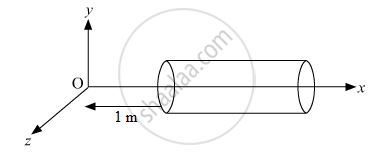
A hollow cylindrical box of length 1 m and area of cross-section 25 cm2 is placed in a three dimensional coordinate system as shown in the figure. The electric field in the region is given by `vecE = 50xhati` where E is NC−1 and x is in metres. Find
(i) Net flux through the cylinder.
(ii) Charge enclosed by the cylinder.
उत्तर

Given,`vecE = 50xhati and Δs = 25 cm^2 = 25 xx 10^-4 m^2`
As the electric field is only along the x-axis, so, flux will pass only through the cross-section of cylinder.
magnitude of electric field at cross - section A,`E_A = 50 xx 1 =50N C^-1`
magnitude of electric field at cross - section `B,E^B = 50 xx 2 = 100 N C^-1`
The corresponding electric fluxes are :
`ø_A = vecE.Δvecs = 50 xx 25 xx 10^-4 xx cos 180° = -0.125 N m^2 C^-1`
`ø_A = vecE.Δvecs = 100 xx 25 xx 10^-4 xx cos 0° = 0.25N m^2 C^-1`
So, the net flux through the cylinder,`ø =ø_A +ø_B = -0.125 + 0.25 = 0 . 125 N m^2C^-1 `
(ii) Using Gauss’s law: `ointvecE.dvecs =q/in_0⇒ 0.125 = q/(8.85 xx 10^-12)⇒ q=8.85 xx 0.125 xx 10^-12 =1.1 xx 10 ^-12 C `
संबंधित प्रश्न
Can a gravitational field be added vectorially to an electric field to get a total field?
Why does a phonograph record attract dust particles just after it is cleaned?
A point charge q is rotated along a circle in an electric field generated by another point charge Q. The work done by the electric field on the rotating charge in one complete revolution is
Consider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
A block of mass m with a charge q is placed on a smooth horizontal table and is connected to a wall through an unstressed spring of spring constant k, as shown in the figure. A horizontal electric field E, parallel to the spring, is switched on. Find the amplitude of the resulting SHM of the block. 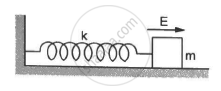
Consider the situation of the previous problem. A charge of −2.0 × 10−4 C is moved from point A to point B. Find the change in electrical potential energy UB − UA for the cases (a), (b) and (c).
The electric potential existing in space is \[\hspace{0.167em} V(x, y, z) = A(xy + yz + zx) .\] (a) Write the dimensional formula of A. (b) Find the expression for the electric field. (c) If A is 10 SI units, find the magnitude of the electric field at (1 m, 1 m, 1 m).
In general, metallic ropes are suspended on the carriers taking inflammable materials. The reason is ______.
Two similar spheres having +Q and -Q charges are kept at a certain distance. F force acts between the two. If at the middle of two spheres, another similar sphere having +Q charge is kept, then it experiences a force in magnitude and direction as ______.
The Electric field at a point is ______.
- always continuous.
- continuous if there is no charge at that point.
- discontinuous only if there is a negative charge at that point.
- discontinuous if there is a charge at that point.
