Advertisements
Advertisements
प्रश्न
Consider the situation of the previous problem. A charge of −2.0 × 10−4 C is moved from point A to point B. Find the change in electrical potential energy UB − UA for the cases (a), (b) and (c).
उत्तर
Given:
Magnitude of charge, q = −2.0 × 10−4 C
(a) The electric field is along the x direction.
Thus, potential difference between (0, 0) and (4, 2),
dV = −E.dx = −20 × 4 = −80 V
Potential energy (UB − UA) between the points = dV × q
⇒ UB − UA = (−80) × (−2.0 × 10−4)
⇒ UB − UA = 160 × 10−4 = 0.016 J
(b) A = (4 m, 2m), B = (6 m, 5 m)
dV = −E.dx = − 20 × 2 = −40 V
Potential energy (UB − UA) between the points = dV × q
⇒ UB − UA = (−40) × (−2 × 10−4)
⇒ UB − UA = 80 × 10−4 = 0.008 J
(c) A = (0, 0) B = (6m, 5m)
dV = −E.dx = −20 × 6 = −120 V
Potential energy (UB − UA) between the points A and B = dV × q
⇒ UB − UA = (−120) × (−2 × 10−4)
⇒ UB − UA = 240 × 10−4 = 0.024 J
APPEARS IN
संबंधित प्रश्न
Consider a system of n charges q1, q2, ... qn with position vectors `vecr_1,vecr_2,vecr_3,...... vecr_n`relative to some origin 'O'. Deduce the expression for the net electric field`vec E` at a point P with position vector `vecr_p,`due to this system of charges.
Why does a phonograph record attract dust particles just after it is cleaned?
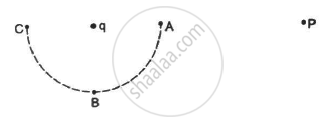
Consider the situation in the figure. The work done in taking a point charge from P to Ais WA, from P to B is WB and from P to C is WC.
The electric field and the electric potential at a point are E and V, respectively.
Which of the following quantities does not depend on the choice of zero potential or zero potential energy?
Consider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
A particle of mass m and charge q is thrown at a speed u against a uniform electric field E. How much distance will it travel before coming to momentary rest ?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. Find the electric force and the force of gravity acting on this particle. Can one of these forces be neglected in comparison with the other for approximate analysis?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. How long will it take for the particle to travel a distance of 40 cm?
An electric field of 20 NC−1 exists along the x-axis in space. Calculate the potential difference VB − VA where the points A and B are
(a) A = (0, 0); B = (4 m, 2m)
(b) A = (4 m, 2 m); B = (6 m, 5 m)
(c) A = (0, 0); B = (6 m, 5 m)
Do you find any relation between the answers of parts (a), (b) and (c)?
An electric field \[\vec{E} = \vec{i}\] Ax exists in space, where A = 10 V m−2. Take the potential at (10 m, 20 m) to be zero. Find the potential at the origin.
The kinetic energy of a charged particle decreases by 10 J as it moves from a point at potential 100 V to a point at potential 200 V. Find the charge on the particle.
Find the magnitude of the electric field at the point P in the configuration shown in the figure for d >> a.
Two identical blocks are kept on a frictionless horizontal table connected by a spring of stiffness k and of original length l0. A total charge Q is distributed on the block such that maximum elongation of spring at equilibrium is equal to x. Value of Q is ______.
The Electric field at a point is ______.
- always continuous.
- continuous if there is no charge at that point.
- discontinuous only if there is a negative charge at that point.
- discontinuous if there is a charge at that point.
Five charges, q each are placed at the corners of a regular pentagon of side ‘a’ (Figure).
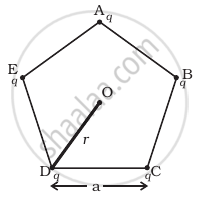
(a) (i) What will be the electric field at O, the centre of the pentagon?
(ii) What will be the electric field at O if the charge from one of the corners (say A) is removed?
(iii) What will be the electric field at O if the charge q at A is replaced by –q?
(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its corners?
