Advertisements
Advertisements
Question
For any prism, prove that :
'n' or `mu = sin((A + delta_m)/2)/sin(A/2)`
where the terms have their usual meaning
Solution
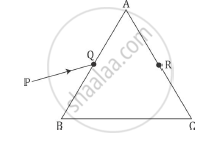
In the figure, a ray of light PQ is incident at an angle i on the face AB of prism ABC. This ray is
refracted along QR at an angle r. This reflected ray is incident on the face AC at an angle r ' and
emerges along RS at an angle i '.
In ΔQDR
`delta = (1 - r )+ (i' + r')`
`= (i + i') - (r + r')` ....(i)

In Quad. AQER, A + E = 180° ...(ii)
In ΔQER r + r' + E = 180° ...(iii)
r + r' = A [From eq ii and iii]
Putting value r + r' inequation (i)
`delta = i + i' - A`
In the position of minimum deviation condition
`i = i', r = r', delta = delta_m`
So r + r' = A
2r = A
or `r = A/2`
`delta_m = 2i - A`
`i = (A + delta_m)/2`
Putting value of i and r from (v), (vi), in Snell’s law,
`n = sini/sin r`
`m = (sin ((A+delta_m)/2))/(sin (A/2))`
APPEARS IN
RELATED QUESTIONS
A ray PQ incident on the face AB of a prism ABC, as shown in the figure, emerges from the face AC such that AQ = AR.
Draw the ray diagram showing the passage of the ray through the prism. If the angle of the prism is 60° and refractive index of the material of prism is `sqrt3` , determine the values of angle of incidence and angle of deviation
State any two difference between the primary rainbow and secondary rainbow
Out of blue and red light which is deviated more by a prism? Give reason.
If a glass prism is dipped in water, its dispersive power ___________ .
A certain material has refractive indices 1.56, 1.60 and 1.68 rfor red, yellow and violet lightespectively. (a) Calculate the dispersive power. (b) Find the angular dispersion produced by a thin prism of angle 6° made of this material.
Two prisms of identical geometrical shape are combined with their refracting angles oppositely directed. The materials of the prisms have refractive indices 1.52 and 1.62 for violet light. A violet ray is deviated by 1.0° when passes symmetrically through this combination. What is the angle of the prisms?
The refractive index of a material M1 changes by 0.014 and that of another material M2 changes by 0.024 as the colour of the light is changed from red to violet. Two thin prisms, one made of M1(A = 5.3°) and the other made of M2(A = 3.7°) are combined with their refracting angles oppositely directed. (a) Find the angular dispersion produced by the combination. (b) The prisms are now combined with their refracting angles similarly directed. Find the angular dispersion produced by the combination.
The deviation produced for violet, yellow and red lights for crown glass are 3.75°, 3.25° and 2.86° respectively. Calculate the dispersive power of the crown glass.
A ray of light is incident on a prism whose refractive index is 1.52 at an angle of 40°. If the angle of emergence is 60°, calculate the angle of the prism.
The refractive indices of material for red, violet and yellow colour light are 1.52, 1.62 and 1.59 respectively.
Calculate the dispersive power of the material. If the mean deviation is 40°. What will be the angular dispersion produced by a prism of this material?
