Advertisements
Advertisements
प्रश्न
For any prism, prove that :
'n' or `mu = sin((A + delta_m)/2)/sin(A/2)`
where the terms have their usual meaning
उत्तर
In the figure, a ray of light PQ is incident at an angle i on the face AB of prism ABC. This ray is
refracted along QR at an angle r. This reflected ray is incident on the face AC at an angle r ' and
emerges along RS at an angle i '.
In ΔQDR
`delta = (1 - r )+ (i' + r')`
`= (i + i') - (r + r')` ....(i)

In Quad. AQER, A + E = 180° ...(ii)
In ΔQER r + r' + E = 180° ...(iii)
r + r' = A [From eq ii and iii]
Putting value r + r' inequation (i)
`delta = i + i' - A`
In the position of minimum deviation condition
`i = i', r = r', delta = delta_m`
So r + r' = A
2r = A
or `r = A/2`
`delta_m = 2i - A`
`i = (A + delta_m)/2`
Putting value of i and r from (v), (vi), in Snell’s law,
`n = sini/sin r`
`m = (sin ((A+delta_m)/2))/(sin (A/2))`
APPEARS IN
संबंधित प्रश्न
Plot a graph to show the variation of the angle of deviation as a function of the angle of incidence for light passing through a prism. Derive an expression for the refractive index of the prism in terms of angle of minimum deviation and angle of the prism.
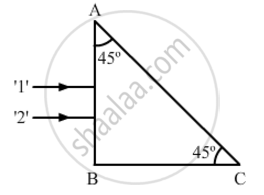
Two monochromatic rays of light are incident normally on the face AB of an isosceles right-angled prism ABC. The refractive indices of the glass prism for the two rays '1' and '2' are respectively 1.38 and 1.52. Trace the path of these rays after entering through the prism.
Draw the ray diagram showing refraction of light through a glass prism and hence obtain the relation between the refractive index μ of the prism, angle of prism and angle of minimum deviation.
If a glass prism is dipped in water, its dispersive power ___________ .
A thin prism is made of a material having refractive indices 1.61 and 1.65 for red and violet light. The dispersive power of the material is 0.07. It is found that a beam of yellow light passing through the prism suffers a minimum deviation of 4.0° in favourable conditions. Calculate the angle of the prism.
Three thin prisms are combined as shown in figure. The refractive indices of the crown glass for red, yellow and violet rays are μr, μy and μv respectively and those for the flint glass are μ'r, μ'y and μ'v respectively. Find the ratio A'/A for which (a) there is no net angular dispersion, and (b) there is no net deviation in the yellow ray.
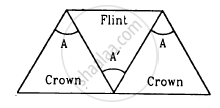
A thin prism of crown glass (μr = 1.515, μv = 1.525) and a thin prism of flint glass (μr = 1.612, μv = 1.632) are placed in contact with each other. Their refracting angles are 5.0° each and are similarly directed. Calculate the angular dispersion produced by the combination.
A thin prism of angle 6.0°, ω = 0.07 and μy = 1.50 is combined with another thin prism having ω = 0.08 and μy = 1.60. The combination produces no deviation in the mean ray. (a) Find the angle of the second prism. (b) Find the net angular dispersion produced by the combination when a beam of white light passes through it. (c) If the prisms are similarly directed, what will be the deviation in the mean ray? (d) Find the angular dispersion in the situation described in (c).
How does the angle of minimum deviation of a glass prism vary if the incident violet light is replaced by red light?
Prove that in case of a prism, i + e = A + δ, where the symbols have their usual meanings.
