Advertisements
Advertisements
प्रश्न
Draw the ray diagram showing refraction of light through a glass prism and hence obtain the relation between the refractive index μ of the prism, angle of prism and angle of minimum deviation.
उत्तर
The figure below shows the passage of light through a triangular prism ABC.
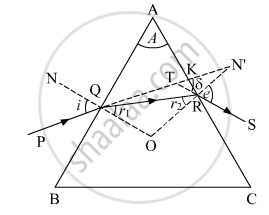
The angles of incidence and refraction at first face AB are ∠i and ∠r1.
The angle of incidence at the second face AC is ∠r2 and the angle of emergence ∠e.
δ is the angle between the emergent ray RS and incident ray PQ and is called the angle of deviation.
Here, ∠PQN = i
∠SRN'=e
∠RQO = r1
∠QRO = r2
∠KTS = δ
∴ ∠TQO = i and ∠RQO = r1, we have
∠TQR = i − r1
∠TRO = e and ∠QRO = r2
∠TRQ = e − r2
In triangle TQR, the side QT has been produced outwards. Therefore, the exterior angle δ should be equal to the sum of the interior opposite angles.
i.e, δ= ∠TQR + ∠TRQ = (i − r1) + (e − r2)
δ = (i + e) − (r1+ r2) ..…(i)
In triangle QRO,
r1+ r2+ ∠ROQ = 180° ..…(ii)
From quadrilateral AROQ, we have the sum of angles (∠AQO + ∠ARO = 180°). This means that the sum of the remaining two angles should be 180°.
i.e , ∠A + ∠QOR = 180° [∠A is called the angle of prism]
From equations (i) and (ii),
r1+ r2 = A (iii)
Substituting (iii) in (i), we obtain
δ = (i + e) − A
\[A + \delta = i + e\]
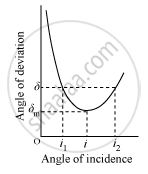
If the angle of incidence is increased gradually, then the angle of deviation first decreases, attains a minimum value (δm), and then again starts increasing.
When angle of deviation is minimum, the prism is said to be placed in the minimum deviation position.
There is only one angle of incidence for which the angle of deviation is minimum.
When
δ = δm [prism in minimum deviation position],
e = i and r2 = r1 = r …(iv)
\[\because r_1 + r_2 = A\]
From equation (iv), r + r = A
\[r = \frac{A}{2}\]
Also, we have
A + δ = i + e
Setting,
δ = δm and e = i
A + δm = i + i
\[i = \frac{\left( A + \delta_m \right)}{2}\]
\[ \because \mu = \frac{\sin i}{\sin r}\]
\[ \therefore \mu = \frac{\sin\left( \frac{A + \delta_m}{2} \right)}{\sin\left( \frac{A}{2} \right)}\]
APPEARS IN
संबंधित प्रश्न
Plot a graph to show the variation of the angle of deviation as a function of the angle of incidence for light passing through a prism. Derive an expression for the refractive index of the prism in terms of angle of minimum deviation and angle of the prism.
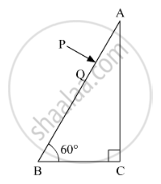
A ray PQ incident normally on the refracting face BA is refracted in the prism BAC made of material of refractive index 1.5. Complete the path of ray through the prism. From which face will the ray emerge? Justify your answer.
For any prism, prove that :
'n' or `mu = sin((A + delta_m)/2)/sin(A/2)`
where the terms have their usual meaning
Can the dispersive power \[\omega = \frac{\mu_u - \mu_r}{\mu - 1}\] be negative? What is the sign of ω if a hollow prism is immersed into water?
By properly combining two prisms made of different materials, it is possible to
(a) have dispersion without average deviation
(b) have deviation without dispersion
(c) have both dispersion and average deviation
(d) have neither dispersion nor average deviation
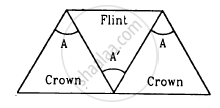
Three thin prisms are combined as shown in figure. The refractive indices of the crown glass for red, yellow and violet rays are μr, μy and μv respectively and those for the flint glass are μ'r, μ'y and μ'v respectively. Find the ratio A'/A for which (a) there is no net angular dispersion, and (b) there is no net deviation in the yellow ray.
The refractive index of a material M1 changes by 0.014 and that of another material M2 changes by 0.024 as the colour of the light is changed from red to violet. Two thin prisms, one made of M1(A = 5.3°) and the other made of M2(A = 3.7°) are combined with their refracting angles oppositely directed. (a) Find the angular dispersion produced by the combination. (b) The prisms are now combined with their refracting angles similarly directed. Find the angular dispersion produced by the combination.
The deviation produced for violet, yellow and red lights for crown glass are 3.75°, 3.25° and 2.86° respectively. Calculate the dispersive power of the crown glass.
In a regular prism, what is the relation between angle of incidence and angle of emergence when it is in the minimum deviation position?
Define angular dispersion.
