Advertisements
Advertisements
Question
For the given capacitor configuration
- Find the charges on each capacitor
- potential difference across them
- energy stored in each capacitor.
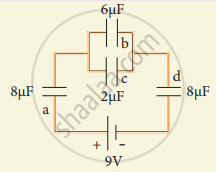
Solution
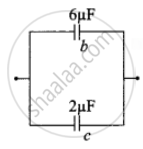
Capacitor b and c in parallel combination
Cp = Cb + Cc = (6 + 2) μF = 8 μF
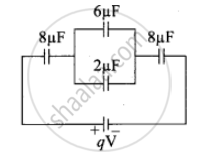
Capacitor a, cp and d are in series combination, so the resulatant copacitance
`1/"C"_"s" = 1/"C"_"a" + 1/"C"_"cp" + 1/"C"_"d"`
`= 1/8 + 1/8 + 1/8 = 3/8`
`"C"_"s" = 8/3` μF

- Charge on each capacitor,
Charge on capacitor a, Qa = Cs V = `8/3 xx 9`
Qa = 24 μC
Charge on capacitor, d, Qd = Cs V = `8/3 xx 9`
Qd = 24 μC
Capacitor b and c in parallel
Charge on capacitor, b, Qb = `6/3 xx 9` = 18
Qb = 18 μC
Charge on capacitor, c, Qc = `2/3 xx 9` = 6
Qc = 6 μC - Potential difference across each capacitor, V = `"q"/"c"`
Capacitor Ca, Va = `"q"_"a"/"C"_"a" = (24 xx 10^6)/(8 xx 10^6)` = 3 V
Capacitor Cb, Vb = `"q"_"b"/"C"_"b" = (18 xx 10^6)/(6 xx 10^6)` = 3 V
Capacitor Cc, Vc = `"q"_"c"/"C"_"c" = (6 xx 10^6)/(2 xx 10^6)` = 3 V
Capacitor Cd, Vd = `"q"_"d"/"C"_"d" = (24 xx 10^6)/(8 xx 10^6)` = 3 V - Energy stores in a capacitor, U = `1/2` CV2
Energy in capacitor Ca, Ua = `1/2 "C"_"a" "V"_"a"^2 = 1/2 xx 8 xx 10^-6 xx (3)^2`
Ua = 36 μJ
Capacitor Cb, Ub = `1/2 "C"_"b" "V"_"b"^2 = 1/2 xx 6 xx 10^-6 xx (3)^2`
Ua = 27 μJ
Cc, Uc = `1/2 "C"_"c" "V"_"c"^2 = 1/2 xx 2 xx 10^-6 xx (3)^2`
Ua = 9 μJ
Cd, Ud = `1/2 "C"_"d" "V"_"d"^2 = 1/2 xx 8 xx 10^-6 xx (3)^2`
Ua = 36 μJ
APPEARS IN
RELATED QUESTIONS
The outer cylinders of two cylindrical capacitors of capacitance 2⋅2 µF each, are kept in contact and the inner cylinders are connected through a wire. A battery of emf 10 V is connected as shown in figure . Find the total charge supplied by the battery to the inner cylinders.

Each of the plates shown in figure has surface area `(96/∈_0) xx 10^-12` Fm on one side and the separation between the consecutive plates is 4⋅0 mm. The emf of the battery connected is 10 volts. Find the magnitude of the charge supplied by the battery to each of the plates connected to it.

A 5⋅0 µF capacitor is charged to 12 V. The positive plate of this capacitor is now connected to the negative terminal of a 12 V battery and vice versa. Calculate the heat developed in the connecting wires.
Derive the expression for resultant capacitance, when the capacitor is connected in series.
A capacitor is charged by a battery. The battery is removed and another identical uncharged capacitor is connected in parallel. The total electrostatic energy of resulting system ______.
The work done in placing a charge of 8 × 10–18 coulomb on a condenser of capacity 100 micro-farad is ______.
The capacitance of a parallel plate capacitor is 60 µF. If the distance between the plates is tripled and area doubled then new capacitance will be ______.
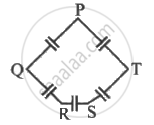
Five capacitor each of capacitance value C are connected as shown in the figure. The ratio of capacitance between P to R, and the capacitance between P and Q is ______.
A 5µF capacitor is charged fully by a 220 V supply. It is then disconnected from the supply and is connected in series to another uncharged 2.5 µF capacitor If the energy change during the charge redistribution is `"X"/100`J then value of X to the 100 nearest integer is ______.
