Advertisements
Advertisements
Question
For the sectors with given measures, find the length of the arc, area and perimeter. (π = 3.14)
central angle 45°, r = 16 cm
Solution
central angle 45°, r = 16 cm
Length of the arc l = `theta^circ/(360^circ) xx 2pi"r" "units"`
l = `(45^circ)/(360^circ) xx 2 xx 3.14 xx 16 "cm"`
l = `1/8 xx 2 xx 3.14 xx 16 "cm"`
l = 12.56 cm
Area of the sector = `theta^circ/(360^circ) xx pi"r"^2 "sq. units"`
A = `(45^circ)/(360^circ) xx 3.14 xx 16 xx 16`
A = 100.48 cm2
Perimeter of the sector P = l + 2r units
P = 12.56 + 2(16) cm
P = 44.56 cm
APPEARS IN
RELATED QUESTIONS

In the given figure, square ABCD is inscribed in the sector A - PCQ. The radius of sector C - BXD is 20 cm. Complete the following activity to find the area of shaded region
In Δ ABC, if ∠ A = 65° ; ∠ B = 40° then find the measure of ∠ C.
Length of a rectangular tank is twice its breadth. If the
depth of the tank is 3 m and area of its four walls is 108 m2, find the
length of the tank.
Match the following:
| Column A | Column B |
| (i) Area of a circle | (a) `1/4 pi"r"^2` |
| (ii) Circumference of a circle | (b) (π + 2)r |
| (iii) Area of the sector of a circle | (c) πr2 |
| (iv) Circumference of a semicircle | (d) 2πr |
| (v) Area of a quadrant of a circle | (e) `theta^circ/360^circ xx pi"r"^2` |
For the sectors with given measures, find the length of the arc, area and perimeter. (π = 3.14)
central angle 120°, d = 12.6 cm
A circle of radius 120 m is divided into 8 equal sectors. Find the length of the arc of each of the sectors
Two gates are fitted at the entrance of a library. To open the gates easily, a wheel is fixed at 6 feet distance from the wall to which the gate is fixed. If one of the gates is opened to 90°, find the distance moved by the wheel (π = 3.14)
If x = `θ/360` × 2πr then what is x in the formula?
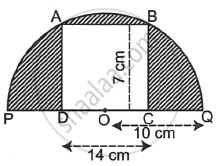
In the given figure, a rectangle ABCD is inscribed inside a semi-circle of radius 10 cm. Using the dimensions given in the figure, determine the area of the shaded region.
The perimeter of an arc of radius 4.2 cm is 12.8 cm. Determine the angle subtended by the arc at the centre of circle.
