Advertisements
Advertisements
प्रश्न
For the sectors with given measures, find the length of the arc, area and perimeter. (π = 3.14)
central angle 45°, r = 16 cm
उत्तर
central angle 45°, r = 16 cm
Length of the arc l = `theta^circ/(360^circ) xx 2pi"r" "units"`
l = `(45^circ)/(360^circ) xx 2 xx 3.14 xx 16 "cm"`
l = `1/8 xx 2 xx 3.14 xx 16 "cm"`
l = 12.56 cm
Area of the sector = `theta^circ/(360^circ) xx pi"r"^2 "sq. units"`
A = `(45^circ)/(360^circ) xx 3.14 xx 16 xx 16`
A = 100.48 cm2
Perimeter of the sector P = l + 2r units
P = 12.56 + 2(16) cm
P = 44.56 cm
APPEARS IN
संबंधित प्रश्न
The measure of an arc of a circle is 80° and its radius is 18 cm. Find the length of the arc. (π = 3.14)
Area of a sector of a circle of radius 15 cm is 30 cm2 . Find the length of the arc of the sector.
The area of a sector of a circle of 6 cm radius is 15 \[\pi\] sq.cm. Find the measure of the arc and length of the arc corresponding to the sector.
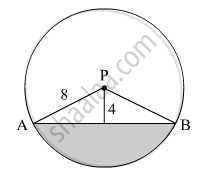
In the given figure, seg AB is a chord of a circle with centre P. If PA = 8 cm and distance of chord AB from the centre P is 4 cm, find the area of the shaded portion. ( \[\pi\] = 3.14, \[\sqrt{3}\]= 1.73 )

In the given figure, square ABCD is inscribed in the sector A - PCQ. The radius of sector C - BXD is 20 cm. Complete the following activity to find the area of shaded region
In Δ ABC, if ∠ A = 65° ; ∠ B = 40° then find the measure of ∠ C.
Match the following:
| Column A | Column B |
| (i) Area of a circle | (a) `1/4 pi"r"^2` |
| (ii) Circumference of a circle | (b) (π + 2)r |
| (iii) Area of the sector of a circle | (c) πr2 |
| (iv) Circumference of a semicircle | (d) 2πr |
| (v) Area of a quadrant of a circle | (e) `theta^circ/360^circ xx pi"r"^2` |
A circle of radius 120 m is divided into 8 equal sectors. Find the length of the arc of each of the sectors
Two gates are fitted at the entrance of a library. To open the gates easily, a wheel is fixed at 6 feet distance from the wall to which the gate is fixed. If one of the gates is opened to 90°, find the distance moved by the wheel (π = 3.14)
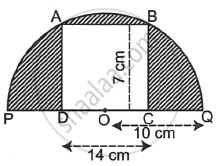
In the given figure, a rectangle ABCD is inscribed inside a semi-circle of radius 10 cm. Using the dimensions given in the figure, determine the area of the shaded region.