Advertisements
Advertisements
Question
How many three-digit numbers, which are divisible by 5, can be formed using the digits 0, 1, 2, 3, 4, 5 if repetition of digits are not allowed?
Solution
The repetition of digits are not allowed.
The given digits are 0, 1, 2, 3, 4, 5.
A number will be divisible by 5
if the digit in the unit place is 0 or 5
So the unit place can be filled by 0 or 5
(a) When the unit place is 0 it is filled in 1 way
And so 10’s place can be filled in 5 ways (by using 1, 2, 3, 4, 5)
And 100’s place can be filled in (5 – 1)4 ways
So the number of 3 digit numbers with unit place 0 = 1 × 5 × 4 = 20
(b) When the unit place is 5 it is filled in 1 way
Since 0 is given as a digit to fill 100’s place 0 should be excluded
So 100’s place can be filled in (excluding 0 and 5)4 ways
And 10’s place can be filled in (excluding 5 and one digit and including 0)4 ways
So the number of 3 digit numbers with unit place 5 = 1 × 4 × 4 = 16
∴ Number of 3 digit numbers ÷ by 5 = 20 + 16 = 36
APPEARS IN
RELATED QUESTIONS
How many 3-digit numbers can be formed from the digits 1, 2, 3, 4 and 5 assuming that repetition of the digits is allowed?
How many two-letter words can be formed using letters from the word SPACE, when repetition of letters is not allowed?
A Signal is generated from 2 flags by putting one flag above the other. If 4 flags of different colours are available, how many different signals can be generated?
How many two letter words can be formed using letters from the word SPACE, when repetition of letters is not allowed?
How many three-digit numbers can be formed using the digits 2, 3, 4, 5, 6 if digits can be repeated?
A letter lock contains 3 rings, each ring containing 5 different letters. Determine the maximum number of false trials that can be made before the lock is opened?
Answer the following:
A hall has 12 lamps and every lamp can be switched on independently. Find the number of ways of illuminating the hall.
There are 3 types of toy car and 2 types of toy train available in a shop. Find the number of ways a baby can buy a toy car and a toy train?
Four children are running a race:
In how many different ways could they finish the race?
How many three-digit numbers are there with 3 in the unit place?
without repetition
Count the numbers between 999 and 10000 subject to the condition that there are no digit is repeated
Find the value of 3! – 2!
Find the value of `(12!)/(9! xx 3!)`
Evaluate `("n"!)/("r"!("n" - "r")!)` when n = 10, r = 3
Choose the correct alternative:
In an examination there are three multiple choice questions and each question has 5 choices. Number of ways in which a student can fail to get all answer correct i
In how many ways can this diagram be coloured subject to the following two conditions?
(i) Each of the smaller triangle is to be painted with one of three colours: red, blue or green.
(ii) No two adjacent regions have the same colour.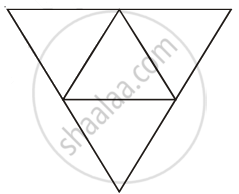
The number of different four-digit numbers that can be formed with the digits 2, 3, 4, 7 and using each digit only once is ______.
The sum of the digits in unit place of all the numbers formed with the help of 3, 4, 5 and 6 taken all at a time is ______.
The number of six-digit numbers, all digits of which are odd is ______.
In a steamer there are stalls for 12 animals, and there are horses, cows and calves (not less than 12 each) ready to be shipped. They can be loaded in 312 ways.
