Advertisements
Advertisements
Question
If |A| = 2 and |B| = 4, then match the relations in column I with the angle θ between A and B in column II
| Column I | Column II |
| (a) |A × B| = 0 | (i) θ = 30° |
| (b) |A × B| = 8 | (ii) θ = 45° |
| (c) |A × B| = 4 | (iii) θ = 90° |
| (d) |A × B| = `4sqrt(2)` | (iv) θ = 0° |
Solution
| Column I | Column II |
| (a) |A × B| = 0 | (iv) θ = 0° |
| (b) |A × B| = 8 | (iii) θ = 90° |
| (c) |A × B| = 4 | (i) θ = 30° |
| (d) |A × B| = `4sqrt(2)` | (ii) θ = 45° |
Explanation:
Given |A| = 2 and |B| = 4
(a) |A × B| = AB sin θ = 0
⇒ 2 × 4 sin θ = 0
⇒ sin θ = 0 = sin 0°
⇒ θ = 0°
(b) |A × B| = AB sin θ = 8
⇒ 2 × 4 sin θ = 8
⇒ sin θ = 1 = sin 90°
⇒ θ = 90°
(c) |A × B| = AB sin θ = 4
⇒ 2 × 4 sin θ = 4
⇒ sin θ = `1/2` = sin 30°
⇒ θ = 30°
(d) |A × B| = AB sin θ = `4sqrt(2)`
⇒ 2 × 4 sin θ = `4sqrt(2)`
⇒ sin θ = `1/sqrt(2)` = sin 45°
⇒ θ = 45°
APPEARS IN
RELATED QUESTIONS
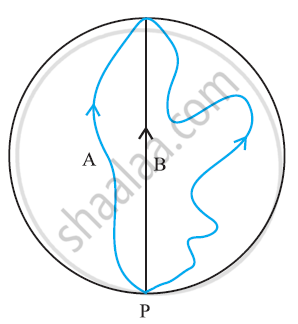
Three girls skating on a circular ice ground of radius 200 m start from a point P on the edge of the ground and reach a point Q diametrically opposite to P following different paths as shown in the figure. What is the magnitude of the displacement vector for each? For which girl is this equal to the actual length of the path skated?
Vectors `vecA = 5hati - ahatj + 3hatk` and `vecB = 10hati + 8hatj + bhatk` are parallel to each other, then values of 'a' and 'b' are
