Advertisements
Chapters
2: Units and Measurements
3: Motion In a Straight Line
▶ 4: Motion In a Plane
5: Laws of Motion
6: Work, Energy and Power
7: System of Particles and Rotational Motion
8: Gravitation
9: Mechanical Properties of Solids
10: Mechanical Properties of Fluids
11: Thermal Properties of Matter
12: Thermodynamics
13: Kinetic Theory
14: Oscillations
15: Waves
![NCERT Exemplar solutions for Physics [English] Class 11 chapter 4 - Motion In a Plane NCERT Exemplar solutions for Physics [English] Class 11 chapter 4 - Motion In a Plane - Shaalaa.com](/images/physics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 4: Motion In a Plane
Below listed, you can find solutions for Chapter 4 of CBSE NCERT Exemplar for Physics [English] Class 11.
NCERT Exemplar solutions for Physics [English] Class 11 4 Motion In a Plane Exercises [Pages 19 - 28]
MCQ I
The angle between A = `hati` + `hatj` and B = `hati` − `hatj` is ______.
45°
90°
– 45°
180°
Which one of the following statements is true?
A scalar quantity is the one that is conserved in a process.
A scalar quantity is the one that can never take negative values.
A scalar quantity is the one that does not vary from one point to another in space.
A scalar quantity has the same value for observers with different orientation of the axes.
Figure shows the orientation of two vectors u and v in the XY plane.
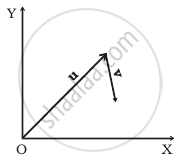
If `u = ahati + bhatj` and `v = phati + qhatj`
which of the following is correct?
a and p are positive while b and q are negative.
a, p and b are positive while q is negative.
a, q and b are positive while p is negative.
a, b, p and q are all positive.
The component of a vector r along X-axis will have maximum value if ______.
r is along positive Y-axis
r is along positive X-axis
r makes an angle of 45° with the X-axis
r is along negative Y-axis
The horizontal range of a projectile fired at an angle of 15° is 50 m. If it is fired with the same speed at an angle of 45°, its range will be ______.
60 m
71 m
100 m
141 m
Consider the quantities, pressure, power, energy, impulse, gravitational potential, electrical charge, temperature, area. Out of these, the only vector quantities are ______.
Impulse, pressure and area
Impulse and area
Area and gravitational potential
Impulse and pressure
In a two dimensional motion, instantaneous speed v0 is a positive constant. Then which of the following are necessarily true?
The average velocity is not zero at any time.
Average acceleration must always vanish.
Displacements in equal time intervals are equal.
Equal path lengths are traversed in equal intervals.
In a two dimensional motion, instantaneous speed v0 is a positive constant. Then which of the following are necessarily true?
The acceleration of the particle is zero.
The acceleration of the particle is bounded.
The acceleration of the particle is necessarily in the plane of motion.
The particle must be undergoing a uniform circular motion.
Three vectors A, B and C add up to zero. Find which is false.
(A × B) × C is not zero unless B, C are parallel
(A × B) . C is not zero unless B, C are parallel
If A, B, C define a plane, (A × B) × C is in that plane
(A × B) . C = |A| |B| |C| → C2 = A2 + B2
It is found that |A + B| = |A|.This necessarily implies ______.
B = 0
A, B are antiparallel
A, B are perpendicular
A . B ≤ 0
MCQ II
Two particles are projected in air with speed vo at angles θ1 and θ2 (both acute) to the horizontal, respectively. If the height reached by the first particle is greater than that of the second, then tick the right choices
- Angle of projection: q1 > q2
- Time of flight: T1 > T2
- Horizontal range: R1 > R2
- Total energy: U1 > U2
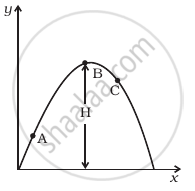
A particle slides down a frictionless parabolic (y = x2) track (A – B – C) starting from rest at point A (Figure). Point B is at the vertex of parabola and point C is at a height less than that of point A. After C, the particle moves freely in air as a projectile. If the particle reaches highest point at P, then

KE at P = KE at B
height at P = height at A
total energy at P = total energy at A
time of travel from A to B = time of travel from B to P
Following are four differrent relations about displacement, velocity and acceleration for the motion of a particle in general. Choose the incorrect one (s):
- `v_(av) = 1/2 [v(t_1) + v(t_2)]`
- `v_(av) = (r(t_2) - r(t_1))/(t_2 - t_1)`
- `r = 1/2 (v(t_2) - v(t_1))(t_2 - t_1)`
- `a_(av) = (v(t_2) - v(t_1))/(t_2 - t_1)`
For a particle performing uniform circular motion, choose the correct statement(s) from the following:
- Magnitude of particle velocity (speed) remains constant.
- Particle velocity remains directed perpendicular to radius vector.
- Direction of acceleration keeps changing as particle moves.
- Angular momentum is constant in magnitude but direction keeps changing.
For two vectors A and B, A + B = A − B is always true when
- |A| = |B| ≠ 0
- A ⊥ B
- |A| = |B| ≠ 0 and A and B are parallel or anti parallel
- When either |A| or |B| is zero
VSA
A cyclist starts from centre O of a circular park of radius 1 km and moves along the path OPRQO as shown figure. If he maintains constant speed of 10 ms–1, what is his acceleration at point R in magnitude and direction?

A particle is projected in air at some angle to the horizontal, moves along parabola as shown in figure, where x and y indicate horizontal and vertical directions, respectively. Show in the diagram, direction of velocity and acceleration at points A, B and C.
A ball is thrown from a roof top at an angle of 45° above the horizontal. It hits the ground a few seconds later. At what point during its motion, does the ball have
- greatest speed.
- smallest speed.
- greatest acceleration?
Explain
A football is kicked into the air vertically upwards. What is its acceleration?
A football is kicked into the air vertically upwards. What is its velocity at the highest point?
A, B and C are three non-collinear, non co-planar vectors. What can you say about direction of A × (B × C)?
SA
A boy travelling in an open car moving on a levelled road with constant speed tosses a ball vertically up in the air and catches it back. Sketch the motion of the ball as observed by a boy standing on the footpath. Give explanation to support your diagram.
A boy throws a ball in air at 60° to the horizontal along a road with a speed of 10 m/s (36 km/h). Another boy sitting in a passing by car observes the ball. Sketch the motion of the ball as observed by the boy in the car, if car has a speed of (18 km/h). Give explanation to support your diagram.
In dealing with motion of projectile in air, we ignore effect of air resistance on motion. This gives trajectory as a parabola as you have studied. What would the trajectory look like if air resistance is included? Sketch such a trajectory and explain why you have drawn it that way.
A fighter plane is flying horizontally at an altitude of 1.5 km with speed 720 km/h. At what angle of sight (w.r.t. horizontal) when the target is seen, should the pilot drop the bomb in order to attack the target?
Earth can be thought of as a sphere of radius 6400 km. Any object (or a person) is performing circular motion around the axis of earth due to earth’s rotation (period 1 day). What is acceleration of object on the surface of the earth (at equator) towards its centre? what is it at latitude θ? How does these accelerations compare with g = 9.8 m/s2?
Earth also moves in circular orbit around sun once every year with on orbital radius of 1.5 × 1011 m. What is the acceleration of earth (or any object on the surface of the earth) towards the centre of the sun? How does this acceleration compare with g = 9.8 m/s2?
Given below in column I are the relations between vectors a, b and c and in column II are the orientations of a, b and c in the XY plane. Match the relation in column I to correct orientations in column II.
| Column I | Column II | ||
| (a) | a + b = c | (i) | 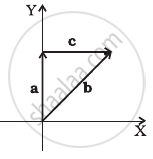 |
| (b) | a – c = b | (ii) |  |
| (c) | b – a = c | (iii) | 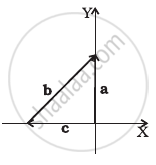 |
| (d) | a + b + c = 0 | (iv) | 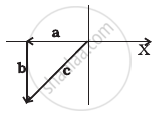 |
If |A| = 2 and |B| = 4, then match the relations in column I with the angle θ between A and B in column II.
| Column I | Column II |
| (a) A.B = 0 | (i) θ = 0 |
| (b) A.B = + 8 | (ii) θ = 90° |
| (c) A.B = 4 | (iii) θ = 180° |
| (d) A.B = – 8 | (iv) θ = 60° |
If |A| = 2 and |B| = 4, then match the relations in column I with the angle θ between A and B in column II
| Column I | Column II |
| (a) |A × B| = 0 | (i) θ = 30° |
| (b) |A × B| = 8 | (ii) θ = 45° |
| (c) |A × B| = 4 | (iii) θ = 90° |
| (d) |A × B| = `4sqrt(2)` | (iv) θ = 0° |
LA
A hill is 500 m high. Supplies are to be sent across the hill using a canon that can hurl packets at a speed of 125 m/s over the hill. The canon is located at a distance of 800 m from the foot of hill and can be moved on the ground at a speed of 2 m/s; so that its distance from the hill can be adjusted. What is the shortest time in which a packet can reach on the ground across the hill? Take g = 10 m/s2.
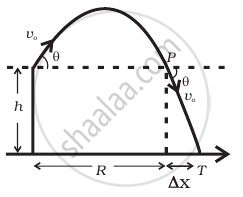
A gun can fire shells with maximum speed v0 and the maximum horizontal range that can be achieved is R = `v_0^2/g`. If a target farther away by distance ∆x (beyond R) has to be hit with the same gun (Figure), show that it could be achieved by raising the gun to a height at least `h = Δx[ 1 + (Δx)/R]`
A particle is projected in air at an angle β to a surface which itself is inclined at an angle α to the horizontal (Figure).
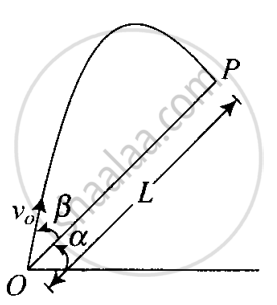
- Find an expression of range on the plane surface (distance on the plane from the point of projection at which particle will hit the surface).
- Time of flight.
- β at which range will be maximum.
A particle falling vertically from a height hits a plane surface inclined to horizontal at an angle θ with speed vo and rebounds elastically (Figure). Find the distance along the plane where if will hit second time.

A girl riding a bicycle with a speed of 5 m/s towards north direction, observes rain falling vertically down. If she increases her speed to 10 m/s, rain appears to meet her at 45° to the vertical. What is the speed of the rain? In what direction does rain fall as observed by a ground based observer?
A river is flowing due east with a speed 3 m/s. A swimmer can swim in still water at a speed of 4 m/s (Figure).

- If swimmer starts swimming due north, what will be his resultant velocity (magnitude and direction)?
- If he wants to start from point A on south bank and reach opposite point B on north bank, (a) which direction should he swim? (b) what will be his resultant speed?
- From two different cases as mentioned in (a) and (b) above, in which case will he reach opposite bank in shorter time?
A cricket fielder can throw the cricket ball with a speed vo. If he throws the ball while running with speed u at an angle θ to the horizontal, find
- the effective angle to the horizontal at which the ball is projected in air as seen by a spectator.
- what will be time of flight?
- what is the distance (horizontal range) from the point of projection at which the ball will land?
- find θ at which he should throw the ball that would maximise the horizontal range as found in (iii).
- how does θ for maximum range change if u > vo, u = vo, u < vo?
- how does θ in (v) compare with that for u = 0 (i.e.45)?
Motion in two dimensions, in a plane can be studied by expressing position, velocity and acceleration as vectors in cartesian co-ordinates A = `A_xhati + A_yhatj` where `hati` and `hatj` are unit vector along x and y directions, respectively and Ax and Ay are corresponding components of (Figure). Motion can also be studied by expressing vectors in circular polar co-ordinates as A = `A_rhatr + A_θhatθ` where `hatr = r/r = cos θhati + sin θj` and `hatθ = - sin θhati + cos θ hatj` are unit vectors along direction in which `r` and `θ` are increasing.

- Express `hati` and `hatj` in terms of `hatr` and `hatθ`
- Show that both `hatr` and `hatθ` are unit vectors and are perpendicular to each other.
- Show that `d/(dt) (hatr) = ωhatθ` where `θ = (dθ)/(dt)` and `d/(dt) (hatθ) = - ωhatr`
- For a particle moving along a spiral given by `t = aθhatr`, where a = 1 (unit), find dimensions of ‘a’.
- Find velocity and acceleration in polar vector representation for particle moving along spiral described in (d) above.
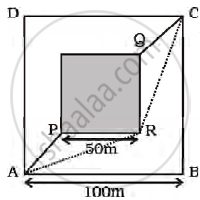
A man wants to reach from A to the opposite corner of the square C (Figure). The sides of the square are 100 m. A central square of 50 m × 50 m is filled with sand. Outside this square, he can walk at a speed 1 m/s. In the central square, he can walk only at a speed of v m/s (v < 1). What is smallest value of v for which he can reach faster via a straight path through the sand than any path in the square outside the sand?
Solutions for 4: Motion In a Plane
![NCERT Exemplar solutions for Physics [English] Class 11 chapter 4 - Motion In a Plane NCERT Exemplar solutions for Physics [English] Class 11 chapter 4 - Motion In a Plane - Shaalaa.com](/images/physics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
NCERT Exemplar solutions for Physics [English] Class 11 chapter 4 - Motion In a Plane
Shaalaa.com has the CBSE Mathematics Physics [English] Class 11 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. NCERT Exemplar solutions for Mathematics Physics [English] Class 11 CBSE 4 (Motion In a Plane) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. NCERT Exemplar textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Physics [English] Class 11 chapter 4 Motion In a Plane are Scalar (Dot) and Vector (Cross) Product of Vectors, Relative Velocity in Two Dimensions, Cases of Uniform Velocity, Scalars and Vectors, Multiplication of Vectors by a Real Number or Scalar, Addition and Subtraction of Vectors - Graphical Method, Resolution of Vectors, Vector Addition – Analytical Method, Motion in a Plane, Motion in a Plane with Constant Acceleration, Projectile Motion, General Vectors and Their Notations, Rectangular Components, Uniform Circular Motion (UCM), Cases of Uniform Acceleration Projectile Motion, Introduction of Motion in One Dimension, Motion in a Plane - Average Velocity and Instantaneous Velocity, Motion in a Plane - Average Acceleration and Instantaneous Acceleration, Angular Velocity.
Using NCERT Exemplar Physics [English] Class 11 solutions Motion In a Plane exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in NCERT Exemplar Solutions are essential questions that can be asked in the final exam. Maximum CBSE Physics [English] Class 11 students prefer NCERT Exemplar Textbook Solutions to score more in exams.
Get the free view of Chapter 4, Motion In a Plane Physics [English] Class 11 additional questions for Mathematics Physics [English] Class 11 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
