Advertisements
Chapters
2: Units and Measurements
3: Motion In a Straight Line
4: Motion In a Plane
5: Laws of Motion
6: Work, Energy and Power
7: System of Particles and Rotational Motion
8: Gravitation
9: Mechanical Properties of Solids
10: Mechanical Properties of Fluids
11: Thermal Properties of Matter
12: Thermodynamics
▶ 13: Kinetic Theory
14: Oscillations
15: Waves
![NCERT Exemplar solutions for Physics [English] Class 11 chapter 13 - Kinetic Theory NCERT Exemplar solutions for Physics [English] Class 11 chapter 13 - Kinetic Theory - Shaalaa.com](/images/physics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 13: Kinetic Theory
Below listed, you can find solutions for Chapter 13 of CBSE NCERT Exemplar for Physics [English] Class 11.
NCERT Exemplar solutions for Physics [English] Class 11 13 Kinetic Theory Exercises [Pages 90 - 96]
MCQ I
A cubic vessel (with faces horizontal + vertical) contains an ideal gas at NTP. The vessel is being carried by a rocket which is moving at a speed of 500 ms–1 in vertical direction. The pressure of the gas inside the vessel as observed by us on the ground ______.
remains the same because 500 ms−1 is very much smaller than vrms of the gas.
remains the same because motion of the vessel as a whole does not affect the relative motion of the gas molecules and the walls.
will increase by a factor equal to `(v_(rms)^2 + (500)^2)/v_(rms)^2` where vrms was the original mean square velocity of the gas.
will be different on the top wall and bottom wall of the vessel.
1 mole of an ideal gas is contained in a cubical volume V, ABCDEFGH at 300 K (Figure). One face of the cube (EFGH) is made up of a material which totally absorbs any gas molecule incident on it. At any given time ______.
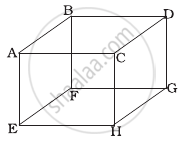
the pressure on EFGH would be zero.
the pressure on all the faces will the equal.
the pressure of EFGH would be double the pressure on ABCD.
the pressure on EFGH would be half that on ABCD.
Boyle’s law is applicable for an ______.
adiabatic process.
isothermal process.
isobaric process.
isochoric process.
A cylinder containing an ideal gas is in vertical position and has a piston of mass M that is able to move up or down without friction (Figure). If the temperature is increased ______.
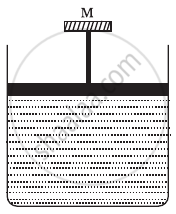
both p and V of the gas will change.
only p will increase according to Charle’s law.
V will change but not p.
p will change but not V.
Volume versus temperature graphs for a given mass of an ideal gas are shown in figure at two different values of constant pressure. What can be inferred about relation between P1 and P2?
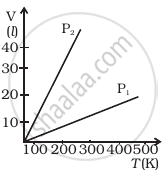
P1 > P2
P1 = P2
P1 < P2
data is insufficient.
1 mole of H2 gas is contained in a box of volume V = 1.00 m3 at T = 300K. The gas is heated to a temperature of T = 3000K and the gas gets converted to a gas of hydrogen atoms. The final pressure would be (considering all gases to be ideal) ______.
same as the pressure initially.
2 times the pressure initially.
10 times the pressure initially.
20 times the pressure initially.
A vessel of volume V contains a mixture of 1 mole of Hydrogen and 1 mole of Oxygen (both considered as ideal). Let f1(v)dv, denote the fraction of molecules with speed between v and (v + dv) with f2(v)dv, similarly for oxygen. Then ______.
f1(v) + f2(v) = f(v) obeys the Maxwell’s distribution law.
f1(v), f2(v) will obey the Maxwell’s distribution law separately.
Neither f1(v), nor f2(v) will obey the Maxwell’s distribution law.
f2(v) and f1(v) will be the same.
An inflated rubber balloon contains one mole of an ideal gas, has a pressure p, volume V and temperature T. If the temperature rises to 1.1 T, and the volume is increased to 1.05 V, the final pressure will be ______.
1.1 p
p
less than p
between p and 1.1.
MCQ II
ABCDEFGH is a hollow cube made of an insulator (Figure). Face ABCD has positive charge on it. Inside the cube, we have ionized hydrogen. The usual kinetic theory expression for pressure ______.
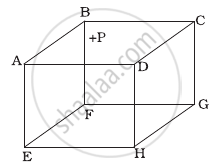
- will be valid.
- will not be valid since the ions would experience forces other than due to collisions with the walls.
- will not be valid since collisions with walls would not be elastic.
- will not be valid because isotropy is lost.
Diatomic molecules like hydrogen have energies due to both translational as well as rotational motion. From the equation in kinetic theory `pV = 2/3` E, E is ______.
- the total energy per unit volume.
- only the translational part of energy because rotational energy is very small compared to the translational energy.
- only the translational part of the energy because during collisions with the wall pressure relates to change in linear momentum.
- the translational part of the energy because rotational energies of molecules can be of either sign and its average over all the molecules is zero.
In a diatomic molecule, the rotational energy at a given temperature ______.
- obeys Maxwell’s distribution.
- have the same value for all molecules.
- equals the translational kinetic energy for each molecule.
- is (2/3)rd the translational kinetic energy for each molecule.
Which of the following diagrams (Figure) depicts ideal gas behaviour?
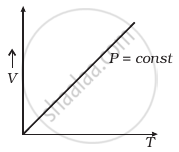 (a) |
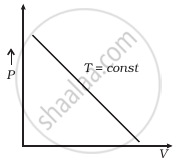 (b) |
 (c) |
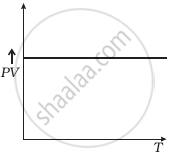 (d) |
When an ideal gas is compressed adiabatically, its temperature rises: the molecules on the average have more kinetic energy than before. The kinetic energy increases ______.
- because of collisions with moving parts of the wall only.
- because of collisions with the entire wall.
- because the molecules gets accelerated in their motion inside the volume.
- because of redistribution of energy amongst the molecules.
VSA
Calculate the number of atoms in 39.4 g gold. Molar mass of gold is 197g mole–1.
The volume of a given mass of a gas at 27°C, 1 atm is 100 cc. What will be its volume at 327°C?
The molecules of a given mass of a gas have root mean square speeds of 100 ms−1 at 27°C and 1.00 atmospheric pressure. What will be the root mean square speeds of the molecules of the gas at 127°C and 2.0 atmospheric pressure?
Two molecules of a gas have speeds of 9 × 10 6 ms−1 and 1 × 106 ms−1, respectively. What is the root mean square speed of these molecules?
A gas mixture consists of 2.0 moles of oxygen and 4.0 moles of neon at temperature T. Neglecting all vibrational modes, calculate the total internal energy of the system. (Oxygen has two rotational modes.)
Calculate the ratio of the mean free paths of the molecules of two gases having molecular diameters 1 A and 2 A. The gases may be considered under identical conditions of temperature, pressure and volume.
The container shown in figure has two chambers, separated by a partition, of volumes V1 = 2.0 litre and V2 = 3.0 litre. The chambers contain µ1 = 4.0 and µ2 = 5.0 moles of a gas at pressures p1 = 1.00 atm and p2 = 2.00 atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.
| V1 | V2 |
| µ1, p1 | µ2 |
| p2 |
A gas mixture consists of molecules of types A, B and C with masses mA > mB > mC. Rank the three types of molecules in decreasing order of average K.E.
A gas mixture consists of molecules of types A, B and C with masses mA > mB > mC. Rank the three types of molecules in decreasing order of rms speeds.
We have 0.5 g of hydrogen gas in a cubic chamber of size 3 cm kept at NTP. The gas in the chamber is compressed keeping the temperature constant till a final pressure of 100 atm. Is one justified in assuming the ideal gas law, in the final state?
(Hydrogen molecules can be consider as spheres of radius 1 Å).
When air is pumped into a cycle tyre the volume and pressure of the air in the tyre both are increased. What about Boyle’s law in this case?
A ballon has 5.0 g mole of helium at 7°C. Calculate
- the number of atoms of helium in the balloon
- the total internal energy of the system.
Calculate the number of degrees of freedom of molecules of hydrogen in 1 cc of hydrogen gas at NTP.
An insulated container containing monoatomic gas of molar mass m is moving with a velocity vo. If the container is suddenly stopped, find the change in temperature.
Explain why there is no atmosphere on moon.
Explain why there is fall in temperature with altitude.
Consider an ideal gas with following distribution of speeds.
| Speed (m/s) | % of molecules |
| 200 | 10 |
| 400 | 20 |
| 600 | 40 |
| 800 | 20 |
| 1000 | 10 |
Calculate Vrms and hence T. (m = 3.0 × 10−26 kg)
Consider an ideal gas with following distribution of speeds.
| Speed (m/s) | % of molecules |
| 20 | 10 |
| 400 | 20 |
| 600 | 40 |
| 800 | 20 |
| 1000 | 10 |
If all the molecules with speed 1000 m/s escape from the system, calculate new Vrms and hence T.
Ten small planes are flying at a speed of 150 km/h in total darkness in an air space that is 20 × 20 × 1.5 km3 in volume. You are in one of the planes, flying at random within this space with no way of knowing where the other planes are. On the average about how long a time will elapse between near collision with your plane. Assume for this rough computation that a saftey region around the plane can be approximated by a sphere of radius 10 m.
A box of 1.00 m3 is filled with nitrogen at 1.50 atm at 300K. The box has a hole of an area 0.010 mm2. How much time is required for the pressure to reduce by 0.10 atm, if the pressure outside is 1 atm.
Consider a rectangular block of wood moving with a velocity v0 in a gas at temperature T and mass density ρ. Assume the velocity is along x-axis and the area of cross-section of the block perpendicular to v0 is A. Show that the drag force on the block is `4ρAv_0 sqrt((KT)/m)`, where m is the mass of the gas molecule.
Solutions for 13: Kinetic Theory
![NCERT Exemplar solutions for Physics [English] Class 11 chapter 13 - Kinetic Theory NCERT Exemplar solutions for Physics [English] Class 11 chapter 13 - Kinetic Theory - Shaalaa.com](/images/physics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
NCERT Exemplar solutions for Physics [English] Class 11 chapter 13 - Kinetic Theory
Shaalaa.com has the CBSE Mathematics Physics [English] Class 11 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. NCERT Exemplar solutions for Mathematics Physics [English] Class 11 CBSE 13 (Kinetic Theory) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. NCERT Exemplar textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Physics [English] Class 11 chapter 13 Kinetic Theory are Specific Heat Capacities - Gases, Mean Free Path, Kinetic Theory of Gases - Concept of Pressure, Equation of State of a Perfect Gas, Work Done in Compressing a Gas, Introduction of Kinetic Theory of an Ideal Gas, Interpretation of Temperature in Kinetic Theory, Gases and Its Characteristics, Assumptions of Kinetic Theory of Gases, RMS Speed of Gas Molecules, Degrees of Freedom, Avogadro's Number, Molecular Nature of Matter, Law of Equipartition of Energy.
Using NCERT Exemplar Physics [English] Class 11 solutions Kinetic Theory exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in NCERT Exemplar Solutions are essential questions that can be asked in the final exam. Maximum CBSE Physics [English] Class 11 students prefer NCERT Exemplar Textbook Solutions to score more in exams.
Get the free view of Chapter 13, Kinetic Theory Physics [English] Class 11 additional questions for Mathematics Physics [English] Class 11 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
