Advertisements
Advertisements
Question
In a diatomic molecule, the rotational energy at a given temperature ______.
- obeys Maxwell’s distribution.
- have the same value for all molecules.
- equals the translational kinetic energy for each molecule.
- is (2/3)rd the translational kinetic energy for each molecule.
Solution
a and d
Explanation:
Consider a diatomic molecule as shown in the diagram.
The total energy associated with the molecule is
`E = 1/2 mv_x^2 + 1/2 mv_y^2 + 1/2 mv_z^2 + 1/2 I_xω_x^2 + 1/2 I_yω_y^2`
This above expression contains translational kinetic energy `(1/2 mv^2)` corresponding to velocity in each x, y and z-directions as well as rotational KE `(1/2 Iω^2)` associated with the axis of rotations x and y.
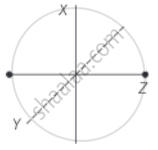
The number of independent terms in the above expression is 5.
As we can predict the velocities of molecules by Maxwell's distribution, hence the above expression also obeys Maxwell's distribution.
∵ 2 rotational and 3 translational energies are associated with each molecule.
∴ Rational energy at a given temperature is `(2/3)`rd of translational KE of each molecule.
APPEARS IN
RELATED QUESTIONS
The energy of a given sample of an ideal gas depends only on its
Keeping the number of moles, volume and temperature the same, which of the following are the same for all ideal gases?
Find the number of molecules in 1 cm3 of an ideal gas at 0°C and at a pressure of 10−5mm of mercury.
Use R = 8.31 J K-1 mol-1
Let Q and W denote the amount of heat given to an ideal gas and the work done by it in an isothermal process.
A vessel containing one mole of a monatomic ideal gas (molecular weight = 20 g mol−1) is moving on a floor at a speed of 50 m s−1. The vessel is stopped suddenly. Assuming that the mechanical energy lost has gone into the internal energy of the gas, find the rise in its temperature.
An ideal gas (Cp / Cv = γ) is taken through a process in which the pressure and the volume vary as p = aVb. Find the value of b for which the specific heat capacity in the process is zero.
1 litre of an ideal gas (γ = 1.5) at 300 K is suddenly compressed to half its original volume. (a) Find the ratio of the final pressure to the initial pressure. (b) If the original pressure is 100 kPa, find the work done by the gas in the process. (c) What is the change in internal energy? (d) What is the final temperature? (e) The gas is now cooled to 300 K keeping its pressure constant. Calculate the work done during the process. (f) The gas is now expanded isothermally to achieve its original volume of 1 litre. Calculate the work done by the gas. (g) Calculate the total work done in the cycle.
The figure shows an adiabatic cylindrical tube of volume V0 divided in two parts by a frictionless adiabatic separator. Initially, the separator is kept in the middle, an ideal gas at pressure p1 and temperature T1 is injected into the left part and another ideal gas at pressure p2 and temperature T2 is injected into the right part. Cp/Cv = γ is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the volumes of the two parts (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.

An ideal gas of density 1.7 × 10−3 g cm−3 at a pressure of 1.5 × 105 Pa is filled in a Kundt's tube. When the gas is resonated at a frequency of 3.0 kHz, nodes are formed at a separation of 6.0 cm. Calculate the molar heat capacities Cp and Cv of the gas.
We have 0.5 g of hydrogen gas in a cubic chamber of size 3 cm kept at NTP. The gas in the chamber is compressed keeping the temperature constant till a final pressure of 100 atm. Is one justified in assuming the ideal gas law, in the final state?
(Hydrogen molecules can be consider as spheres of radius 1 Å).
