Advertisements
Advertisements
Question
If ABCD is a rectangle with ∠BAC = 32°, find the measure of ∠DBC.
Solution
Figure is given as :
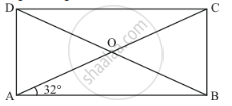
Suppose the diagonals AC and BD intersect at O.
Since, diagonals of a rectangle are equal and they bisect each other.
Therefore, in ΔOAB, we have
OA = OB
Angles opposite to equal sides are equal.
Therefore,
∠OAB = ∠OBA
∠BAC = ∠DBA
But, ∠BAC = 32°
∠DBA = 32°
Now,
∠ABC = 90°
∠DBA + ∠DBC = 90°
32° +∠DBC = 90°
∠DBC = 58°
Hence, the measure of ∠DBC is 58°.
APPEARS IN
RELATED QUESTIONS
Complete of the following, so as to make a true statement:
The sum of the angles of a quadrilateral is ......
In Fig. 16.19, ABCD is a quadrilateral.
Name a pair of opposite sides.
A quadrilateral has three acute angles each measures 80°. What is the measure of the fourth angle?
One diagonal of a rectangle is 18 cm. What is the length of its other diagonal?
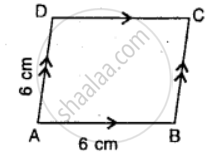
Write, giving reason, the name of the figure drawn alongside. Under what condition will this figure be a square.
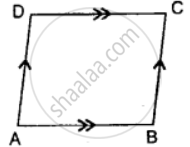
Write two conditions that will make the adjoining figure a square.
Observe the figure below and find out their name.
ABCDE is a pentagon in which AB is parallel to DC and ∠A : ∠E : ∠D = 1 : 2 : 3. Find angle A.
In given figure, What is BD – BE?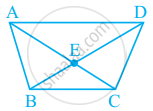
Draw a rough sketch of a quadrilateral KLMN. State two pairs of adjacent angles.
