Advertisements
Advertisements
Question
The diagonals of a rectangle ABCD meet at O, If ∠BOC = 44°, find ∠OAD.
Solution
The rectangle ABCD is given as:
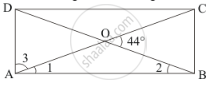
We have,
∠BOC +∠BOA = 180° (Linear pair)
44° +∠BOA = 180°
∠BOA = 180° -44°
∠BOA = 136°
Since, diagonals of a rectangle are equal and they bisect each other. Therefore, in ΔOAB, we have
OA = OB (Angles opposite to equal sides are equal.)
Therefore,
∠1 = ∠2
Now,in ΔOAB, we have
∠BOA + ∠1 +∠2 = 180
∠BOA + 2∠1 = 180°
2∠1 = 44°
∠1 = 22°
Since, each angle of a rectangle is a right angle.
Therefore,
∠BAD = 90°
∠1+∠3 = 90°
22° +∠3 = 90°
∠3 = 68°
Thus, ∠OAD = 68°
Hence, the measure of∠OAD is 68°.
APPEARS IN
RELATED QUESTIONS
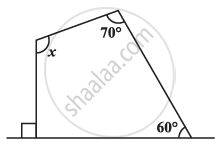
Find the angle measure x in the given Figure
Three angles of a quadrilateral are respectively equal to 110°, 50° and 40°. Find its fourth angle
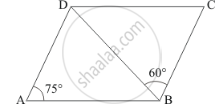
In Fig. below, ABCD is a parallelogram in which ∠DAB = 75° and ∠DBC = 60°. Compute
∠CDB and ∠ADB.
If an angle of a parallelogram is two-third of its adjacent angle, the smallest angle of the parallelogram is
In a rhombus ABCD, if ∠ACB = 40°, then ∠ADB =
All the angles of a quadrilateral are equal. What special name is given to this quadrilateral?
The angle between two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 60º. Find the angles of the parallelogram.
In the following figure, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP = ∠DAP. Prove that AD = 2CD.

In a quadrilateral PQRS, ∠P = 50°, ∠Q = 50°, ∠R = 60°. Find ∠S. Is this quadrilateral convex or concave?
Three angles of a quadrilateral are equal. Fourth angle is of measure 120°. What is the measure of equal angles?
