Advertisements
Advertisements
Question
If the area of a trapezium is 28 cm2 and one of its parallel sides is 6 cm, find the other parallel side if its altitude is 4 cm.
Solution
Given:
Area of the trapezium = 28 cm2
Length of one of its parallel sides = 6 cm
Altitude = 4 cm
Let the other side be x cm.
Area of trapezium \[=\frac{1}{2}\times(\text{ Sum of the parallel sides })\times(\text{ Altitude })\]
\[ \Rightarrow 28=\frac{1}{2}\times(6+x)\times(4)\]
\[ \Rightarrow 28 = 2 \times (6 + x)\]
\[ \Rightarrow 6+x=\frac{28}{2}=14\]
\[ \Rightarrow x=14-6=8 cm\]
Hence, the length of the other parallel side of the trapezium is 8 cm.
APPEARS IN
RELATED QUESTIONS
Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.
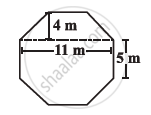
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 8 m and 60 dm, altitude = 40 dm
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 150 cm and 30 dm, altitude = 9 dm.
Find the area of a trapezium whose parallel sides of lengths 10 cm and 15 cm are at a distance of 6 cm from each other. Calculate this area as the difference of the area of a rectangle and the sum of the areas of two triangles.
Length of the two parallel sides of a trapezium are 8.5 cm and 11.5 cm respectively and its height is 4.2 cm, find its area.
Find the missing values.
| Height 'h' | Parallel side 'a` | Parallel side 'b` | Area |
| 10 m | 12 m | 20 m |
The area of a trapezium is 1586 sq.cm. The distance between its parallel sides is 26 cm. If one of the parallel sides is 84 cm then find the other side
The sunshade of a window is in the form of isosceles trapezium whose parallel sides are 81 cm and 64 cm and the distance between them is 6 cm. Find the cost of painting the surface at the rate of ₹ 2 per sq.cm
When the non-parallel sides of a trapezium are equal then it is known as
The areas of two circles are in the ratio 49 : 64. Find the ratio of their circumferences.
