Advertisements
Advertisements
Question
If two zeroes of the polynomial x4 – 6x3 – 26x2 + 138x – 35 are 2 ± `sqrt3` , find other zeroes
Advertisements
Solution 1
Given that 2 + `sqrt3` and 2 - `sqrt3` are zeroes of the given polynomial
Therefore, `(x - 2-sqrt3)(x-2+sqrt3)` = x2 + 4 - 4x - 3
= x2 - 4x + 1 is a factor of the given polynomial
For finding the remaining zeroes of the given polynomial, we will find the quotient by dividing by x4 - 6x3 - 26x2 + 138x - 35 by x2 - 4x + 1
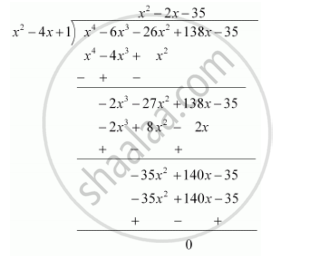
Clearly x4 - 6x3 - 26x2 + 138x - 35 = (x2 - 4x +1)(x2 -2x -35)
It can be observed that (x2 - 2x - 35) is also a factor of the given polynomial.
And (x2 - 2x - 35) = (x -7)(x + 5)
Therefore, the value of the polynomial is also zero when x - 7 = 0 or x + 5 = 0
or x = 7 or -5
Hence, 7 and - 5 are also zeroes of this polynomial
Solution 2
2+√3 and 2-√3 are two zeroes of the polynomial p(x) = x4 – 6x3 – 26x2 + 138x – 35.
Let x = 2±√3
So, x-2 = ±√3
On squaring, we get x2 - 4x + 4 = 3,
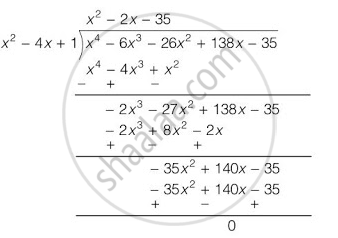
= (x2 - 4x + 1) (x2 - 2x - 35)
= (x2 - 4x + 1) (x2 - 7x + 5x - 35)
= (x2 - 4x + 1) [x(x - 7) + 5 (x - 7)]
= (x2 - 4x + 1) (x + 5) (x - 7)
∴ (x + 5) and (x - 7) are other factors of p(x).
∴ - 5 and 7 are other zeroes of the given polynomial.
APPEARS IN
RELATED QUESTIONS
Find the zeros of the quadratic polynomial 9x2 - 5 and verify the relation between the zeros and its coefficients.
Prove relation between the zeros and the coefficient of the quadratic polynomial ax2 + bx + c
Find the zeroes of the following quadratic polynomial and verify the relationship between the zeroes and the coefficients:
3x2 – x – 4
Find a quadratic polynomial with the given numbers as the sum and product of its zeroes, respectively.
`sqrt2 , 1/3`
Find a quadratic polynomial with the given numbers as the sum and product of its zeroes respectively.
4, 1
Find a cubic polynomial with the sum, sum of the product of its zeroes taken two at a time, and the product of its zeroes as 2, − 7, − 14 respectively
If α and β are the zeroes of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate `1/(aalpha+b)+1/(abeta+b)`.
If 𝛼 and 𝛽 are the zeros of the quadratic polynomial p(x) = 4x2 − 5x −1, find the value of α2β + αβ2.
If a and 3 are the zeros of the quadratic polynomial f(x) = x2 + x − 2, find the value of `1/alpha-1/beta`.
If α and β are the zeros of the quadratic polynomial p(s) = 3s2 − 6s + 4, find the value of `alpha/beta+beta/alpha+2[1/alpha+1/beta]+3alphabeta`
If the squared difference of the zeros of the quadratic polynomial f(x) = x2 + px + 45 is equal to 144, find the value of p.
If one zero of the quadratic polynomial f(x) = 4x2 − 8kx − 9 is negative of the other, find the value of k.
Find the zeroes of the quadratic polynomial `2x^2 ˗ 11x + 15` and verify the relation between the zeroes and the coefficients.
Find the zeroes of the quadratic polynomial `(3x^2 ˗ x ˗ 4)` and verify the relation between the zeroes and the coefficients.
Given that the zeroes of the cubic polynomial x3 – 6x2 + 3x + 10 are of the form a, a + b, a + 2b for some real numbers a and b, find the values of a and b as well as the zeroes of the given polynomial.
A quadratic polynomial the sum and product of whose zeroes are – 3 and 2 respectively, is ______.
If α, β are zeroes of the quadratic polynomial x2 – 5x + 6, form another quadratic polynomial whose zeroes are `1/α, 1/β`.
Find a quadratic polynomial whose zeroes are 6 and – 3.
The zeroes of the polynomial p(x) = 25x2 – 49 are ______.
The zeroes of the polynomial p(x) = 2x2 – x – 3 are ______.
