Advertisements
Advertisements
Question
In a photographic process, the developing time of prints may be looked upon as a random variable having the normal distribution with a mean of 16.28 seconds and a standard deviation of 0.12 second. Find the probability that it will take less than 16.35 seconds to develop prints
Solution
Let x be the random variable have long the normal distribution with a mean of 16.28 seconds and a standard deviation of 0.12 second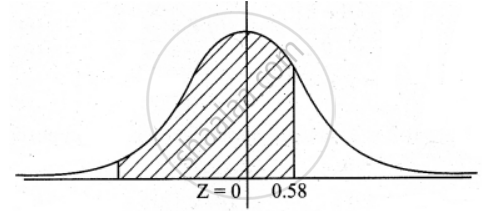
µ = 16,28 and σ = 0.12
The standard normal variate
z = `(x - mu)/sigma = (x - 16.28)/0.12` = 1
P(Less than 16.35 seconds) = P(x < 16.35)
When x = 16.35
z = `(16.35 - 16.28)/0.12 = 0.17/0.12` = 0.583
P(X < 16.35) = P(Z < 0.583)
= P(`-oo` < z < 0) + P(0 < z < 0.583)
= 0.5 + 0.2190
= 0.7190
APPEARS IN
RELATED QUESTIONS
In a particular university 40% of the students are having newspaper reading habit. Nine university students are selected to find their views on reading habit. Find the probability that none of those selected have newspaper reading habit
Forty percent of business travellers carry a laptop. In a sample of 15 business travelers, what is the probability that 3 will have a laptop?
Write any 2 examples for Poisson distribution
The average number of customers, who appear in a counter of a certain bank per minute is two. Find the probability that during a given minute no customer appears
In a distribution 30% of the items are under 50 and 10% are over 86. Find the mean and standard deviation of the distribution
If the heights of 500 students are normally distributed with mean 68.0 inches and standard deviation 3.0 inches, how many students have height between 65 and 71 inches
Choose the correct alternative:
If Z is a standard normal variate, the proportion of items lying between Z = – 0.5 and Z = – 3.0 is
Choose the correct alternative:
A manufacturer produces switches and experiences that 2 percent switches are defective. The probability that in a box of 50 switches, there are atmost two defective is :
Choose the correct alternative:
Which of the following cannot generate a Poisson distribution?
Hospital records show that of patients suffering from a certain disease 75% die of it. What is the probability that of 6 randomly selected patients, 4 will recover?
