Advertisements
Advertisements
Question
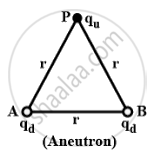
- In a quark model of elementary particles, a neutron is made of one up quarks [charge (2/3) e] and two down quarks [charges –(1/3) e]. Assume that they have a triangle configuration with side length of the order of 10–15 m. Calculate electrostatic potential energy of neutron and compare it with its mass 939 MeV.
- Repeat above exercise for a proton which is made of two up and one down quark.
Solution
This system is made up of three charges. The potential energy of the system is equal to the algebraic sum of the PE of each pair. So,
a. `q_d = 1/3 e` charge on down quark
`q_u = + 2/3 e` charge on up quark
Potential energy U = `(kq_1q_2)/r`
`k = 1/(4piε_0)`
U = `(kq_1q_2)/r + (kq_1q_3)/r + (kq_2q_3)/r`
∴ Un = `1/(4piε_0) ((-q_d)(-q_d))/r + ((-q_d)q_u)/(4piε_0) + (q_u (-q_d))/(4piε_0r)`
= `(q_d)/(4piε_0r) [+q_d - q_u - q_u]` Talking sign of charge
= `(q_d)/(4piε_0r) [q_d - 2q_u]`
= `(9 xx 10^9 xx 1/3 e)/(10^-15) [1/3 e - 2 * 2/3 e]` nature sign of charge taken already
= `(9 xx 10^9 xx e)/(3 xx 10^-15) * e/3 [1 - 4]` Joule
= `(-3 xx 9 xx 10^9 xx 1.6 xx 10^-19)/(9 xx 10^-15)` e Joule
= `- 4.8 xx 10^(9 - 19 - 15) eV`
= `4.8 xx 10^5 eV`
= `- 0.4.8 xx 10^6 eV`
U = – 0.48 MeV
So, charges inside neutron [1qu and 2qd] are attracted by energy of 0.48 MeV.
Energy released by a neutron when converted into energy is 939 MeV.
∴ Required ratio = `(1 - 0.481 MeV)/(939 MeV)` = 0.0005111 = 5.11 × 10–4
b. P.E. of proton consists of 2 up and 1 down quark
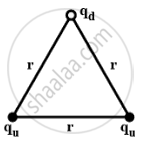
r = 10–15 m
qd = `- 1/3 e, q_u = 2/3 e`
Up = `1/(4 piε_0) (q_u xx q_u)/r + (q_u (-q_d))/(4 piε_0r) + (q_u (-q_d))/(4 piε_0r)`
= `q/(4 piε_0r) [q_u - q_d - q_d]`
= `q/(4 piε_0r) [q_u - 2q_d]`
= `(9 xx 10^9)/10^-13 2/3 e [2/3 e - 2 1/3 e]` = 0
APPEARS IN
RELATED QUESTIONS
If one of the two electrons of a H2 molecule is removed, we get a hydrogen molecular ion `"H"_2^+`. In the ground state of an `"H"_2^+`, the two protons are separated by roughly 1.5 Å, and the electron is roughly 1 Å from each proton. Determine the potential energy of the system. Specify your choice of zero potential energy.
Four point charges Q, q, Q and q are placed at the corners of a square of side 'a' as shown in the figure.

Find the
1) resultant electric force on a charge Q, and
2) potential energy of this system.
Figure shows the field lines due to a positive point charge. Give the sign of potential energy difference of a small negative charge between the points Q and P.
1 volt is equivalent to ______.
Consider a uniform electric field in the z-direction. The potential is a constant ______.
- Assertion (A): An electron has a high potential energy when it is at a location associated with a more negative value of potential, and a low potential energy when at a location associated with a more positive potential.
- Reason (R): Electrons move from a region of higher potential to region of lower potential.
Select the most appropriate answer from the options given below:
Calculate potential energy of a point charge – q placed along the axis due to a charge +Q uniformly distributed along a ring of radius R. Sketch P.E. as a function of axial distance z from the centre of the ring. Looking at graph, can you see what would happen if – q is displaced slightly from the centre of the ring (along the axis)?
- Assertion (A): Work done in moving a charge around a closed path, in an electric field is always zero.
- Reason (R): Electrostatic force is a conservative force.
Justify your answers for each case.
State the significance of the negative value of electrostatic potential energy of a system of charges.
Three charges are placed at the corners of an equilateral triangle ABC of side 2.0 m as shown in the figure. Calculate the electric potential energy of the system of three charges.
Charges (+q) and (–q) are placed at points A and B respectively which are a distance 2L apart. C is the midpoint between A and B. What is the work done in moving a charge +Q along the semicircle CRD?